The tricky part is to explain the plateaus in $\sigma_{xy}$ and why they coincide with vanishings of $\sigma_{xx}$.
To make this more evident let us suppose first you had a system without disorder, say you have an interacting Galilean that is fully translationally invariant. Ideally this would be realized e.g. in a Torus. Then you can show, following Kohn's theorem, that the Hall conductivity is always exactly $\sigma_{xy}=\nu e^2/h$, and that $\sigma_{xx}=0$. This is completely independent of the microscopic state of the system, e.g. it woud be true if you had a Laughlin state or a Charge density wave or anything. It follows from the fact that the center of mass of the system as a whole moves as a free charged particle in electric and magnetic fields. Thus there are no plateaus in this translationally invariant limit: the Hall conductivity traces a straight line when you plot it vs $\nu$.
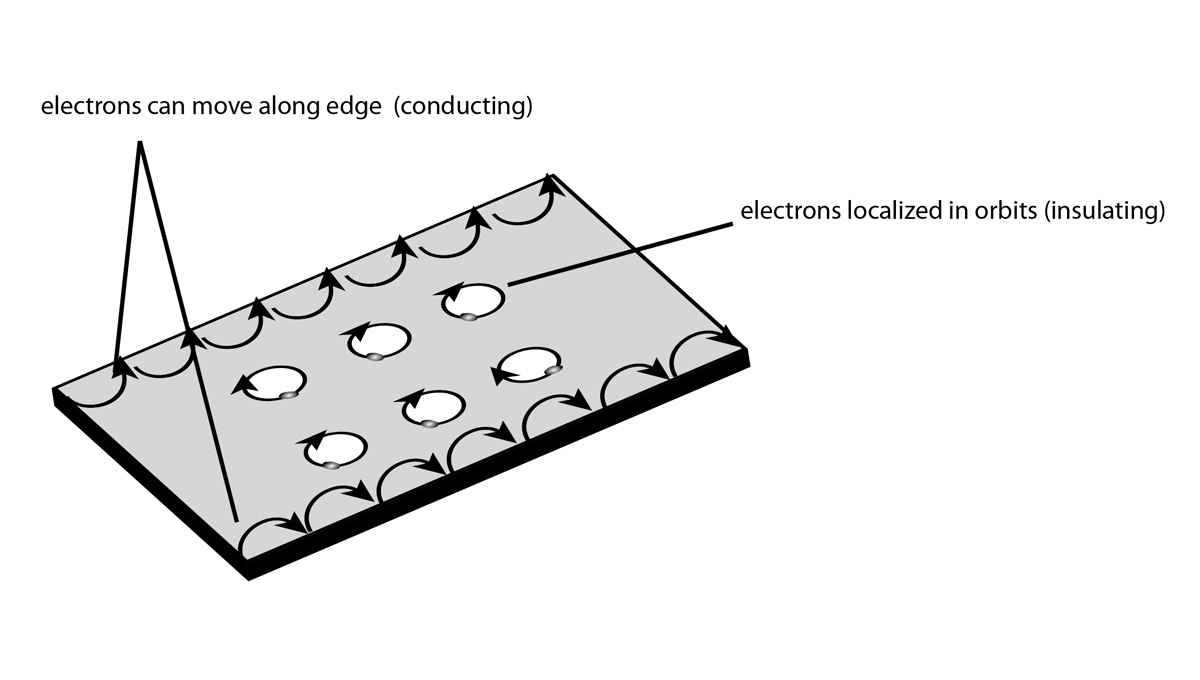
Therefore, what is non-trivial is to explain why the Hall conductivity gets "stuck" at $\sigma_{xy}=\nu_0 e^2/h$ when you change the filling $\nu$ slightly away from a special filling $\nu_0$, namely, the existence of a "plateau" and its coincidence with the vanishing of $\sigma_{xx}$. Essentially, the picture is that as you change the electron density (filling) away from $\nu_0$, they are added into states localized by the disorder and don't contribute to transport (A caveat is that there can be bulk states deep below the chemical potential that are delocalized and can contribute to current flow, see previous reponse).
In the Laughlin argument, which essentially applies only to non-interacting disordered electrons (i.e. only to the integer quantum Hall effect), after threading a flux quantum the Hamiltonian is the same, but the state has changed into an excited state. In this excited state, the occupation numbers of the single particle eigenstates have changed (we are imagining here the exact eigenstates of the single body problem in the presence of disorder). One needs to argue that the change of electron occupations occurs only at the edge and not in the bulk. This is why the argument relies not only on gauge invariance, but also in the existence of a bulk mobility gap. As long as the chemical potential lies in the bulk mobility gap, the electron occupation will only change at the edge after the flux insertion, and due to electron number conservation, this change is an exact integer which determines the Hall conductivity.