1
$\begingroup$

The Klein-Gordon equation explicitly reads

$\left( \frac{\partial ^2}{c^2\partial t^2} - \nabla ^2+\left( \frac{m_0 c}{\hbar}\right)^2\right) \psi =0$

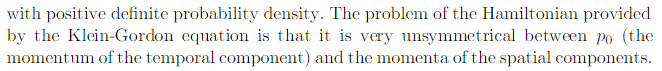
Now I read here on page 8 that: enter image description here

What is meant by this, does he mean the $c^2$? Why is that a problem? I think it's also worded in a weird way, how does a component have a momentum? Isn't it the other way around?

Improve this question
$\endgroup$
3
  • 3
    $\begingroup$ Its more likely that the author was talking about the relative sign of the time and spatial derivatives. Without more context, I can't tell you why he believed that to be a problem though! $\endgroup$
    – Prahar
    Commented Aug 14, 2015 at 0:45
  • $\begingroup$ @PatronBernard I know this question was already answered, but I just noticed that the paper at the link you provided contains no text similar to the one you posted, on pg.8 or elsewhere. Is it still the right link? $\endgroup$
    – udrv
    Commented Aug 15, 2015 at 10:35
  • $\begingroup$ Yeah I can't find it either, perhaps we should delete this question because it offers no real help to anyone else. It's a question about what most likely is a type, so there's no point really. $\endgroup$
    – Jan M.
    Commented Aug 15, 2015 at 11:28

1 Answer 1

Reset to default
3
$\begingroup$

While I can't speak for the author, I find it very likely that this is a typo. The author probably meant the Schrödinger equation, not Klein-Gordon.

Improve this answer
$\endgroup$
1
  • $\begingroup$ Most likely, I don' think it's peer-reviewed. $\endgroup$
    – Jan M.
    Commented Aug 14, 2015 at 12:48

Not the answer you're looking for? Browse other questions tagged or ask your own question.