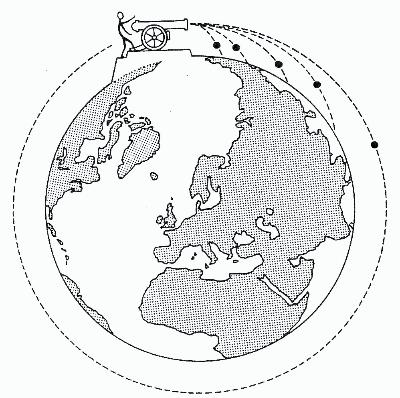
I was searching about 'why do not moon crash to earth due to its gravity?'Then by reading physics stake exchange existing questions I came to know that its the sideways motion of the moon that keeps moon in its orbit.
So my question is:- Moon is in circular or ellipthitical orbit and during its orbit moon is always pulled towards the centre of the earth but moon is changing its direction at every point because it is in circular or ellipthitical orbit, so the direction of force pulling moon towards the centre of the earth will also change at every point on the other hand the sideways motion of moon is always in a particular direction then there must be a point in the moon's orbit when sideways motion and gravity will act in same direction and moon should crash to earth.Then why it does not crash?