In classical electrodynamics, the process of how much light refracts, passing through the glass, and how much light reflects, is determined by the Huygens-Fresnel principle.
This principle, named after Christiaan Huygens and Augustin-Jean Fresnel, is a method of analyzing the wave propagation patterns of light, especially in diffraction and refraction. It states that every unobstructed point on a wave-front emanates secondary spherical waves in all directions. Hence, the net light amplitude at a given point is the vector sum of all wave amplitudes at that point. This principle makes it very useful in visualizing what happens during light diffraction.
Although, as Alex says in his answer, you can use the QFT approach, I would like to provide an alternative answer, using classical, (that is not quantum based) reasoning. It's just easier, for me anyway, to understand :), and, hopefully, to answer.
From Wikipedia: Fresnel Equations
In classical electrodynamics, light is considered as an electromagnetic wave, which is described by Maxwell's equations. Light waves incident on a material induce small oscillations of polarisation in the individual atoms (or oscillation of electrons, in metals), causing each particle to radiate a small secondary wave in all directions, like a dipole antenna. All these waves add up to give specular reflection and refraction, according to the Huygens–Fresnel principle.
In the case of dielectrics such as glass, the electric field of the light acts on the electrons in the material, and the moving electrons generate fields and become new radiators. The refracted light in the glass is the combination of the forward radiation of the electrons and the incident light. The reflected light is the combination of the backward radiation of all of the electrons
When light moves from a medium of a given refractive index n1 into a second medium with refractive index n2, both reflection and refraction of the light may occur. The Fresnel equations describe what fraction of the light is reflected and what fraction is refracted (i.e., transmitted). They also describe the phase shift of the reflected light.
The incident light is polarized with its electric field perpendicular to the plane containing the incident, reflected, and refracted rays. This plane is called the plane of incidence; it is the plane of the diagram below. The light is said to be s-polarized. The incident light is polarized with its electric field parallel to the plane of incidence. Such light is described as p-polarized.
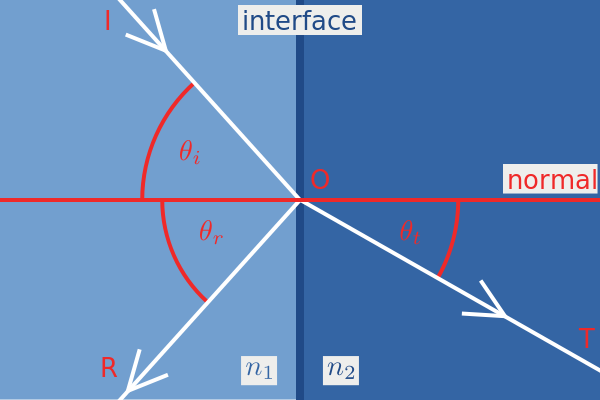
An incident light ray IO strikes the interface between two media of refractive indices n1 and n2 at point O. Part of the ray is reflected as ray OR and part refracted as ray OT. The angles that the incident, reflected and refracted rays make to the normal of the interface are given as θi, θr and θt, respectively.
The relationship between these angles is given by the law of reflection and Snell's law:
The fraction of the incident power that is reflected from the interface is given by the reflectance or reflectivity R and the fraction that is refracted is given by the transmittance or transmissivity T (unrelated to the transmission through a medium).
If you can follow the math, mostly just trigonometry, you can get the proportion of light passing through the glass, and the proportion that reflects, here:
Refraction and Reflection Coefficients
It's not easy , for me at least, to immediately find an answer to your question based on QFT, as most of the QFT explanations seem to deal with mirrors and how they reflect light, rather than explain how some goes through the glass and some reflects, (as in your particular question), but a good explanation, which is basically a copy of Feymann's book, can be found here:
Light Reflection
Just a thought. Is this due to atoms of different substances like water,glass or wood etc curve spacetime differently and thus it influences how photons interact with matter? i.e. some photons reflect and some refract.
I would say no to that reasoning, spacetime is not curved enough in a plate of glass to have a significant effect.

