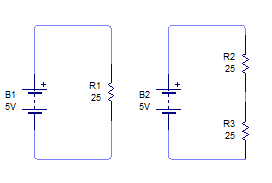
I'm having a hard time understanding the nature of voltage and am hoping you guys can help. The main issue is the concept of the voltage drop.
Take the following circuits :
In regard to the first, we have a 5v supply, which from my understanding means that if you were able to enclose a coulomb of charge eminating from the negative terminal, you'd find that it has 5 joules of energy.
This means that each electron eminating from the negative terminal has :
- 5 / 6.24x10^18e = 0.8x10^18e joules of energy each
(relative to an electron entering the postive terminal)
Now, as the voltage across the battery is 5v and the voltage drop across the resistor is 5v, this means that an electron entering the resistor will have :
0.8x10^18e joules of energy
and one leaving must have 0 energy.
This leads me to a few questions :
1) Why is less energy lost going through R3 than R1? or rather, why does an electron have more energy after passing through R3 than an electron passing through R1
2) If the electron loses all its energy on exiting R1, why does it still end up moving to the positive terminal? If it's still moving to it, doesn't that mean it's got energy?
3) Why doesn't resistance value have an effect on the total energy loss of an electron passing through the circuit?
I understand how this can be explained through ohms law, and I understand that KVL tells us this must be the case, but I want to understand what is physically going on (what the electrons are doing), as opposed to what the equations say.
I'd also appreciate an answer without using an anolgy if possible, as anologies generally always lead me to more questions.