The whole problem is really about knowing what the words mean.
An event is a time and a place together as single object. For instance the event where a light sends its first, last, or only pulse. Or the event where a beam or particle touches something and bounces.
Anything you can describe with a time and place together is an event. And different people that are either using different coordinates or are moving might assign a different collection of four numbers for the $(t,x,y,z)$ describing the time and the spatial coordinates. But it will be the same event. Just lime two people using two spatial coordinates can refer to the same point in space by using a different triple of numbers if they use a different coordinate system.
An observation is when you see things and measure things and then (if necessary) do a computation to find out the numerical values of the event, i.e. find the $(t,x,y,z)$ of the event.
The word simultaneous means two events that happen at the same time, this depends on frame. So it means that in a frame, the observation of the event yields the same $t$ values.
Practice reading that last sentence with all the correct meanings and you are almost there.
A proper length or time is the most extreme version that can be measured in any frame. For a length (proper length) it is the longest length anyone measures, you observe a moving thing to be shorter than the person at rest to it. For a duration (proper time) it is the shortest duration anyone measures, you observe a moving thing to age slower than the person at rest to it.
More mathematically it happens that for two events you can compute $\sqrt{(\Delta x)^2
+(\Delta y)^2
+(\Delta z)^2
-(c\Delta t)^2}$ and you get proper length or you can compute $\frac{1}{c}\sqrt{(c\Delta t)^2
-(\Delta x)^2
-(\Delta y)^2
-(\Delta z)^2} $ and you get proper time whichever one gives a real (not imaginary) result. And everyone agrees in these values so unlike simultaneity, proper time and proper length are things that different frames agree on.
There is something else that different frames agree on. This is that if something (even light) moves at speed $c$ in one (inertial) frame then it moves at speed $c$ in another (inertial) frame.
So that's SR in a nutshell, at least the vocabulary part.
So let's assume that you and the train both move inertially and that you think the train is moving at speed $v$ (and the train thinks you are moving at speed $v$).
Now the person on the ground thinks (observes i.e. computes) the train has length $l<L$ and you want to consider two events that you "observe" to be simultaneous. How can you observe two events to be simultaneous? You'd have to compute when they happen. So what if there was a light source on the train that made a pulse. That would be an event, so in your frame that has a time it happened and a location. Since it is the first event let's label the event with coordinates $(t_1,x_1,0,0)$ then after an amount of time $\Delta t$ that pulse has expanded to a sphere of radius $c\Delta t.$ and when that sphere finally touches the front of the train that is an event. And when the sphere finally touches the back of the train that is an event.
Events are just times and locations, we know where the train is at all times so we know where the front and back of the train is at all times. So if after time $\Delta t$ the sphere finally gets to the front it traveled a distance $c\Delta t$ and hits it at time $t_2=t_1+\Delta t.$ Similarly if after time $\Delta t$ the sphere finally gets to the back it traveled a distance $c\Delta t$ and hits it at time $t_2=t_1+\Delta t.$ And I used the same time $\Delta t$ because I wanted the two events $(t_2,x_2,0,0)$ when-where the light hits the front to be simultaneous to the event $(t_2,x_3,0,0)$ when-where the light hits the back.
Why? Because I just wanted to consider two events both located at the back and front respectively that the person on the ground says happened at the same time. In fact it could be a hypothetical light flash that started in a definite but hypothetical place. Really you can imagine two simultaneous (to the ground person) events and then imagine a hypothetical pulse that would have hit those two locations at that one time.
Because I just wanted to be clear about what two events I was talking about and it can be clearly to reference those two events be referring to a single event. In fact we know the two events, they are $(t_1+\Delta t,x_1+c\Delta t,0,0)$ and $(t_1+\Delta t,x_1-c\Delta t,0,0)$ so really we just picked any two events that were located at the ends of the train and were simultaneous to the person at the ground and then we can imagine a hypothetical light blast that would have hit those two locations at the two times. So really we have one event (the light going off) that is clearly linked to the two events (the light hitting the front and back simultaneous to the person in the ground).
And since events are really just the time-place when-where it happened. These events (the when-where) exist even if there was no light, even if the light was hypothetical.
OK so we know how to relate the events that are simultaneous to the person on the ground to a (potentially hypothetical) event where light flashes.
But where does it happen? Let's say the train has meter and cm and mm marks painted on the floor. The person on the ground doesn't agree that they are calibrated correctly. But they do agree that they are equally spaced and hence they can be used to mark out what percentage of the way across you are.
So what if the light were placed in the center, would that work? By the time the light gets to where the front started the front has since moved on. But by that time the light has also gotten to where the back used to be which has already moved on too, but that means if we placed it there in the center the light hit the back before it hit the front, so that wasn't the place to put it so that it hits the two ends at the same time.
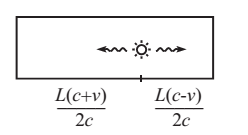
However that was the amount of time $\Delta t$ to wait because that's when that expanding sphere was long enough to reach from one end of where the train used to be to the other end of where the train used to be. So it has a diameter that is the size of the train, it was just placed in the wrong spot. If you move the location of the light over by $v\Delta t$ towards the front of the train then we can ask where it ended up after time $\Delta t$ it is a sphere of the right length whose front end is $v\Delta t$ in front of where the front of the train was originally. So that edge is right where the train's front is right then. That's the perfect spot to be touching both ends.
So if the train has length $l<L$ then $c\Delta t =l/2$ because that is how long it takes for the expanding sphere to get long enough. So the location to start at isn't the center it is $v[\Delta t]$=$v[l/(2c)]$ towards the front compared to the middle.
So it is $(l/2)+(vl/(2c))$ away from the back. So it is $l(c/2c+(v/2c))$ from the back. Which is a fraction $(c+v)/(2c)$ of the way from the back towards the front.
And the two observers disagree about how far from the back this magic place is. But they do agree about what percentage of the way it is.
So now we are done with the observer on the ground. From here on out we are going to work with the train observer. Let's say you asked the guy on the ground where he wants to place a light and they say to place it the fraction $(c+v)/(2c)$ of the way between the front and the back (and they tell you which end is which since you don't feel the train moving) with it closer to the front. Then you see that magic location as $L(c+v)/(2c)$ from the back, hence $L-L(c+v)/(2c)$=$L(c-v)/(2c)$ from the front. So that's where that came from in your text.
All that work was just to make it super crystal clear that there is one event (the light flashing) that clearly identifies two other events (the event of the light hitting the front and the event of the light hitting the back) and we've been asked what the time readings on those clocks at those events are.
Now on the train you placed two clocks, one of the front and one at the back. You think the train is at rest and you think your clocks read the accurate time when it is the same time. So to find out the reading of the clock when the light strike them. We just need to know the time when the light strikes them.
So if the first event (light going off) happens at $(t_3,L(c+v)/(2c),0,0)$ (I had to use a different time because it is a different frame, same event but different frame just like the same vector has different components in different coordinate systems) then the event of the light hitting the back happens $\frac{L(c+v)/(2c)}{c}$ seconds later at $t=t_3+\frac{L(c+v)/(2c)}{c}.$ Whereas the event of the light hitting the front happens $\frac{L(c-v)/(2c)}{c}$ seconds later at $t=t_3+\frac{L(c-v)/(2c)}{c}.$
So the front hit first (it was closer to the front) and so the clock in the front reads $t_3+\frac{L(c-v)/(2c)}{c}$ and the clock at the back reads $ t_3+\frac{L(c+v)/(2c)}{c}$ which is ahead of the one in the front by $\left(t_3+\frac{L(c+v)/(2c)}{c}\right)$-$\left(t_3+\frac{L(c-v)/(2c)}{c}\right)$=$Lv/c.$
Time to get to your questions.
Why is it legitimate to use light source to represent you seeing the two clocks?
We used the one event of the light flash to clearly indicate which two events (lights hitting the ends) we were trying to talk about. Later you can use coordinates and Lorentz transformations to compute the same things with much less effort.
Everyone would agree that there is a time and place a light goes off. And everyone agrees there is a time and a place when-where that light reaches the front. And everyone agrees there is a time and a place when-where that light reaches the back. They just might. It agree on those times. Or places or even about whether the times are the same. But everyone clearly agrees on the events. In a sense the events are labels for a region of spacetime (a 4d collection like space is a 3d collection) and people will disagree about what numbers to assign to them, but they will agree that this is the event where that did or could have happened and so forth.
How is it justified that the time difference between light hitting the ends in train's frame is indeed the difference you see in your frame?
That's why I had to write the whole answer. We literally computed what the clocks read at those two events. If the train had glass walls and traveled through a dark universe and that light went off then those two clocks readings would literally be what your eyeballs see from he clocks since the only time you see the clock us when he pulse of light hits it. You can't see in the dark so those two events would be the only times you saw the clocks.
Now if by see in your frame, don't think you see those images at the same time. You don't see those clocks until the light gets from them to you, but I set it up so after you collect the data you will compute that you saw the clock readings from the same time.
We worked hard to place the light at exactly the right spot so the pulse from the light would hit the clocks on the train at a time that you (on the ground) compute to be simultaneous.
So the answer here is that all the effort I went to to find that magic spot, the spot $v\Delta t$ from the center of where the train was originally. That effort was so that those two events are things you think happened at the same time, you think both events happened $\Delta t$ after the one event of the light going off.