It's a complicated subject - but very well studied in the context of eddy current brakes, where the retarding force is used to create a braking force without mechanical friction / wear. For me, the starting point for finding out more was this post - in particular the posting by Jim Hardy contained lots of good links.
It seems that some of the most significant analyses were done by Smythe (1942), Schiber (1974) and Wouterse (1992) - see this thesis chapter for lots of detail.
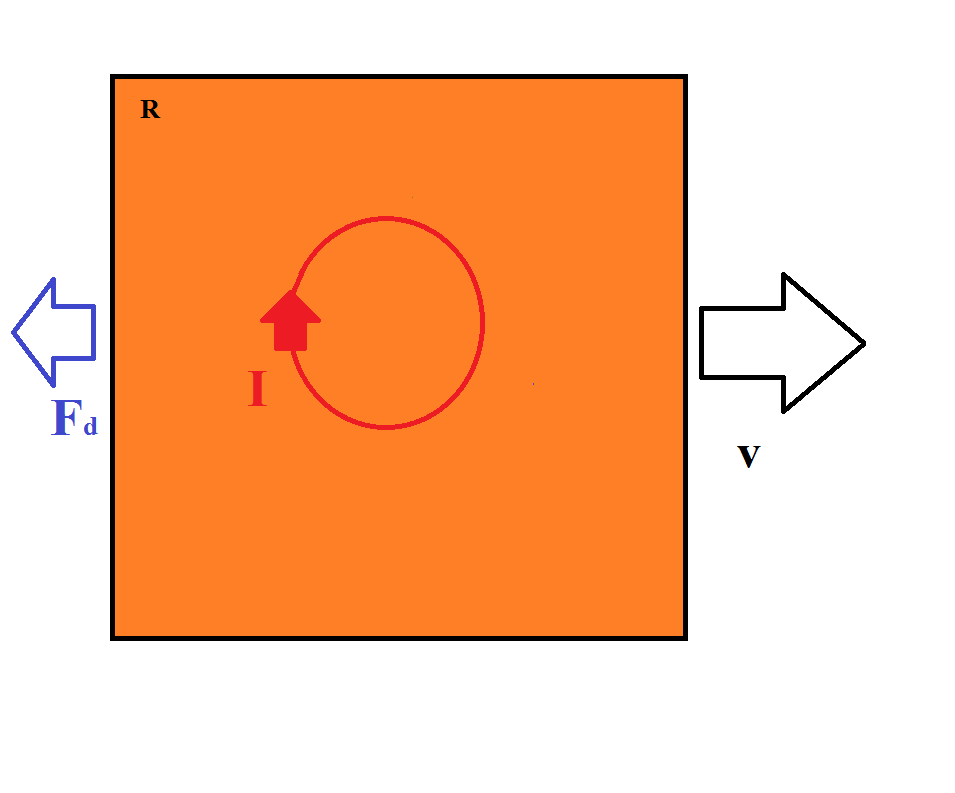
Just summarizing, it seems there are four significant factors (surprise). These are
- The magnetic field itself: you expect the force to scale with the square of the field since the current induced scales with the field, and the force scales with the product of field and current.
- The velocity of the motion: the faster you move, the greater the change in flux and thus the greater the force. To first order you expect a linear relationship, although there is an indirect effect on resistance:
- The effective resistance of the plate (resistance per unit area). For non-ferromagnetic materials, the skin effect is not very large and current will flow through the body of the plate: but with ferromagnetic materials and high velocities, the current will only occur in the surface. This increases the resistance, and lowers the current and therefore the force
- The size of the magnetic patch: the larger the patch, the greater the line segment of current on which the magnetic force can act.
This means that for low speed, the force is expressed as
$$F = \frac{v\cdot B^2\cdot A}{\rho/t}$$
where $v$ is the velocity, $B$ the magnetic field, $A$ the area of the magnetic patch, $\rho$ the volume resistivity and $t$ the thickness of the plate (area resistivity $\sigma = \rho / t$). Note that I modified the equation given in the reference slightly - it was showing the torque for a rotating disk, from which I deduced the force ($F = \frac{\Gamma}{R}$)
Now when the speed becomes higher, the induced currents can generate a field that is a significant fraction of the applied field; and as I mentioned, the skin effect can start to play. Both of these will generate additional term in the relationship between force and velocity, but at this point the calculations become tricky to do analytically and are typically done by fitting experimental data.
But the above should give us a start. It tells us that thicker conductors experience greater force, and that as things move faster, the force will increase. There is a lovely experiment demonstrating eddy currents in which a strong magnet is dropped down a thick copper tube, and appears almost to float: this observation is entirely consistent with the above equation (you need thick copper to get sufficient induction; and since the force increases with velocity there will be a velocity at which the retarding force cancels gravity).
A video showing this phenomenon is shown here - incidentally it shows that the effect is stronger for the copper tube than for the aluminum tube, as is consistent with the fact that the volume conductivity of copper is greater than that of aluminum (by about 1.5x).