Free fall into circular motion

Question

If I'm on a roller coaster free falling from height $h$ and then suddenly start going into horizontal motion with a radius $r$ of turn what is the $g$-force I experience?

I worked out the equation like this but am not sure if it is correct:

• (1) instant velocity of free-fall $v=\sqrt{2 g h}$
• (2) uniform circular motion acceleration $a = \frac{v^2}{r}$
• (3) $g$-force $ gf = \frac{a}{g} = \frac{v^2}{g r}$

My doubts are:

• I don't know if I can use uniform circular motion equation since $v$ is not constant
• Where is the g-force directed towards? The center of the turn?

Answer 1

There are two accelerations involved: The gravitational acceleration $g$ that points down, and the centripetal acceleration $a_r = \frac{v^2}{r}$ that points along the radius vector of the curve. The component of the gravitational acceleration that is tangential to the curve does not contribute to the g-force as it accelerates the cart and us in this direction. We do feel the component of the gravitational acceleration that points along the radius vector given by $a_g = g \text{sin}\phi$ where $\phi \in [0,\frac{\pi}{2}]$ is the angle that starts with zero when you enter the curve and ends with $\frac{\pi}{2}$ when you enter the horizontal part. We can add the two accelerations as they are parallel:

$$a_{tot} = a_g +a_r$$

We now observe: $ v = \sqrt{2g(h+r\text{sin}\phi)}$ and so

$$a_r = \frac{v^2}{r} = \frac{2g(h+r\text{sin}\phi)}{r}$$

thus:

$$a_{tot} = g \text{sin}\phi + \frac{2g(h+r\text{sin}\phi)}{r} = 3g \text{sin}\phi + \frac{2gh}{r}$$

The g-force is $ a = \frac{a_{tot}}{g}$ thus

$$a = 3 \text{sin}\phi + \frac{2h}{r}$$

So the g-force is no constant but depends on where you are in the curve (on $\phi$). And as sin is monoton increasing over $[0,\frac{\pi}{2}]$ so is the g-force. it reaches it's maximum as it enters the horizontal part.

What you might also wanto observe is that if you build a rollercoaster like this people would run screaming, as the g-force is not continues. There is a jump as you enter the curve and a big jump as you go onto the horizontal. And basically at these discontinuities the g-force is infinite :)

Answer 2

The roller coaster falls a height $h$ before entering the loop (the path of the RC will look like a J. The straight bit of the J is of length $h$), and I assume it started with zero velocity. Within the loop it's total kinetic energy will equal the total potential energy lost, which is $$ E_\text{kin}=mg(h+r\sin\theta). $$ Here $\theta$ is defined such that it is zero at the end of the straight bit of the J, i.e., it is defined wrt to a horizontal axis. Also note that $h+r\sin\theta$ is just the total height the RC has lost. Therefore, at any point in the circle, the speed of the RC is $$ v=\sqrt{2g(h+r\cos\theta)}. $$ The total force on the RC has two components. One is directed straight to the centre of the turn, as you said, and the other one is gravity. In the absence of the latter, the centripetal acceleration (your second formula) $$ a=\frac{2g(h+r\sin\theta)}{r} $$ would be sufficient to force the RC on a circular path. However, gravity also tries to push the RC away from the circle, adding a contribution $$ a_\bot=g\sin\theta. $$ (This is simply the component of $g$ that points radially outward.) Therefore the total $g$-force (=total acceleration divided by $g$) is $$ \frac{2(h+r\sin\theta)}{r}+\sin\theta = \frac{2h}{r}+3\sin\theta. $$ The force does indeed point radially inwards (remember that you can't "feel" the pull of gravity -- the only force is the one from the tracks which can only push "up" and neither forwards nor backwards (neglecting friction of course)).

Answer 3

SECTION A : Free fall of roller coaster into circular motion (kinetics)

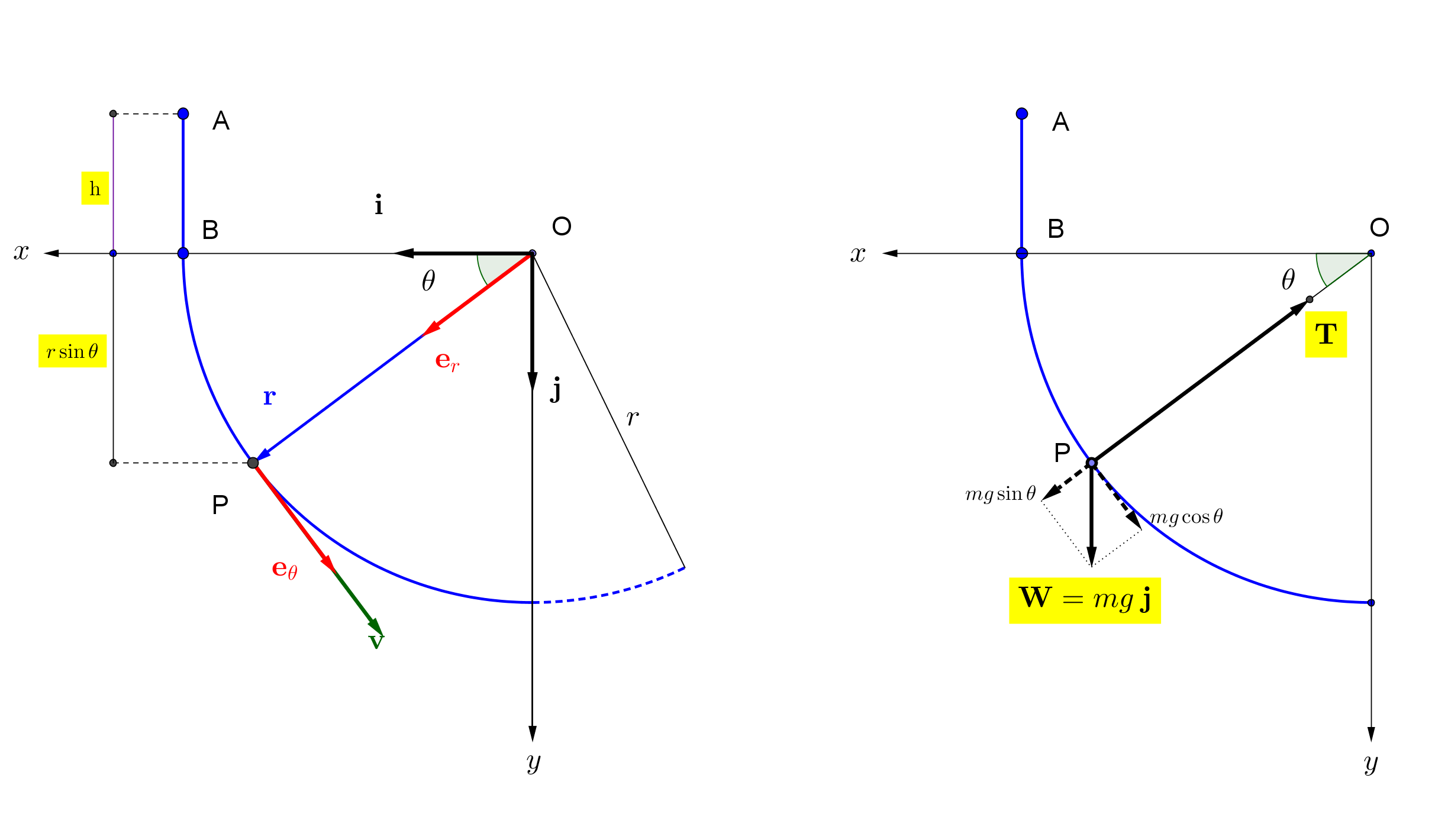
Suppose that the roller coaster, called from now on "particle", is at rest at point A ($\:\upsilon_{A}=0\:$) and starts free falling till point B where it starts its circular motion. Well-known is that at B the speed is $\:\upsilon_{B}=\sqrt{2gh}\:$ under the assumption of no energy loss (zero air resistance etc).

Now, if the meaning of $\:g$-force in present case is the acceleration caused by the "pushing up" force $\:\mathbf{T}\:$ in $\:g \:$ units, then we must determine the magnitude $\:T=\Vert\mathbf{T}\Vert \:$ and by this : $\:g$-force =$\: T/mg\:$.

As shown in above Figure, the force $\:\mathbf{T}\:$ is normal to the circular orbit with the assumption of no friction. If $\:\mathbf{W}\:$ is the weight of the particle and $\:\mathbf{a}\:$ its acceleration then : \begin{equation} \mathbf{T} + \mathbf{W}= m\mathbf{a} \tag{A-01} \end{equation} It's convenient to use the $\:\left(r,\theta \right)\:$ coordinates. The expression of $\:\mathbf{a}\:$ as proved in SECTION B, equation (B-13), is

\begin{equation} \mathbf{a}=\underbrace{\left(-r\dot{\theta}^2\right)\mathbf{e}_{r}}_{centripetal}+\underbrace{\left(r\ddot{\theta}\right)\mathbf{e}_{\theta}}_{orbital} =\mathbf{a}_{r}+\mathbf{a}_{\theta} \tag{A-02} \end{equation} So the analysis of (A-01) in $\:\mathbf{e}_{r}\:$ and $\:\mathbf{e}_{\theta}\:$ components is respectively \begin{align} \left( \mathbf{T} + \mathbf{W}\right)\circ\mathbf{e}_{r} &= m\mathbf{a}_{r}\: \Longrightarrow \: -T + mg\sin\theta =- mr\dot{\theta}^2 \tag{A-03a}\\ \left( \mathbf{T} + \mathbf{W}\right)\circ\mathbf{e}_{\theta} &= m\mathbf{a}_{\theta}\: \Longrightarrow \: mg\cos\theta =mr\ddot{\theta} \tag{A-03b}\\ \end{align} Only equation (A-03a) is important here since \begin{equation} T= m\;r\dot{\theta}^2 + m\;g\sin\theta \tag{A-04} \end{equation} To find an expression for $\:\dot{\theta}\:$ we use equation (B-09) \begin{equation} \dot{\theta}=\dfrac{\upsilon}{r} \tag{A-05} \end{equation} since in this case and at any instant $\:t\:$ the motion is counter-clockwise ($\:\dot{\theta}> 0\:$).

The magnitude $\:\upsilon \:$ at any point P on the circular orbit is determined from the energy conservation under the assumption of no energy loss (zero air resistance, zero friction on the track etc): \begin{equation} \dfrac{1}{2}m \Delta \upsilon^{2} + mg\Delta y = 0\:\Longrightarrow \: \dfrac{1}{2}m \left( \upsilon^{2}-\upsilon_{A}^{2}\right)=mg\left(h+r\sin\theta\right) \tag{A-06} \end{equation} so (since $\:\upsilon_{A}=0\:$) \begin{equation} \upsilon=\sqrt{2g\left(h+r\sin\theta\right)} \tag{A-07} \end{equation} and \begin{equation} r\dot{\theta}^2=\dfrac{2g\left(h+r\sin\theta\right)}{r} \tag{A-08} \end{equation} Inserting above expression in (A-04) yields \begin{equation} T= mg\left( \dfrac{2h}{r}+3\sin\theta\right) \tag{A-09} \end{equation} Finally \begin{equation} \text{$g$-force}=\dfrac{T}{mg} = \dfrac{2h}{r}+3\sin\theta \tag{A-10} \end{equation}

Note : In above Figure under scale the ratio $\:\dfrac{h}{r}\:$, the position P (that is $\:\sin\theta\:$) and the resulting $g$-force are as follow \begin{equation} \dfrac{h}{r}=0.40 \:,\quad \sin\theta =0.60 \:\Longrightarrow \: \text{$g$-force}=2.60 \tag{A-11} \end{equation}

---

SECTION B : Kinematics of a Particle in Circular Motion

The following analysis concerns the kinematics of a particle in plane circular motion exclusively. It's a special case of a plane curvilinear motion which in its turn is special case between curvilinear motions in space.
The motion of a particle is given by the vector function $\mathbf{r}\left(t\right)$, that is by its position in time $\:t \:$. The velocity vector $\mathbf{v}\left(t\right)$ is the rate of change in time of this position vector

\begin{equation} \mathbf{v}\left(t\right)\equiv \dfrac{d\mathbf{r}}{dt}= \dot{\mathbf{r}} \tag{B-01} \end{equation}

We'll use one upper dot or two upper dots for the 1st or 2nd derivative with respect to $\:t\:$, for example

\begin{equation} \dot{\mathbf{r}}\equiv \dfrac{d\mathbf{r}}{dt}\;, \quad \dot{\theta}\equiv \dfrac{d\theta}{dt}\;, \quad \ddot{\theta}\equiv \dfrac{d^{2}\theta}{dt^{2}} \tag{B-02} \end{equation}

Now, let a system of coordinates $\:\left(x,y\right)\:$ in the plane as in above Figure and $\:\mathbf{i},\mathbf{j}\:$ the unit basic vectors along axis $\:Ox,Oy\:$ respectively. For plane circular motion the position vector $\mathbf{r}\left(t\right)$ of the particle may be expressed as follows : \begin{equation} \mathbf{r}\left(t\right)= \left[r \cos \theta \left(t\right)\right]\mathbf{i}+\left[r \sin \theta \left(t\right)\right]\mathbf{j} \tag{B-03} \end{equation}

Note that all quantities as position vector $\:\mathbf{r}\:$, velocity vector $\:\mathbf{v}\:$, acceleration vector $\:\mathbf{a}\:$, angle $\:\theta\:$ and as we see bellow the unit vectors $\:\mathbf{e}_{r},\mathbf{e}_{\theta}\:$ are functions of time and so it's convenient to omit $\:t\:$. The magnitude $\:r=\Vert\mathbf{r}\Vert\:$ of the position vector is of course constant in time.

So (B-03) yields \begin{equation} \mathbf{r}= r\left[\left(\cos\theta\right)\mathbf{i}+ \left(\sin\theta\right)\mathbf{j}\right]= r\mathbf{e}_{r} \tag{B-04} \end{equation} where by definition \begin{equation} \mathbf{e}_{r} \equiv \left(\cos\theta\right)\mathbf{i}+ \left(\sin\theta\right)\mathbf{j} \tag{B-05} \end{equation} is a unit vector along $\:\mathbf{r} \:$, as in Figure. The velocity vector is \begin{equation} \mathbf{v}=\dfrac{d\mathbf{r}}{dt}= \dot{\mathbf{r}}=r\dot{\theta}\left[\left(-\sin\theta\right)\mathbf{i}+ \left(\cos\theta\right)\mathbf{j}\right]= r\dot{\theta}\mathbf{e}_{\theta} \tag{B-06} \end{equation} where by definition \begin{equation} \mathbf{e}_{\theta} \equiv \left(-\sin\theta\right)\mathbf{i}+ \left(\cos\theta\right)\mathbf{j} \tag{B-07} \end{equation} is a unit vector tangent to the circle and normal to $\:\mathbf{r} \:$, as in Figure. Note that :

(a) The quantity $\:\dot{\theta}\:$ is essentially the instantaneous angular velocity \begin{equation} \dot{\theta}\equiv \dfrac{d\theta}{dt}=\omega\left(t\right) \tag{B-08} \end{equation} If $\:\dot{\theta}=\omega_{o}=\text{constant}$, then we have uniform circular motion.

(b) from (B-06) for the magnitude $\:\upsilon\:$ of the velocity $\:\mathbf{v} \:$ \begin{equation} \upsilon =r\vert\dot{\theta}\vert=r\vert\omega\vert \tag{B-09} \end{equation} where $\:\omega=\dot{\theta}> 0\:$ ($\:<0\:$) if the particle moves instantly counter-clockwise (clockwise).

(c) as a general rule, if a variable vector $\:\mathbf{w}\left(\lambda\right)\:$, where $\:\lambda \:$ a real parameter, has constant norm, then its 1st derivative is always normal to it \begin{equation} \Vert\mathbf{w}\Vert ^{2}=\text{const}\rightarrow \mathbf{w}\circ \mathbf{w}=\text{const}\rightarrow \dfrac{d\left( \mathbf{w}\circ \mathbf{w}\right)}{d\lambda}=0\rightarrow \left( \mathbf{w}\circ \dfrac{d\mathbf{w}}{d\lambda}\right)=0 \tag{B-10} \end{equation} That's why $\:\dot{\mathbf{e}}_{r}=\dot{\theta}\mathbf{e}_{\theta}\:$, that is $\:\mathbf{e}_{\theta}\:$, is always normal to $\:\mathbf{e}_{r}\:$.

For the acceleration $\:\mathbf{a}\:$ we have from (B-06) \begin{equation} \mathbf{a}=\dot{\mathbf{v}}=\dfrac{d\mathbf{v}}{dt}=\dfrac{d\left(r\dot{\theta}\mathbf{e}_{\theta}\right)}{dt}=\left(-r\dot{\theta}^2\right)\mathbf{e}_{r}+\left(r\ddot{\theta}\right)\mathbf{e}_{\theta} \tag{B-11} \end{equation} since from (B-07) \begin{equation} \dot{\mathbf{e}}_{\theta}=\;-\;\dot{\theta}\mathbf{e}_{r} \tag{B-12} \end{equation} Equation (B-11) is written as \begin{equation} \mathbf{a}=\underbrace{\left(-r\dot{\theta}^2\right)\mathbf{e}_{r}}_{centripetal}+\underbrace{\left(r\ddot{\theta}\right)\mathbf{e}_{\theta}}_{orbital} =\mathbf{a}_{r}+\mathbf{a}_{\theta} \tag{B-13} \end{equation}

Acceleration vector $\:\mathbf{a}\:$ is analysed in two normal components

(1) The so called centripetal acceleration \begin{equation} \mathbf{a}_{r} \equiv \left(-r\dot{\theta}^2\right)\mathbf{e}_{r} \tag{B-14} \end{equation} which is pointing always inwards to the center and is "trying" to change only the direction of the velocity vector $\:\mathbf{v}\:$, and

(2) The orbital (tangent) acceleration \begin{equation} \mathbf{a}_{\theta} \equiv \left(r\ddot{\theta}\right)\mathbf{e}_{\theta} \tag{B-14} \end{equation} which is always tangent to the circle and is "trying" to change only the magnitude of the velocity vector $\:\mathbf{v}\:$. Note that the acceleration vector $\:\mathbf{a}\:$ doesn't point to the center in general.

Answer 4

You're good.

Yeah, you can pretty much assume that it's a constant velocity, as long as $h \gg r.$ As I recall, the expressions involved are extremely simple as long as you don't try to figure out exactly what's happening in time: actually solving the Euler-Lagrange equations gives you some sort of $\int d\theta / \sqrt{a + b \sin\theta} = t$ equation for $\theta(t)$, or something awful like that.

So, let's have some coordinates and geometry: You start out at $[x, y] = [0, r + h]$, then at $[x, y] = [0, r]$ you enter a circle centered at $[r, r]$: and your progress along that circle I'll denote with $\theta$ as $\vec r = [x, y] = [r, r] - r\cdot[\cos\theta, \sin\theta]$. You then emerge after $\theta = \pi/2$ at position $[r, 0]$, moving forward. We'll express time derivatives as dots, and I'll define $\omega = \dot\theta$.

Your net acceleration during this arc is $\vec a(\theta) = - g\cdot[0, 1] + c(\theta)\cdot [\cos\theta, \sin\theta]$ for some $c(\theta)$, since that's the direction the constraint force pushes. However we know that this must also have a very special shape: $$\ddot{\vec r} = \frac{d}{dt} \left(r~\omega~[\sin\theta, -\cos\theta]\right)= r~ \dot\omega~ [\sin\theta, -\cos\theta] + r~ \omega^2~ [\cos\theta, \sin\theta].$$The $x$-component gives the simpler version of this constraint, $c(\theta) = r~ \dot\omega~ \tan\theta + r~ \omega^2.$

Since $\dot{\vec r} = \vec v = r~\omega~[\sin\theta, \cos\theta]$, we can quickly state that from energy conservation$$v^2 = r^2\omega^2 = 2g(h + r\sin\theta),$$as the constraint force does no work. With a time-derivative this also gives$$r^2~2~\omega ~\dot \omega = 2 ~g ~r~\omega~ \cos\theta $$so we have $r \omega^2 = 2g(\sin\theta + h/r)$ and $r~\dot\omega=g\cos\theta,$ so if I've done all of the mathematics right,$$c(\theta) = g~\left(3~\sin\theta + 2~\frac hr\right).$$In the limit $h\gg r$ you get an approximately-constant $c(\theta) = 2 h g / r$, and so your g-force on the turn is maximized at $\theta\approx0$ where it is $a \approx \sqrt{g^2 + c^2} \approx c.$

This solution corresponds exactly to $v^2 / r$ with $v = \sqrt{2 g h}$, as you're inclined to do.

If you don't have $h \gg r$, then you'll have to instead either space-average the force (as I said, time-averaging is probably a nightmare) or maximize the force with$$\frac d{d\theta} \big\lVert[c(\theta)~\cos(\theta),~~c(\theta)~\sin(\theta) - g]\big\rVert = 0.$$In general you will find that your acceleration points "almost" at the center of rotation but a little bit lower than it, because the gravitational force is also in there.

Answer 5

I don't know if I can use uniform circular motion equation since v is not constant

The equation for centripetal force is independent of whether the motion is uniformly circular or not.

However, irrespective of the radius of the track, the velocity at that point, and the weight of the roller-coaster, or whether the equation for centripetal force is still valid, etc; since it becomes horizontal at that particular point, any force that must cause your 'g-force' must be acting in the horizontal direction. As no such force acts in the horizontal, the g-force on you as soon as you go horizontal is zero. As simple as that.

Answer 6

You asked two simple questions - I will give two simple answers.

I don't know if I can use uniform circular motion equation since v is not constant

At the very instant that the curve starts, the velocity is given by $\sqrt{2gh}$ - and for that first instance it is constant. So yes, you can use uniform circular motion

Where is the g-force directed towards? The center of the turn?

It depends on how you define "G force". Usually, it is the "non gravitational acceleration experienced". If that is so, then it is pointing at the center of the circle at the moment you start moving around the circle.

If you accept that a person experiences "1 g" when standing still, then the g force due to gravity will depend on the angle of the rail - it will increase with the $\sin$ of the angle of the radial vector, and will cause the force to point slightly above the center of the circle.

Of course real rollercoaster tracks describe a spline - that is, the rate of change of curvature is continuous. Otherwise the sudden change in g force would be most unpleasant.