8
$\begingroup$

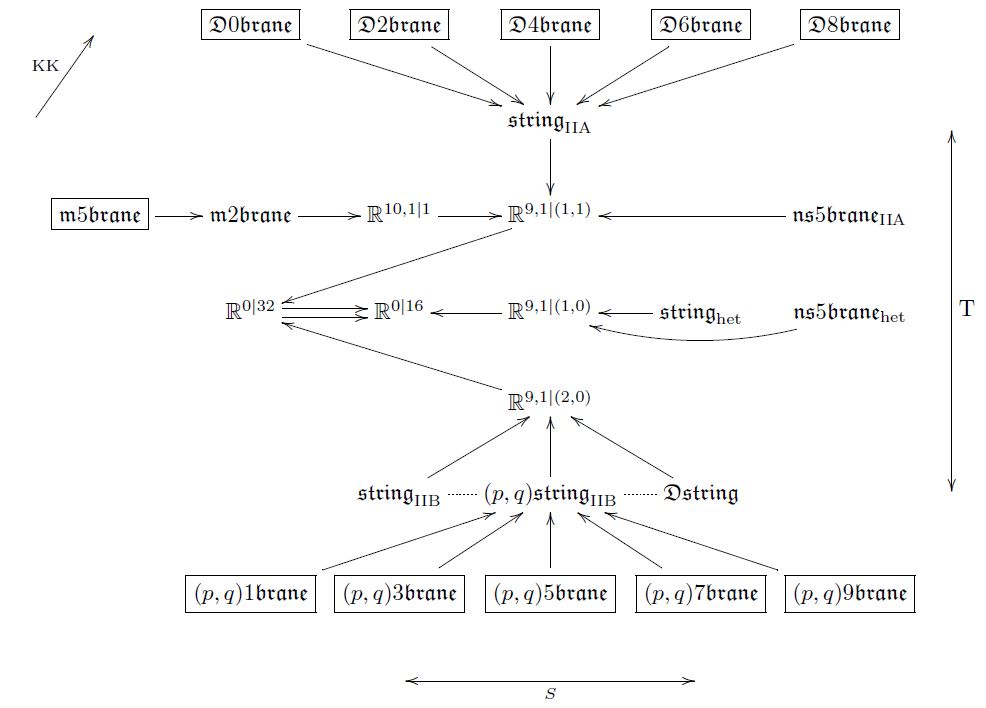
Why does the heterotic string (or heterotic supergravity) have no brane solutions?

According to David Tong's notes:

the heterotic string doesn’t have (finite energy) D-branes. This is due to an inconsistency in any attempt to reflect left-moving modes into right- moving modes.

I know that the heterotic string does not have the same structure in the left- moving and right- moving sectors (whether one uses the Green Schwarz or the RNS formulation). But why does that imply no branes?

BPS-brane solutions are obtained by starting from the supersymmetry transformations of the fields and solving them in a zero-fermion background. This leads to stable solutions if they exist. Why would such an approach not work with the heterotic supergravity action?

EDIT: It seems that there is a simple qualitative explanation (source). Specifically, a heterotic string must necessarily be closed, and unlike other string theories which have closed strings and open strings (which end on D-branes) there are no heterotic open strings of type $E_8 \times E_8$ (cf. this paper, which I haven't yet read) and so no D-branes.

Improve this question
$\endgroup$
1
  • 4
    $\begingroup$ Actually, there are branes in heterotic string theory, but they just don't behave as boundary conditions for fundamental strings. See here for an answer. $\endgroup$
    – Abhimanyu Pallavi Sudhir
    Commented Jun 30, 2015 at 4:24

1 Answer 1

Reset to default
8
$\begingroup$

The systematic way to deduce the brane content of string/M-theory is to classify the WZW-terms ($\kappa$-symmetry terms) for the would-be Green-Schwarz action functionals for the possible super $p$-branes (the "brane scan" or "brane bouquet").

Doing this for the would-be D-branes for the heterotic string, one proves that the required non-trivial cocycles don't exist.

This is shown in

C. Chrysso‌malakos, José de Azcárraga, J. M. Izquierdo and C. Pérez Bueno, "The geometry of branes and extended superspaces", Nuclear Physics B Volume 567, Issues 1–2, 14 February 2000, Pages 293–330 (arXiv:hep-th/9904137)

see footnote 14 there, a side remark in the corresponding computation which finds the D-branes in type IIA this way.

The analogous computation classifying the type IIB branes is in section 2 of

Makoto Sakaguchi, "IIB-Branes and New Spacetime Superalgebras", JHEP 0004 (2000) 019 (arXiv:hep-th/9909143).

One may organize these computations in a classifying "bouquet" of super Lie $n$-algebra extensions, which shows the brane spectrum in string/M-theory systematically from super Lie $n$-algebra cohomology:

Here each item denotes a super Lie $n$-algebra and each edge denotes a Lie $n$-algebra extension classified by the relevant super Lie $n$-algebra cocycle needed for the Green-Schwarz sigma-model. Moreover, for every edge the brane species that it points to may end on the brane species that it starts at.

The un-boxed items are those branes appearing already in the "old brane scan", while the boxed items are those branes appearing only as one generalized from super Lie algebras to super Lie $n$-algebras. Since there are no relevant extensions over the super Lie 2-algebra coresponding to the heterotic string, there are no branes that it may end on, in contrast to the type II strings.

For more see

Domenico Fiorenza, Hisham Sati, Urs Schreiber, "Super Lie $n$-algebra extensions, higher WZW models and super $p$-branes with tensor multiplet fields", International Journal of Geometric Methods in Modern Physics, Volume 12, Issue 02 (2015) 1550018 (arXiv:1308.5264)

Improve this answer
$\endgroup$
11
  • 2
    $\begingroup$ The reason why branes on which other branes may end have Green-Schwarz cocycles not on the spacetime super Lie algebra, but on the super Lie n-algebra extension that are classified by the GS-cocycles for the branes that end on them is explained in remark 3.3 of arxiv.org/abs/1308.5264 . Physically it has to do with the fact that these branes have gauge fields on their worldvolume: this means that if we still model them as sigma-models, then their target space needs to be a twisted product of ordinary spacetime with the classifying stack for the corresponding gauge field. See page 25. $\endgroup$
    – Urs Schreiber
    Commented Aug 26, 2016 at 12:29
  • 1
    $\begingroup$ The degree n is as follows: the WZW cocycle for a p-brane has degree p+2. The extension that this classifies is a Lie p+1-algebra (in Stasheff's sense, not in Filippov's). If the original cocycle is already on a Lie p1+1-algebra- then the extended Lie p+1-algebra corresponds to a brane with a p1-form gauge field on its worldvolume. $\endgroup$
    – Urs Schreiber
    Commented Aug 26, 2016 at 12:40
  • 1
    $\begingroup$ Regarding the question concerning M-theory on a (Horava-Witten-type) boundary: $\endgroup$
    – Urs Schreiber
    Commented Aug 26, 2016 at 12:47
  • 1
    $\begingroup$ So, your paper (arXiv:1308.5264v3) would be a starting point to learn about the WZW cocycle in the first place, am I right? $\endgroup$
    – leastaction
    Commented Aug 26, 2016 at 12:53
  • 1
    $\begingroup$ Regarding the Lie 3-algebra on which the M5-brane is defined: Yes, but in the BGL model (not in the ABJM model, though) it is Filippov n-Lie algebras which appear ncatlab.org/nlab/show/n-Lie+algebra whereas here it is Stasheff Lie n-algebras (L-infinity algebra) which appear ncatlab.org/nlab/show/L-infinity-algebra, the "supergravity Lie 3-algebra" ncatlab.org/nlab/show/supergravity%20Lie%203-algebra , first mentioned on p. 54 of arxiv.org/abs/0801.3480 . $\endgroup$
    – Urs Schreiber
    Commented Aug 26, 2016 at 12:58

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.