Exercise about equilibrium and virtual work [closed]

Question

Two railway carriages of mass $M_1=2m$ and $M_2=m$ are on a 45° inclined plane. A spring, parallel to the plane, keeps both carriages in balance against the gravitational accceleration. The stretching of the spring is based on a linear power law. A coupling keeps a constant distance l between those carraiges. The carriages can be seen as mass points at the center of gravity.

a) How many degrees of freedom does this system have?

b) Determine with the principle of virtual work the equilibrium positions of the carriages.

c)At $t=t_0$ the coupling breaks. How does the equilibrium position of the remaining carriage change?

We started talking about the principle of work this week in my theoretical physics class and this is one of the weekly exercises we are dealing with.

First I used Inkscape trying to draw the situation. It should be something like this:

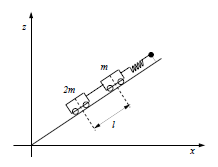
The angle between the plane the carriages are on and the xy-plane is 45°.

My ideas so far:

a) Since they are talking about railway carriages I supposed the carriages are on rails, meaning they have no degree of freedom along the y-direction, correct? Their only degree of freedom should be along the "plane" $z=x$ if I'm not mistaken.

b) I'm not quite sure how to get the equilibrium positions of the carriages. I don't have anything given besides the distance between the carriages basically, well and the fact that the spring stretching is based on a linear power law. Not sure how to approach this. Also I don't fully understand the concept of virtual work yet. I will have to look into it some more.

c) Well, can't do this one without b) I guess.

Edit: My questions: How do I approach b) and c)? I assume that my idea in a) is somewhat okay, because I can't imagine the carriages having other degrees of freedoms than along the plane.

Answer 1

a) You're correct. Since the two carriages AND the spring can only move in one dimention, the system has only one degree of freedom. But wait. They are not moving in a plane! (that is a two domentional space), but in a straight LINE (that has only one dimention). *I know, this is only semantics.

b) When you are told that the spring is based on a linear power you can think about it as a spring obeying Hooke's law F = -k dx , where 'k' is a constant determined by what the spring's made of, dx is the displacement position of the spring from its relaxed point (how much is compressed or expanded), the minus sign says that it responds in the oposite way you deform it, and F is the force (so the dimentions of 'k' must be [force/distance]). (m + 2m) * g * Sin(45°) = (3m/sqrt(2)) * g

We can state that (as the system is in equilibrium) the the spring responses with the SAME FORCE in the OPOSITE DIRECTION. So -k dx = (3m/sqrt(2)) * g, and

dx = (3m/sqrt(2)) * g/k

So the position of the second car is

dx + l = ((3m/sqrt(2)) * g/k) + l

and the position of the first car is just

dx = (3m/sqrt(2)) * g/k

c) When the coupling breaks, The remainig car excerts on the spring a force

m * g * Sin(45°) = m * g / sqrt(2)

And the spring responds with

-k dx = m * g / sqrt(2)

So this time

dx = m * g / (k * sqrt(2))

So this is the position of the first car alone.