0
$\begingroup$

I have seen whenever we solve for forces on pulley by rope we take the force on pulley exactly as the tensions in the rope around it. But , why do we do this ? Exactly how does the rope exerts forces on pulley ? enter image description here

Left side of the figure shows an arbitrary situation.Right side shows force on pulley from the rope. (For simplification assume rope and pulley(frictionless) to be massless, i have depicted only an arbitrary situation and i am focussing only on rope and pulley there maybe other forces on the pulley .)

Improve this question
$\endgroup$
10
  • $\begingroup$ Is the center of the pulley constrained? $\endgroup$
    – Ian
    Commented Jun 16, 2015 at 13:39
  • $\begingroup$ @ Ian It may or may not be .Alpha and beta may change with time but the above diagrams are only meant for an instant. $\endgroup$
    – Robin Hood
    Commented Jun 16, 2015 at 13:42
  • $\begingroup$ I would assume that it is. The pulley is hinged and hence the weight of the pulley is really irrelevant in a static situation. The vector sum of the tension + weight of the pulley is being balanced by the hinge reaction. Rope transmits tension (think pulling) evenly due to its very nature $\endgroup$
    – chilljeet
    Commented Jun 16, 2015 at 13:44
  • $\begingroup$ @RobinHood in that case the weight becomes relavant and the vector sum of the tensions + weight of the pulley accelerates the pulley in the direction of the vector at the instance in consideration $\endgroup$
    – chilljeet
    Commented Jun 16, 2015 at 13:46
  • $\begingroup$ @chilljeet can you please elaborate , and exactly how does the rope exerts force on the pulley i mean the rope is in contact with the pulley but how do we know that the force it exerts on pulley is as shown in the diagram ? $\endgroup$
    – Robin Hood
    Commented Jun 16, 2015 at 13:50

1 Answer 1

Reset to default
5
$\begingroup$

Let's assume that the center of the massless pulley is fixed. Consider the force on any small piece of the massless rope. Since the rope is massless, by Newton's Second Law, in order for the rope to not have infinite acceleration the forces on any small piece of the rope must be balanced. Therefore, whatever tension is felt from the left on any given piece of the rope is also felt from the right. In this way, every small piece of rope feels the same forces from the left and the right. By Newton's 3rd Law, any small piece of rope also exerts the exact same forces on the pieces of rope to the left and right. In this way, the tension is uniformly felt throughout the rope.

Now what happens at the pulley, you might ask? The pulley only exerts a normal force on the rope which is perpendicular to the direction of the rope. Therefore, the normal force between the pulley and the rope does not change the uniform tension in the rope, which is parallel to the direction of the rope. In this way, the tension is uniformly felt through the rope as above.

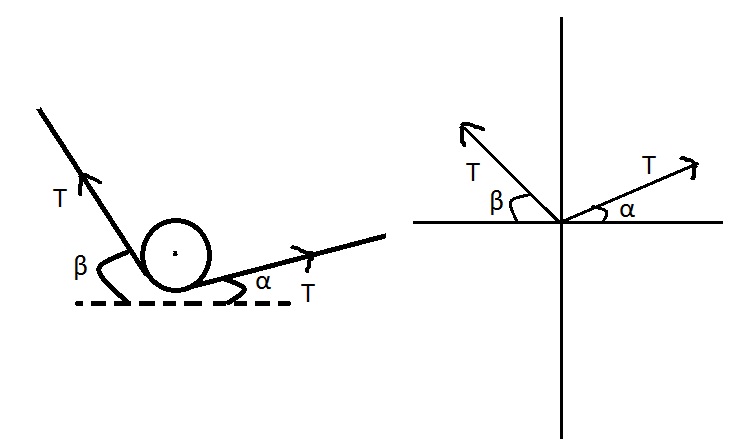
Edit: "Can you give an analysis of the forces between small elements of rope and pulley ?" The most important thing is that, because the rope is massless, the force everywhere on the rope must be balanced. Consider the part of the rope that is curved around the wheel. If we consider any small piece of the rope touching the wheel, we'll see that the forces on the left and right due to the neighboring pieces of rope can't cancel each other out since they are not directed exactly antiparallel to one another due to the curvature of the rope. Therefore, the net force on the small piece of rope is only zero if the normal force between the rope and the wheel balances the forces from the right and left described above. You can probably convince yourself of this by drawing a picture. You might even break the rope into many infinitesimal pieces, find the normal force on each small piece of rope, integrate over all pieces of the rope, and find that magnitude of the vector sum of the normal force between the wheel and rope is equal to $2T\cos \frac{\pi -\beta -\alpha}{2}$. If you want to REALLY understand this problem, that might be a worthwhile exercise.

Improve this answer
$\endgroup$
5
  • $\begingroup$ if you could edit your answer to explain the force felt by the pulley - inturn transferred to the hinge and balanced by it, it would make it clearer. $\endgroup$
    – chilljeet
    Commented Jun 16, 2015 at 14:06
  • $\begingroup$ @Ian i know that tension is uniform but what i am asking for is how those small elements of rope in contact with the pulley give the total force on the pulley from the rope as depicted in the diagram(or i should say how the forces on pulley from rope sum up ?. Can you give an analysis of the forces between small elements of rope and pulley ? $\endgroup$
    – Robin Hood
    Commented Jun 16, 2015 at 14:08
  • $\begingroup$ @Ian exactly that was my question and i REALLY want to understand this problem .Thanks a lot for your time! Can you suggest some good sources from where i can see if the integration i have done is correct and that there are not any conceptual errors ? $\endgroup$
    – Robin Hood
    Commented Jun 16, 2015 at 14:36
  • $\begingroup$ I don't have any sources in mind, but you might as well try it out and see that you get the tension you'd expect from simply assuming that the tension in the rope is T everywhere to the left and right. In essence check the macroscopic picture with a microscopic analysis. Do the integral and post it here and I'll look at it. $\endgroup$
    – Ian
    Commented Jun 16, 2015 at 14:37
  • $\begingroup$ @Ian ok. Can you please look at this problem too ? [link] physics.stackexchange.com/questions/189683/… $\endgroup$
    – Robin Hood
    Commented Jun 16, 2015 at 14:50

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.