Loopwise expansion of effective action $\Gamma[\phi]$

Question

My question is about the loopwise expansion of the effective action $\Gamma(\varphi)$ up to 1-loop contributions. I've understood well the results for both $Z[J]$ and $W[J]$ functionals loopwise expansions. But then something is missing when I follow the path towards the expansion of the effective action. Following this excerpt from Zinn-Justin's "Quantum field theory and critical phenomena":

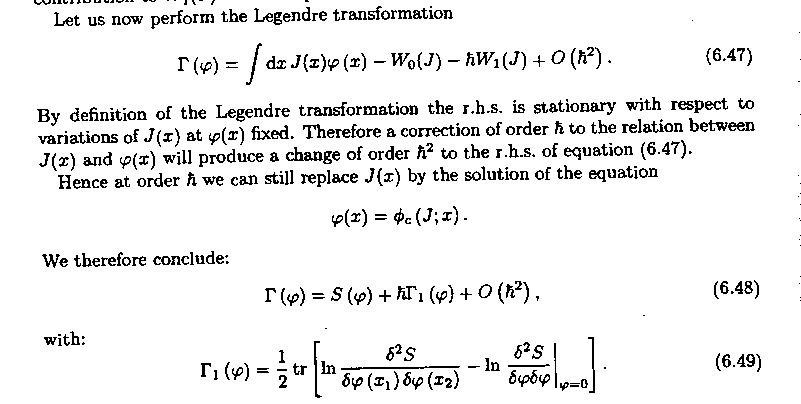
I don't understand the statement: "Therefore a correction of order $\hbar$ to the relation between $J(x)$ and $\varphi(x)$ will produce a change of order $\hbar^2$ to the r.h.s. of equation (6.47)"

Could someone please write down some more details?

My undestanding at this stage is that the relation between $J(x)$ and $\varphi$ is fixed by the stationarity of the (6.47) (as for any Legendre transformation). But then, how to proceed?

Answer 1

The problem is to evaluate $\Gamma[\phi]$ at fixed $\phi$ given an expansion of $W[J]$ in powers of $\hbar$, where $\Gamma[\phi]$ is the Legendre transform of $W[J]$. By definition, $$ \Gamma[\phi]=\sup_{J}\Big[\phi\cdot J-W[J]\Big]. $$ Suppose that the expression in the RHS attains its maximum at some $\hat{J}$. Then formally, we can expand $\phi\cdot J-W[J]$ about $\hat{J}$ to obtain $$ \phi\cdot (\hat{J}+\delta J)-W[\hat{J}+\delta J]=\Gamma[\phi]+\phi\cdot\delta J-\frac{\delta W}{\delta J}\Big|_{\hat{J}}\cdot\delta J+\mathcal{O}(\delta J^2). $$ Since a maximum is attained at $\hat{J}$, the linear term $(\phi-\delta_J W)\cdot\delta J$ vanishes for arbitrary $\delta J$, and we see that corrections start at order $\delta J^2$.
Now we return to the expansion in $\hbar$. If $W[J]$ has an expansion, then it is reasonable that $\hat J$ has an expansion too after solving $\delta_J W=\phi$. In fact, if $\hat J$ has an expansion in $\hbar$ then the zeroth order term must be the solution to $\delta_J W_0 = \phi$, where $W=W_0+\hbar W_1+\dots$. Hence, we can write $\hat J_0=\hat J-\hbar \delta\hat J$, where $\delta \hat J$ includes all higher order corrections. From the above argument, evaluating $\phi\cdot J-W[J]$ at $\hat J_0$ will differ from the evaluation at $\hat J$ by terms of order $\hbar^2$. Hence, to order $\hbar$ we can use $\hat J_0$ in evaluating $\Gamma[\phi]$.