1
$\begingroup$

From Wiki and from physics fundamentals lections I received info that pressure is scalar value. But in definition you have relation between projections of two vector values to normal axe-force and surface area with normal orientation. Are you interesting only in absolute values of this two vectors? (if so then the pressure is always positive.)

Can pressure be negative?

I don't want to discuss the averaged model of the pressure compare to tension elastic model. At current moment from the post answers and comments I realized that pressure scalar field can be defined so:

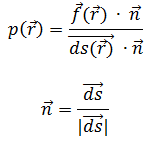
enter image description here

$f$ is support force to support equilibrium in $ds$ surface under other molecules attack. pressure scalar value in some circumstances can be negative. If smth. was wrong here please let me know!

Improve this question
$\endgroup$
8

2 Answers 2

Reset to default
1
$\begingroup$

Your sources were probably trying to keep you from getting confused when they threw in a minus sign later. Typically you deal with a positive scalar pressure which doesn't have a direction. But like if you're doing a fluid mechanics problem and you've just calculated one pressure and you've got other areas of interest, say the other side of a divider, and the teachers throw in the negative.. It tells you which direction the pressure acts. Also you would typically think of pressures as resulting from a gass or a liquid trying to expand in some container. But as noted here Is negative 20 psi / 1.5 bar possible? it'd be reasonable to consider something pulling in on the walls and you could call that a negative pressure.

Improve this answer
$\endgroup$
2
  • $\begingroup$ So your answer is: "yes, it can be. You take in account signs of projections"? p.s. Thanks for link. $\endgroup$
    – Konstantin Burlachenko
    Commented Jun 10, 2015 at 10:07
  • $\begingroup$ I can't type equations in latex in comments, so I'll type it in question $\endgroup$
    – Konstantin Burlachenko
    Commented Jun 10, 2015 at 12:31
0
$\begingroup$

From the definition of temperature from statistical physics and thermodynamics we have:

$$P=T{\partial S(E,V,N) \over \partial V} |_{E,N}$$ where P is the pressure, T is the temperature, E is the energy of the system. So the temperature will have a negative value if

1)The derivative of entropy over volume change is negative, or

2)The absolute temperature is negative

As for the 1), i don't know if it's sign can become negative(an increase in volume meaning a decrease in entropy or the opposite). But for the second, there is an enteire research going on for negative temperatures which suggest also negative pressures.

Hope this helps.

Note: See,if you are interest: http://arxiv.org/abs/1211.0545 , http://physicscentral.com/explore/action/negative-temperature.cfm , Showing existence of negative temperature for a quantum system, Are negative temperatures typically associated with negative absolute pressures?

Improve this answer
$\endgroup$
1

Not the answer you're looking for? Browse other questions tagged or ask your own question.