I was recently looking for answers as to why time slows down the faster you travel and regularly came across the mirror clock experiment. This experiment has a beam of light bouncing between two mirrors as the mirrors travel relative to a stationary observer. The experiment says that from the point of view of the stationary observer the light has to travel a longer distance, down on an angle (due to the sources movement) then after hitting the mirror, backup on an angle to hit the top mirror - ad infinitum. However I thought that the speed of light is the same in all reference frames and not affected by the source. So if correct (I assume I'm wrong here) wouldn't the light still go straight down relative to the stationary observer, and essentially the moving clock would move away from the light?
-
$\begingroup$ Can you give any text quotations, from the textbook. It sure is the stationary not the moved observer who's meant? $\endgroup$– Peter BernhardCommented Nov 14, 2022 at 20:34
2 Answers
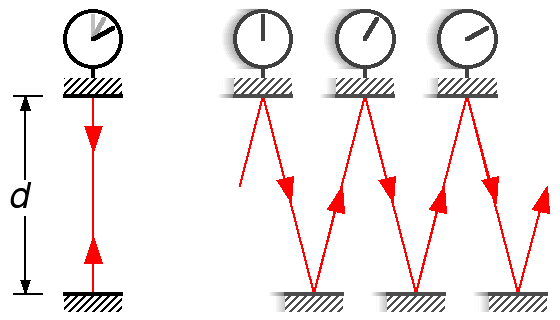
No - the light is moving with the mirrors. To an observer in the mirror / clock frame, because they're in the same frame the light will appear to only move from one mirror to the other (up/down).
However an observer not traveling with the clock, would still observe that inside the clock system (mirrors and the trapped light) the light still moves only from one mirror to the other.
What's different?
In the clock frame, the distance you observe the light to travel is the distance between the mirrors. But to an outside observer, it is more than that, because between each bounce the entire clock system (mirrors and light) has moved forward for that observer.
The part you seem to be confused by is that if the clock were to start moving, then yes, the light wouldn't accelerate with it necessarily. The experiment tends to neglect this detail and assumes that the clock has always been moving.
-
$\begingroup$ Thanks. These experiments always show the light moving WITH the clock which suggest to me that even though the person riding with the clock would see the light going up and down, it is actually travelling through space with the clock and therefore on an "angled" trajectory and therefore is actually travelling the same distance for everyone. I feel there's some fundamental concept I'm not understanding here. $\endgroup$– AKBCommented May 28, 2015 at 3:38
-
$\begingroup$ @AKB Ok, try to look at it using just the clock (C) and the stationary observer (S) ignoring for a moment what the light is doing. S thinks C is moving and S isn't; C however thinks S is moving and C isn't - this is what relative motion implies, that everything will observe everything else to be in motion and itself to be stationary. So, from C's frame, the light will only have the vertical motion because C thinks C is stationary and S isn't, while S sees C (hence the light as well) having additional motion (left-right) and calculates a larger distance traveled for the light. $\endgroup$ Commented May 28, 2015 at 3:54
-
$\begingroup$ What follows is because S sees a longer path for the light, it sees a longer duration between the bounces. However C will observe a shorter duration than that, because light must travel at the same rate (distance per time) for both S and C. In fact if S also had a light clock, both S and C would argue the other has a longer duration between bounces, leading to situations like the twin paradox. $\endgroup$ Commented May 28, 2015 at 4:01
-
$\begingroup$ Thank you. I understand the experiment so long as I accept the fact that things moving with C are actually stationary, but I always say to myself "They're not, they're moving through space, I don't care what C thinks is happening". I guess I struggle to think truly relatively and struggle to accept that C (And things travelling with it) are actually standing still and NOT moving AT ALL. Thinking some more, maybe I need to just accept that NO, C IS stationary, and S is moving (Relative to C). I guess in this case I have to accept relative motion as a physical truism. $\endgroup$– AKBCommented May 28, 2015 at 4:11
-
$\begingroup$ I like the rather unusual explanations refering to "knowing" and "ignoring". In fact, isn't "stationary observer" used as a word to denote the position of the on who "shutters up and calculates" (Feynman quote: shut up and calculate). Otherwise the stationary observer would be some other ineratial frame that would have to consider its own movement (of the train stations he's "waiting on"). Seriously, as it occurs: Stationary observer might want the Lorentz transformation or the Doppler transformation. Some frame must do the "unification", set the norm. - Within the mirrors, it's curvature. $\endgroup$ Commented Nov 14, 2022 at 20:40
The point of that experiment is not that the light goes slower but that the light has a longer distance. This means that a single bounce of the light off the mirror takes longer for the observer's point of view. This is due to time dilation. So it is not because the light is slower, but it is because the light has to travel longer
-
$\begingroup$ I think the OP's confusion is about the change in direction that leads to longer path length $\endgroup$ Commented May 27, 2015 at 1:18