What is meant by the phrase "this operator does not renormalize this other operator", and how can understand it using diagrammatic arguments?

Question

I am trying to understand some sentences in a paper. In section two the following theory of a (complex) massless scalar coupled to a $U(1)$ gauge boson is introduced $$\cal{L}_4=-|D_{\mu}\phi|^2-\lambda_{\phi}|\phi|^4-\frac{1}{4g^2}F_{\mu\nu}^2\qquad{}\cal{L}_6=\frac{1}{\Lambda^2}[c_rO_r+c_6O_6+c_{FF}O_{FF}]$$ where $\Lambda$ is an energy scale suppresing the dimension 6 operators and $$\cal{O}_r=|\phi|^2|D_{\mu}\phi|^2\qquad{}O_6=|\phi|^6\qquad{}O_{FF}=|\phi|^2F_{\mu\nu}F^{\mu\nu}$$ What I want to understand is what is meant in the last paragraph of the same page

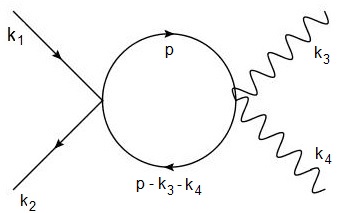
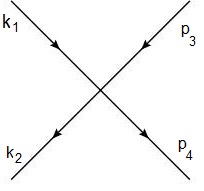
"Many of the one-loop non-renormalization results that we discuss can be understood from arguments based on the Lorentz structure of the vertices involved. Take for instance the non-renormalization of $\cal{O}_{FF}$ by $\cal{O}_R$."

my first question is, what is exactly meant when they say that an operator doesn't renormalize the other? ( I somehow suspect this has something to do with the renormalization group but since my knowledge on this matter is very recent I would like as explicit an explanation as possible)

the paragraph continues

"Integrating by parts and using the EOM, we can eliminate $\cal{O}_r$ in favor of $\cal{O}'_r=(\phi{}D_{\mu}\phi^*)^2+h.c..$ Now it is apparent that $\cal{O}'_r$ cannot renormalize $\cal{O}_{FF}$ because either $\phi{}D_{\mu}\phi^*$ or $\phi^*{}D_{\mu}\phi$ is external in all one-loop diagrams, and these Lorentz structures cannot be completed to form $\cal{O}_{FF}$."

This whole part confuses me. I want to know how do these diagrammatic arguments arise in this context and how can I learn to use them (it would be nice also if someone pointed out which are "all one-loop diagrams" that are mentioned.

Answer 1

Consider for example, simple $\lambda \phi^3$-theory with Lagrangian $$ \mathcal{L}=-\frac{1}{2}\left|\partial_\mu\phi\right|^2-\frac{m^2}{2}\phi^2+\frac{\lambda}{3!}\phi^3. $$ One can say that the $\lambda \phi^3$ term renormalizes the mass term, because the regularization and renormalization of the divergence of the one-loop diagram

will lead to a renormalized mass in the propagator.

In the theory you described, the operator $\mathcal{O}_{\mathcal{FF}}=|\phi|^2F_{\mu\nu}F^{\mu\nu}$ leads to a vertex of the following form:

In the Feynamn rules in momentum space it comes with a factor of $\frac{c_{\mathcal{FF}}}{\Lambda^2}{k_3}_\mu {k_4}_\nu$. The momentum factors are due to the derivatives in $F_{\mu\nu}$. The arrows on the scalar lines correspond to the flow of the conserved $U(1)$ charge and they keep track of the $\phi$ and $\phi^*$. We note in particular that this vertex does not depend on the external momenta of the scalar $k_1$ and $k_2$. These enter only through overall momentum conversation at the vertex.

The coupling $c_{\mathcal{FF}}$ can now get renormalized by divergences in diagrams with the general configuration

where the dependence on the external legs has to be the same as for the vertex above. In particular, the diagrams contributing to the renormalization of the coupling $c_{\mathcal{FF}}$ cannot depend on the scalar external momenta $k_1$ and $k_2$.

Before the integration by parts, there is the vertex

in the Feynman rules coming with the factor $\frac{c_r}{\Lambda^2}{p_3}_\mu{p_4}^\mu$. Note, that the two contributing momenta come from one line with inflowing charge and one line with outgoing charge, because in $\mathcal{O}_r=|\phi|^2|D_{\mu}\phi|^2$, the derivative acts once on $\phi$ and once on $\phi^*$. You can use this vertex to construct a diagram contributing to the renormalization of $c_{\mathcal{FF}}$ in the following way:

so the factor coming from the vertex in this case does not contain $k_1$ and $k_2$.

After integrating by parts, the $\mathcal{O}'_r$

comes with a vertex factor of $\frac{c_r}{\Lambda^2}{k_2}_\mu{p_4}^\mu$ (or$\frac{c_r}{\Lambda^2}{k_1}_\mu{p_3}^\mu$ from the complex conjugate contribution) because now the derivatives act on two $\phi^*$ (or on two $\phi$'s in the complex conjugate contribution). The vertex factor will now always contract the external momentum of one of the scalar legs, so after this reformulation using an integration by parts, the above loop diagram can not renormalize $c_{\mathcal{FF}}$ any more, because the scalar vertex will always contract one of the external scalar momentum if charge conservation is to be obeyed in the loop (i.e. one $\phi$-leg and one $\phi^*$-leg point towards the loop.)

Thus, $\mathcal{O}_{\mathcal{FF}}$ can also not be renormalized at the one-loop level in the original formulation (where it is not obvious), as the two formulations are ultimately equivalent.