Why does the electromagnetic and weak coupling strength do not meet at the electroweak scale?

Question

The running of the coupling strengths is usually visualized on a logarithmic scale like here

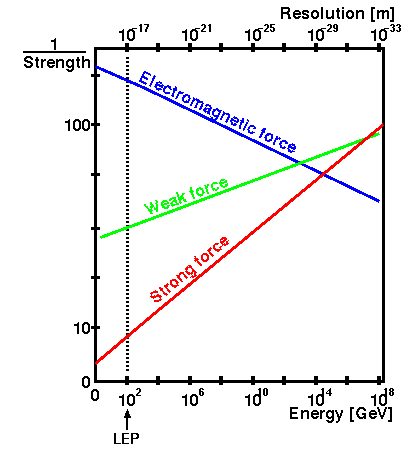
What surprises me is that the weak and the electromagnetic coupling strength do not meet before the GUT scale. Why is this the case?

A common argument in Grand Unified Theories is that all elementary forces meet at some energy scale. Above this threshold we have only one interaction, describe by a gauge group $G$ and correspondingly only one coupling strength. The symmetry gets broken spontaneously to the standard model gauge group $ G \rightarrow SU(3) \times SU(2) \times U(1)$ at lower energies, the coupling strength split and the new gauge bosons and possibly exotic fermions get a mass comparable to the GUT scale (this is called survival hypothesis).

Now, this is speculative beyond the standard model stuff, but in the standard model something very similar happens. The standard model gauge group $SU(3) \times SU(2) \times U(1)$ gets broken at energies below the electroweak scale.

$$SU(3) \times SU(2) \times U(1) \rightarrow SU(3) \times U(1) $$

Most books and papers talk about a unified electroweak interaction. Shouldn't this mean that the electromagnetic and weak coupling strength get unified?

And bonus: Shouldn't all fermions and bosons get a mass comparable to the Electroweak scale? Even without the neutrino the mass difference between the lightest (electron) $\approx 0,5 \cdot 10^{-3}$ GeV and heaviest (top) $\approx 170$ GeV is six orders of magnitude.

Answer 1

Answer to the main question:

It is a well regarded fact that the terminology unified electroweak interaction is a bit of an abuse of terminology. What the term means is that both Quantum Field Theories, the Hypercharge ($U(1)_Y$) and Weak ($SU(2)_L$), are unified in a common framework, which predicts the low energy electromagnetism ($U(1)_{em}$) through the Higgs mechanism $$ U(1)_Y \times SU(2)_L \to U(1)_{em}$$ It does not refer to an unification scenario as in the Grand Unified Theory (GUT) setup, it's meant to refer to an unification in the sense Weak decays and Electromagnetism are understood as remnants of a higher-energy theory, the Standard Model.

In comparison with GUTs the terminology can be applied if you think that regular GUT setups predict unification into a gauge group which is composed of solely one (semi) simple Lie group, e.g. $SU(5)$, $SO(10)$ being 2 of the most popular. In this sense the couplings do unify. The electroweak unification can be regarded as a unification into a group with 2 (semi) simple Lie group factors, the $SU(2)$ and $U(1)$. It is in this way of thinking about it that people refer to as unification. Notice that in the later case each factor can has its own coupling, and so the couplings are not equal, i.e. do not unify.

Answer to the bonus question:

What you asked is a big open question. Fermionic masses come from Lagrangian terms called Yukawa couplings, for example for the electron $$ y H L e+\mbox{h.c.}$$ for example, and the masses are then $$ m \sim v y$$ where $v$ is the vacuum expectation value of the Higgs field, $H$, and $y$ are the Yukawa couplings are not specified in the Standard Model and one should expect them to be of order 1. But this only happens to the top quark, while all the other fermions have a lot smaller (in some cases many orders of magnitude smaller) than 1. Why this is like this is still an open question in Physics.