Why can't a magnet change a charged particle's speed?

Question

I know that magnetic force acts perpendicular to the direction of the original velocity, so the velocity in that original direction is unchanged, but once the magnet starts acting, the particle's velocity in the direction of the force goes from zero to nonzero -- wouldn't that increase the magnitude of the resultant velocity vector?

Answer 1

I know that magnetic force acts perpendicular to the direction of the original velocity

No, the magnetic force acts perpendicular to the current velocity. Once the direction of the velocity changes, the direction of the force changes as well.

Cast in math:

$$ m\dot{\vec v} = \vec F_L = \frac q c \vec v \times \vec B $$

From this we get ($v = |\vec v|$):

$$ \dot v = \partial_t \sqrt{\vec v^2} = \frac{\vec v \cdot \dot{\vec v}}{\sqrt{\vec v^2}} \propto \vec v \cdot (\vec v \times \vec B) = 0$$

(As $\vec v \times \vec B \perp \vec v$).

Answer 2

Since $\vec{F}=m\vec{a}$, the force of the magnetic field causes the charged particle to accelerate. An acceleration, however, is a change in velocity, not necessarily a change in magnitude of the velocity. If the force is parallel to the velocity, the magnitude of the velocity will change. If the force is perpendicular, only the direction of the velocity will change (like in a uniform circular motion.)

If you want the mathematics, you should consider the moving particle, a fixed frame x,y and a frame t,n that moves along with the particle, with the t-axis always parallel with the velocity (and hence, the n-axis always perpendicular to the velocity). In this moving frame, the velocity is: $$\vec{v} = v . \vec{e}_t$$

In general $$\dot{\vec{v}} = \dot{v} . \vec{e}_t + v. \dot{\vec{e}_t}$$

But if only the magnitude changes this becomes $$\dot{\vec{v}} = \dot{v} . \vec{e}_t$$ So a change in magnitude of the velocity is caused by an acceleration parallel with the velocity.

If only the direction of the velocity changes, it becomes $$\dot{\vec{v}} = v . \dot{\vec{e}_t}$$

To calculate this, you need to express t and n in terms of the fixed frame x,y: $$\vec{e}_t = \cos\phi .\vec{e}_x+\sin\phi.\vec{e}_y\\ \vec{e}_n = \cos(\phi+\pi/2). \vec{e}_x+\sin(\phi+\pi/2).\vec{e}_y=-\sin\phi .\vec{e}_x+\cos\phi.\vec{e}_y$$

If you now calculate the derivative: $$\dot{\vec{v}} = v . \dot{\vec{e}_t}= v . \dot{\phi}.\vec{e}_n$$ So a change in direction of velocity is caused by a perpendicular acceleration.

Answer 3

Simply:

The magnetic field does no work on a charged particle, because it's always normal to the particle trajectory. Work equals the change in kinetic energy...i.e. no change in speed.

Answer 4

Maybe one intuitive explanation is that the change in velocity will be $O(\Delta t)$, while the change in speed during a little time $\Delta t$ will be $O(\Delta t^2)$. In fact if the velocity $\mathbf v =v_x\hat x$ is initially in the $x$ and the acceleration in the $y$ direction, then after $\Delta t$: $$\Delta v^2 \approx v_x^2+(a\Delta t)^2-v_x^2=a^2 \Delta t ^2$$and it is easy to show that if $\Delta v^2=O(\Delta t ^2),$ then also $\Delta v = O(\Delta t ^2)$. Now suppose we integrate numerically the motion of the particle for a total time $T$; after each step the speed of the particle $v=\sqrt {\mathbf v\cdot \mathbf v}$ will be increased by an amount proportional to $\Delta t ^2$. The number of integration steps will be proportional to $T/\Delta t$, so the total increase in speed will be proportional to $\Delta t$ and will vanish in the limit $\Delta t \to 0$.

Answer 5

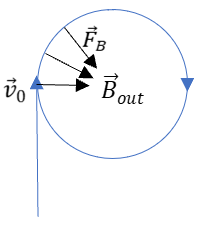
A particle is moving in a straight path when a magnetic field is turned on, exerting a force vxB=F according to the right-hand rule.

For the first time step, the force of the magnetic field, which is perpendicular to the velocity, appears to be increasing the total velocity vector. However, at the second time step, the force vector is pointing slightly backwards against the direction of the initial velocity. If you take these time steps to be infinitely short, then the particle will not increase in speed.

This will in fact happen for any two consecutive time steps, as the force of the second time step will be slightly opposing the velocity vector of the first time step. This has the effect of pulling the particle around in a circle at a constant speed.

Answer 6

Lorentz got a Nobel prize for giving an answer for this. The actual KE depends on the particle rotational direction (Spintronics)) and the magnetic field alignment as well as the Electrical field alignment.