0
$\begingroup$

I'm posting this question as a function of an another question I found here:

What is potential energy truly? The answer of WetSavannaAnimal aka Rod Vance.

As I understand the hole point is to see the interactions of the system and the change of properties so the constants of motion may hold.

But, in the Aharonov-Bohm effect, don't we have a manifestation of the potential and an action from it to the electron outside, even if the field is zero?

So the question is: Does the Aharonov-Bohm effect gives something for the potential(at this example of the effect, the magnetic vector potential) as a physical being?

Improve this question
$\endgroup$
4
  • $\begingroup$ 1. Define physical being. 2. Observe that the phase incurred in A-B may be expressed as an integral of $A$, but really is just the magnetic flux through the loop. $\endgroup$
    – ACuriousMind ♦
    Commented Apr 18, 2015 at 12:18
  • $\begingroup$ By physical being I mean something else than just a mathematical function in space, or something we use for the constants of motion to hold. I mean that it's something there, which can have measurable effects. As for the second I don't understand what you are saying. $\endgroup$
    – Constantine Black
    Commented Apr 18, 2015 at 12:21
  • 1
    $\begingroup$ Possible duplicates: physics.stackexchange.com/q/56926/2451 , physics.stackexchange.com/q/86506/2451 , physics.stackexchange.com/q/99505/2451 and links therein. $\endgroup$
    – Qmechanic ♦
    Commented Apr 18, 2015 at 12:23
  • $\begingroup$ As I understand we have the magnetic field 0 outside but the vector field non 0. From this we see that the vector potential affects the two wavefunctions, thus having some physical interpretation. $\endgroup$
    – Constantine Black
    Commented Apr 18, 2015 at 12:27

1 Answer 1

Reset to default
1
$\begingroup$

What is potential energy truly?

It depends on the circumstances. When you compress a spring it's stress in the bonds or electromagnetic field between the atoms. IMHO at the fundamental level it's essentially spatial stress. That might sound unfamiliar, but it shouldn't, because the stress-energy-momentum tensor "describes the density and flux of energy and momentum in spacetime". However when you lift a brick you do work on it and add potential energy, but this is really just hidden internal kinetic energy in line with Einstein's E=mc². When you drop the brick this is converted into external kinetic energy, then when this kinetic energy is dissipated you're left with a mass deficit, see Wikipedia.

Does the Aharonov-Bohm effect gives something for the potential... as a physical being?

Yes. Again see Wikipedia. Electromagnetic four-potential isn't quite the same thing as potential energy, but it is something very real. As for what it actually is, I'm not sure there's an authoritive definite answer, but I'd say it's something akin to spatial stress-energy-momentum. For an analogy, the four-potential is like the height of the ground whilst the electric field is like the slope, and you can tell when you're on a high plateau.

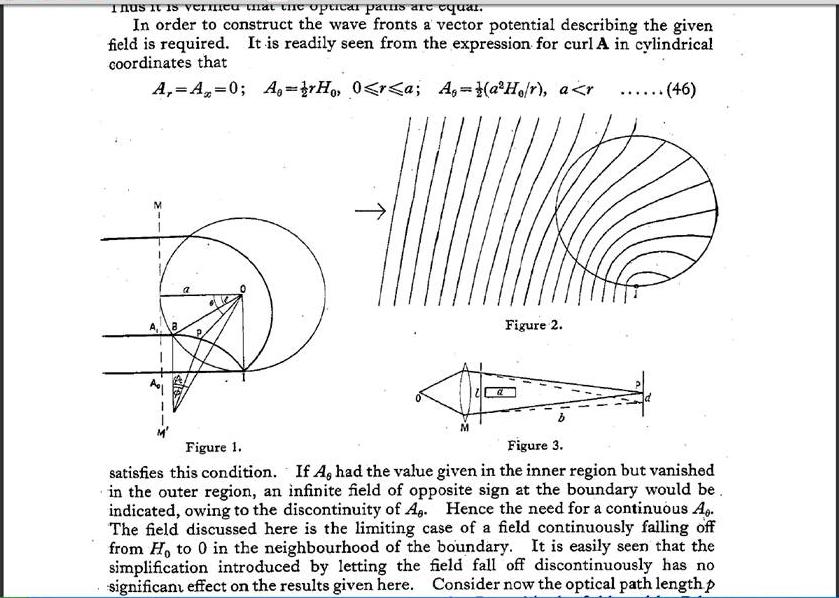
As an aside I should mention that what we call the Aharonov-Bohm effect was in fact predicted by Ehrenberg and Siday in their 1949 semi-classical paper The Refractive Index in Electron Optics and the Principles of Dynamics. Electrons shown as plane waves going past the solenoid are retarded on one side and advanced on the other, see figure 2, so the interference fringes shift as per figure 3:

enter image description here

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.