We all know how to obtain the capacitance $C=\frac{ab}{b-a}$ (ignoring constants) for two concentric spheres of radii $a,b$.
I was just thinking to myself, what would happen to the capacitance for non-concentric spheres?
Suppose we perturbed the inner sphere off the origin. Does capacitance increase? Ideally I would be looking for an explicit calculation (under sufficiently nice assumptions) but I am also guessing it would be a rather ugly formula.
Important notice: My previous result was a little bit incorrect. I found the factor $1/2$ by comparison with the textbook V.V. Batygin, I.N. Toptygin «Problems in electrodynamics».
Let's denote the radius of the inner sphere $S_1$ as $a$, the radius of the outer sphere $S_2$ as $b$ and the displacement as $c$, so that $c\ll a,b$. We choose the origin of the coordinate system to be in the center of the inner sphere. Then, up to the second order in $c$ the distance from the origin to the outer sphere has the form: $$ R\left( \theta\right) =b+c\cos\theta. $$ Therefore, the potential in the space between them can be found as $$ \phi=\left( \alpha_{1}+\frac{\beta_{1}}{r}\right) +c\left( \alpha _{2}r+\frac{\beta_{2}}{r^{2}}\right) \cos\theta, $$ where $\alpha_{i}$ and $\beta_{i}$ are constant which should be found from the boundary conditions: $$ \left. \phi\right\vert _{S_{1}}=const,\quad\left. \phi\right\vert _{S_{2} }=0,\quad \oint_{S_{1}} \mathrm{d}S\,\mathbf{n}\cdot\mathbf{\nabla}\phi=-4\pi Q,\, $$ where $\mathbf{n}=\mathbf{r}/r$. Hence the potential reads as follows: $$ \phi=Q\left( \frac{1}{r}-\frac{1}{b}\right) +\frac{Qc}{b^{3}-c^{3}}\left( r-\frac{a^{3}}{r^{2}}\right) \cos\theta. $$ Therefore the potential on the inner sphere doesn't depend on $c$ up to the second order: $$ \left. \phi\right\vert _{S_{1}}=Q\,\frac{b-a}{ab}. $$ The charge distribution on the inner sphere can be found as follows: $$ \sigma=-\frac{1}{4\pi}\left( \mathbf{n}\cdot\mathbf{\nabla}\phi\right) =-\frac{1}{4\pi}\left. \frac{\partial}{\partial r}\,\phi\right\vert _{r=a}=\frac{Q}{4\pi a^{2}}\left( 1-\frac{3a^{2}c}{b^{3}-c^{3}}\cos \theta\right) . $$ Hence, the force acting on the inner sphere has the form: $$ \mathbf{F}=-\frac{1}{2}\oint_{S_{1}} \mathrm{d}S\,\sigma\mathbf{\nabla}\phi, $$ $$ F =-\frac{Q^{2}}{4}\int_{-1}^{1}\mathrm{d}\cos\theta\,\left( 1-\frac{3ca^{2}}{b^{3}-c^{3}}\cos\theta\right) \left. \frac{\partial }{\partial z}\left[ \frac{1}{r}+\frac{c}{b^{3}-c^{3}}\left( 1-\frac{a^{3} }{r^{3}}\right) z\right] \right\vert _{r=a}\\ =-\,\frac{Q^{2}c}{b^{3}-c^{3}}, $$ where I use the trivial identity: $$ \frac{\partial}{\partial z}\frac{1}{r^{n}}=-\frac{nz}{r^{3}}. $$ The capacity $C$ can be found from the potential energy: $$ U=\frac{CV^{2}}{2}\quad\Rightarrow\quad F=-\frac{\Delta U}{\Delta c} =-\frac{\phi^{2}}{2}\frac{\Delta C}{\Delta c}, $$ thus $$ \frac{\Delta C}{\Delta c}=-\frac{2F}{\phi^{2}}=\frac{2ca^{2}b^{2}}{\left( b^{3}-a^{3}\right) \left( a-b\right) ^{2}}. $$ Finally, we obtain $$ C=\frac{ab}{b-a}+\frac{a^{2}b^{2}c^{2}}{\left( b^{3}-a^{3}\right) \left( a-b\right) ^{2}}\quad\quad (1) $$
UPDATE: The comments given above give the reference to the article of «Capacitance Bounds for Geometries Corresponding to an Advanced Simulator Design» by M.I. Sancer and A.D. Varvatsis. The article in turn contains the reference to the book:
W. R. Smythe, Static and Dynamic Electricity, McGraw-Hill, New York, 1950
where the following exact result for the capacitance is presented: $$ C=ab\sinh\alpha\sum_{n=1}^{\infty}\frac{1}{b\sinh n\alpha-a\sinh\left( n-1\right) \alpha},\quad\quad\left( 2\right) $$ so that $$ \quad\cosh\alpha=\frac{a^{2}+b^{2}-c^{2}}{2ab}. $$ Sancer and Varvatsis claim that they found the approximation of the exact result in the $c\rightarrow0$ limit: $$ C=\frac{ab}{b-a}\left[ \frac{1}{2}\left( \sqrt{\frac{1-y^{2}/\left( 1+x\right) ^{2}}{1-y^{2}/\left( 1-x\right)^{2}}}+1\right) + \frac{x}{2}\left( \sqrt{\frac{1-y^{2}/\left( 1+x\right) ^{2}}{1-y^{2}/\left( 1-x\right) ^{2}}}-1\right) \right] ,\quad\quad\left( 3\right) $$ where $$ x=\frac{a}{b},\quad y=\frac{c}{b}. $$ It is easy to see that the expansion of the result (3) doesn't coincide with mine result (1). The numerical comparison of all three results presented in the figure below:
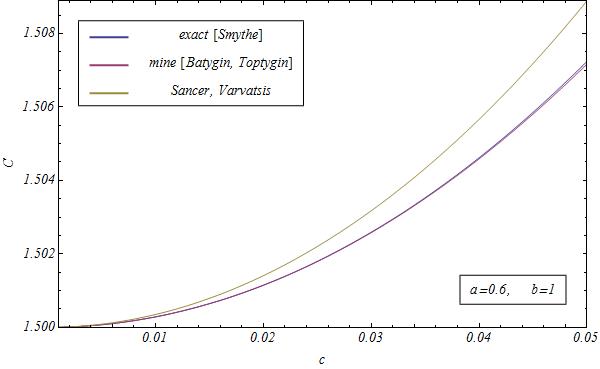
One can see that the result (3) of Sancer and Varvatsis is incorrect.
The whole system is unstable (you can convince yourself by Earnshaw theorem or by drawing the field lines). After you perturb the inner sphere, it'll move all the way until it touches the outer sphere. Since the potential energy is $\frac{Q^2}{2C}$, and it's decreasing along the way -> the capacitance increases.
Here is my estimate for the change in potential energy $\approx -U_0 \frac{b-a}{a}(\frac{\delta}{b})^2$ .
I got this from the change in bulk electrostatic energy $\frac12 E^2 2\pi b^2\delta$
Here I shift the outer conductor because it is easier to follow my calculation this way. The shift is exaggerated for clarity's sake.
Initially, the electrostatic energy comes from region 1 and 2. After the shift, it comes from region 2 and 3. We can roughly see that the energy in region 3 is less than the one in region 1: region 1 is nearer to the inner conductor than region 3.
Clearly the change in energy is
$$U_3 - U_1 = \frac12 (E_3^2 -E_1^2) 2\pi b^2 \delta$$ with $$E_3^2 - E_1^2 \approx 2E_1 \frac{dE}{dr} \delta \approx -4 E_1^2 \frac{\delta}b$$
we get $$U_3 - U_1 = - \frac{e^2}{4\pi b} (\frac{\delta}b)^2= U_0 \frac{b-a}a (\frac{\delta}b)^2$$
This gives us $-\frac{\Delta U}{U}=\frac{\Delta C}C\approx\frac{b-a}{a}(\frac{\delta}{b})^2$.
