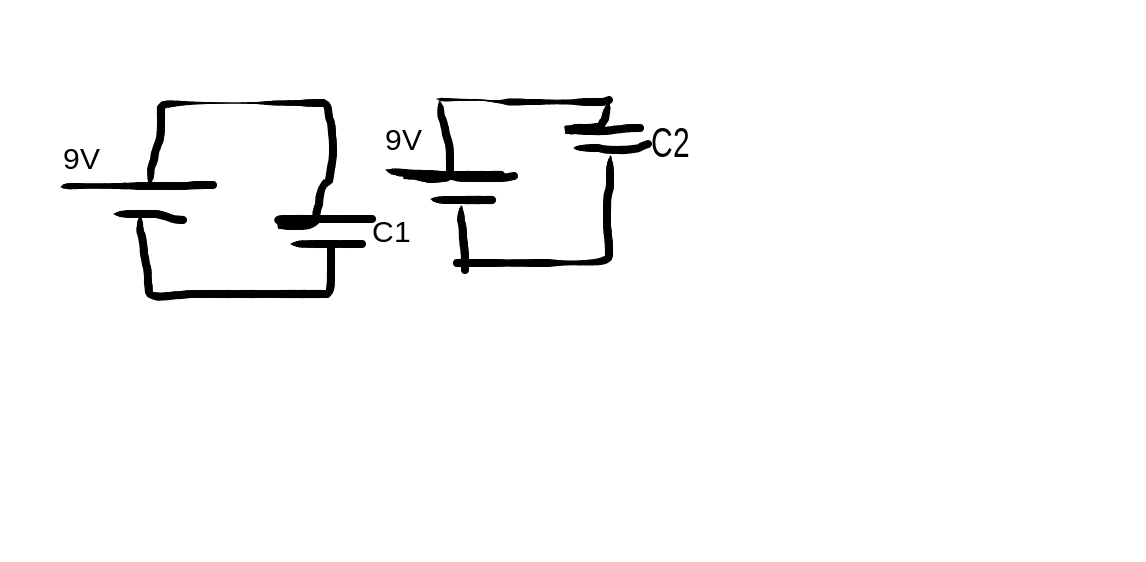
So above are two difference circuits, each with a 9V battery and a capacitor of the same capacitance. $C_1=C_2$
Why is the potential difference across both capacitors 9V? Shouldn't it be less than 9V? The potential difference across the top of the circuit and the bottom of the circuit is 9V. So why is the potential difference along a very tiny capacitor 9V too? The distance between the two capacitor plates arent the same distance as between the top of the circuit and the bottom
For a capacitor with closer plate spacing (all else being equal), the electric field in the dielectric between the plates is stronger.
Assuming a uniform electric field, the potential difference is given by the product of the spacing and the strength of the electric field:
$$\Delta V = E\cdot d $$
So, the potential difference can be the same for both capacitors even though the plate spacing is different but the electric field between the plates will be different.
This is due to the fact that, for smaller spacing, the capacitance $C$ is larger and, thus, more charge $Q$ must be moved by the battery to charge the capacitor to 9V.
$$\Delta V = \frac{Q}{C}$$
The potential difference between the top and bottom of the circuit must be the same as the potential difference between the top and bottom of the "tiny capacitor" as you call it. This is because the wire provides no resistance (theoretically), so the potential difference does not change from the top of the circuit to the top of the capacitor.
On the other hand, it does from the top of the capacitor to the bottom of the capacitor.
