On Earth a pendulum is governed by a set of equations, relating the angle of the swing, the mass etc.
However with a pendulum in orbit there is no fixed point (assume 2 masses attached via a string). If a force is acting on one of the masses (pulling it away from the other mass - analogue to gravity) how does the other mass respond. Intuitively the system would swing like a pendulum, however the net result of this force must in fact be the translation of the masses and string to another location in space.
I've boiled down to a simple problem:
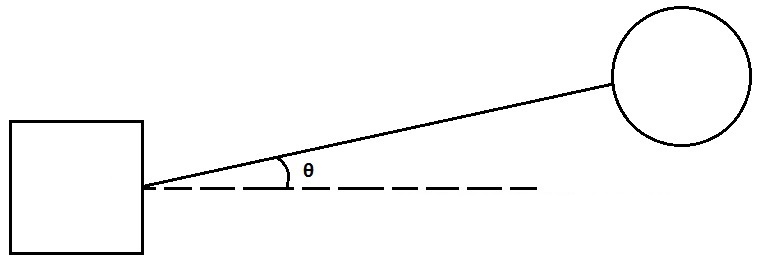
In the above the square mass experience a force along the dotted line away from the round mass. With the offset of theta, what is the force that acts to rotate the square mass so the force aligns with the string?
