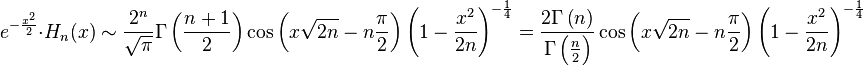3
$\begingroup$

The classical harmonic oscillator obeys an arcsine law in that the distribution of positions of the particle over a single time cycle is proportional to $\frac{1}{\sqrt{A^2-x^2}}$, $A$ being the amplitude.

There is an illustration which seems to be fairly common (I'm looking at figure 2.7b in Griffiths's book on QM) in which a high-$n$ energy eigenstate of the quantum harmonic oscillator is superimposed with the aforementioned distribution. The graphs of the two functions appear to be similar.

Is there a proof that they do coincide in some sense in some limit?

Improve this question
$\endgroup$
1
  • $\begingroup$ Right, classically the energy conservation leads to $\omega dt =\frac{dx}{\sqrt{A^2-x^2}}$, which can be interpreted as the probability distribution $P(x)=\frac{1}{\pi}\frac{1}{\sqrt{A^2-x^2}}$. That's figure 2.5b p. 42 in the 1st edition (1995). $\endgroup$
    – Qmechanic ♦
    Commented Jan 26, 2015 at 22:55

3 Answers 3

Reset to default
2
$\begingroup$

I am not sure about the $ \frac{1}{\sqrt{A^2-x^2}} $ part in you approximation. In the asymptotic limit $n \rightarrow \infty$,the Hermite polynomials behave as follows:

enter image description here

The cosine part relates to the oscillations present in wavefunction which are visible even in fig 2.7b in Griffiths.The $(1-\frac{x^2}{2n})^{\frac{-1}{4}}$ part is the classical behaviour and in this case the graphs seems to match.

References:

Hermite Polynomials on Wikipedia

See the asympotic behaviour part for the above expression.

Improve this answer
$\endgroup$
2
  • 2
    $\begingroup$ Thanks! The exponent discrepancy seems like it comes from squaring the wavefunction. $\endgroup$
    – djk
    Commented Jan 26, 2015 at 6:30
  • 1
    $\begingroup$ @djk You're exactly right. It's the probability amplitude, not the probability density, that's proportional to the Hermite function for the QHO $\endgroup$
    – Selene Routley
    Commented Jan 26, 2015 at 6:56
0
$\begingroup$

I would prefer to approach the classical limit of the harmonic oscillator using the "coherent states". Details can be found in the corresponding Wikipedia article.

Improve this answer
$\endgroup$
0
$\begingroup$

The proof lies in Ehrenfests theorem, which states that quantum expectation values obey classical equations of motion (strictly, if the potential changes slowly over the distance in which the wave function is localized). But such states don't need to look classical at all (like the higher Hermite functions), so it is a bit misleading. As mho points out, coherent states are closer in spirit to classical behavior, and for the special case of the harmonic oscillator have some nice properties.

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.