A typical exercise while introducing the Biot-Savart Law is to calculate the magnetic field caused by a circular current loop at a point P located in its central axis, as shown in the following figure:
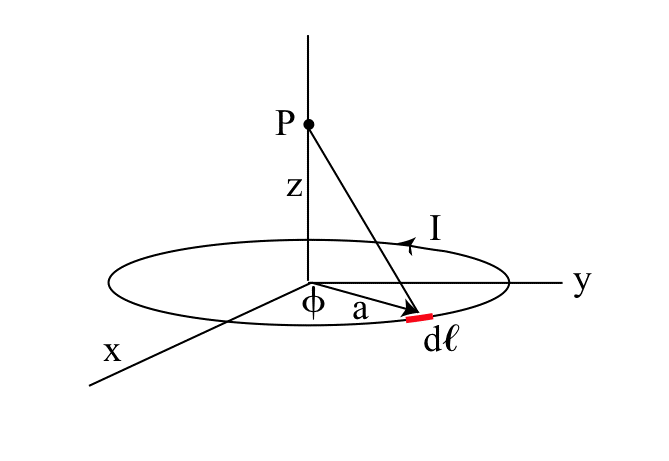
The result is well known:
$$\mathbf{B} = \dfrac{\mu_0 I a^2}{2(a^2+z^2)^{3/2}} \mathbf{\hat{k}}$$
My goal now is to find this magnetic field using Ampère's Law, so what Amperian loop you recommend me to use in order to apply this Law?
Ampere's law is not useful in this case. It says that the line integral of the B field around a closed path is equal to $\mu_0$ times the current passing through the closed path (for steady currents).
To use the law you want the LHS to be simple to evaluate. Usually the B field is constant in magnitude around the path and either parallel or perpendicular to the path. In this case you cannot arrange this. As you have shown with the B-S law, the B field varies with distance from the centre of the current loop, so it is difficult to define a simple line integral path that encloses the current.
No.You cannot apply Ampere's law here. Ampere's law can be applied in some special conditions. This is not one of them.(simply)
