What kind of topological invariants are there for $2D-p+ip$ topological superconductor and to which symmetry class it belongs to?
2 Answers
$\begingroup$
$\endgroup$
1
I don't know what is a "p+ip insulator". There is indeed p+ip superconductor, which belongs to the class D and characterized by an integer invariant, the Chern number.
answered Jan 14, 2015 at 9:12
-
$\begingroup$ I meant here superconductor mistakenly I write the insulator instead of superconductor but now I have edited the question. $\endgroup$– 12saCommented Jan 14, 2015 at 12:07
$\begingroup$
$\endgroup$
All topological insulators can be classified according to their symmetry classes. There is time reversal symmetry ($T$) , charge conjugation symmetry ($C$) and the combination $S=T*C$ symmetry. The $T$ and $C$ symmetries can be either positive or negative, i.e the energy spectrum may change sign under the symmetry operation.
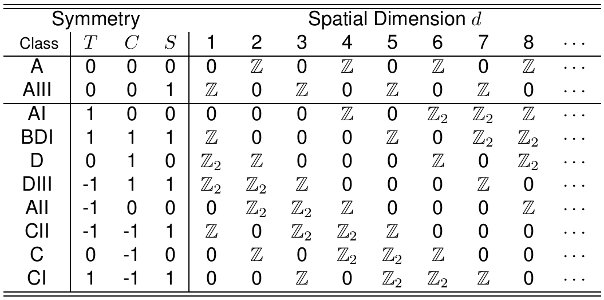
The $p+ip$ superconductor is a $p$-vave superconductor and has positive $C$ symmetry and therefore falls into symmetry class $D$. From the table if the follows that it has an $\mathbb Z$ valued topological invariant.
