I like your question because it puzzled me a lot when I started to learn electronics. Here is the answer.
Just imagine a wire. There is a voltage V applied to its ends. What is the voltage at every section of the wire? If it is uniform wire, every meter of wire causes the same amount of voltage drop. Say, you divide the wire into n sections so that there is V/n voltages per every section or V/n * d if you measure voltage between d consequtive sections. You just add up d voltage drops together. When d=n, you measure voltage along the whole wire and it is not surprising that it equals to V/n * n = V the voltage that you apply. It is fine because you read what you apply.
Just take a very small voltage battery, make a short circuit using a very long wire and measure voltage, sliding with probes along the wire. You'll understand how voltages across non-intersecting intervals add up.
I am just explaining that voltage drop along the whole wire consists or breaks down into voltage drops at its sections. If there is a section which causes some voltage drop then the rest of the wire will expose less voltage drop so that total voltage drop equals the one that you apply at the ends. In your setup, capacitor and resistor amount almost all the input voltage that you apply to the wire. They take up most of the voltage drop so that there is almost nothing is left to drop at the rest of the wire because wire is considered an ideal conductor in this case and ideal conductors do not expose any voltage drops (unless they are loops and coils, which expose inertial 'resistnce' to the current build up, likewise 'resistence' of a massive body -- bodies, like swing, do not fall immediately on the ground despite of virtually no friction just because they have some inductance/inertia, which impedes the immediate acceleration of speed $v \to\infty$.)
Now, you say that element at the left end causes some voltage drop, whether it is $V_R$ or $V_C$. So, the rest of the wire, exposes $V_{in}$ - $V_{first_element}$ drop. You take it as your $V_{out}$. But $V_{out}$ is nothing more than voltage drop at the second half of the wire.
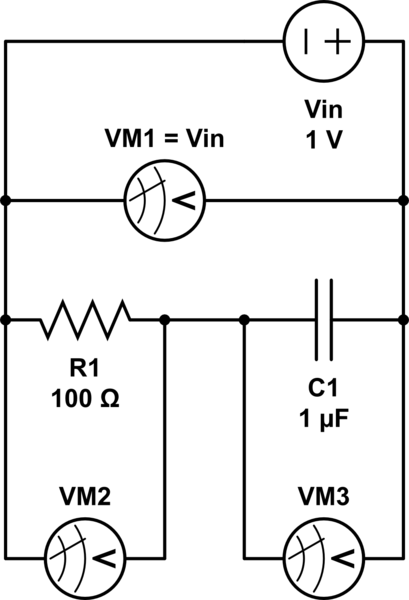
Here is illustration that is somewhat easier to understand. You apply the voltage to two series loads and measure the input voltage with VM1. It should equal the $V_{in}$ voltage that you apply. The two other voltages that you can get are the voltage drops at the two loads. Note that load on the right, whose voltage is measured by VoleMeter3, is what you define as $V_{out}$. You apply 'input' voltage that you have to both loads and read desired 'output' voltage from one of them.