1
$\begingroup$

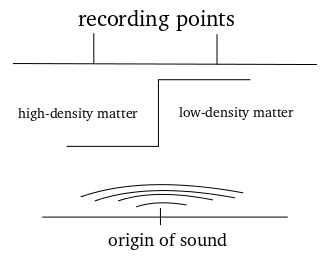
Two superimposed sounds (at source: $s(t) = s_1(t) + s_2(t)$; the two sound components overlap completely in time, partially in spectra) travel through low- and high-density matter and are recorded from two different positions (see figure). Knowing the two different densities $d_1$ and $d_2$ (I don't actually know them yet but it is just a matter of some research), would it be possible to separate the superimposed sounds from each other?

scenario

It is from an actual research problem but this far I have only considered signal processing techniques. I thought people with a different background might see the problem differently. I'd of course acknowledge any contributions that would further tackling the problem.

DSP, including filters and blind source separation has been tested already.

Improve this question
$\endgroup$
6
  • $\begingroup$ Why are the two sounds superimposed? They are recored in two different places, so what mixes them together? There is no reason to mix an air microphone and an underwater microphone signal. $\endgroup$
    – CuriousOne
    Commented Jan 6, 2015 at 21:34
  • $\begingroup$ They are already superimposed at the sound source. It is just known that the situation is so; it would be very desirable to be able to listen to them separately. With signal processing techniques (e.g. independent component analysis), some unmixing is possible to do but I suspect physics could help me even more. $\endgroup$
    – mmh
    Commented Jan 6, 2015 at 21:46
  • $\begingroup$ Without knowing the sound generation mechanism and the spectra and correlation between the two sounds there is almost nothing that you can do with physics. Where the spectra of the two sounds don't overlap, they can be cleanly separated with a linear frequency domain filter, where they do it's not possible. If the spectral ranges overlap but the correlation function is zero or small, one can still use an adaptive time domain filter, even though the theory for that is harder. And if none of that applies, then we are out of luck. $\endgroup$
    – CuriousOne
    Commented Jan 6, 2015 at 21:54
  • $\begingroup$ Too bad. ICA works even with (some) spectral overlap. I was hoping that something could emerge from the fact that sound propagates faster in higher density material (correct me if I am wrong). $\endgroup$
    – mmh
    Commented Jan 6, 2015 at 21:57
  • $\begingroup$ Depends on the mechanism that generates those sounds. The coupling depends on the acoustic impedance, which is high for air and low for dense materials, including water. That's why everything sounds so much louder under water: it couples far better to the tissues in our ear than air does! The same is true for sound sources. Your post does not specify anything about the physics of the source, so it's impossible to make any statement about the expected differences. $\endgroup$
    – CuriousOne
    Commented Jan 6, 2015 at 22:00

1 Answer 1

Reset to default
2
+25
$\begingroup$

I don't see how this can be done, given the problem as-stated. What defines it as being 2 superimposed sounds, rather than just 1 sound, other than just an arbitrary definition? What stops me coming along and saying: "No, it's actually 4 superimposed sounds, or 27!"? If both sounds are coming from the same source then any shift in speed or frequency of each superimposed sound as it travels to each microphone will be the same, so I don't see how you can use this to separate them. Why do you think this high/low density material thing would make a difference?

If the 2 sounds were coming from different sources then they would take different times to reach the 2 microphones, so in that case I'm sure you could make some process or algorithm to separate them.

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.