Why is the speed of light in vacuum constant? [duplicate]

Question

Are there any proof of the speed of light in vacuum being constant? All I hear is that light in vacuum travels at a constant speed because that's an observation and that it fits in a coherent theory with all sorts of other detailed observations. Is there no like mathematical proof or something or is it just purely based on observations/measurements and why is the speed the same for all observers? Please, try to give simple and meticulous explanations since I'm not the best at physics and might have a hard time understanding what you mean otherwise.

Answer 1

Are there any proof of the speed of light being constant?

The speed of light is a physical quantity, so it is not subject of a mathematical proof but subject to measurement.

Interesting enough your demand to proof this particular physical statement is like asking for a proof of a mathematical axiom in the realm of mathematics:

If you hit an axiom in mathematics you can not proof it. An axiom is a statement independent from all other axioms and their derived mathematical truths. In this regard it is a starting point of mathematical reasoning, a fundamental, basic mathematical insight. You can accept a mathematical axiom as true or false and then go away with the more or less interesting or useful consequences of that choice.

The speed of light being constant is a physical principle.

A physical principle is believed to be true and (unlike a mathematical axiom) has to pass all known observations. It shares the fundamental, basic nature of a mathematical axiom in that it is used to derive other physical insights.

All i hear is that light travels at a constant speed because that's an observation

The famous Michelson-Morley interferometer experiment proved that speed of light did not depend on the orientation of the experiment's axes relative to the earth's movement through space. Which surprised the experimentators themselves quite a lot.

There were clever attempts to explain this observation by mechanical argumentations, e.g. the ether wind compressing the axis similiar to the Lorentz contraction. Hendrik Lorentz and the great mathematician Henri Poincaré came up with the correct formulas, but not with the correct physics.

The only satisfiying explanation came through Albert Einstein's special theory of relativity.

A and that it fits in a coherent theory with all sorts of other detailed observations.

Einstein's insight was that the experimental data only made sense if the speed of light is constant in all inertial systems, and that this leads to modifications of the prior known laws for time, length, mass, energy, and addition of velocities.

Is there no like mathematical proof or something or is it just purely based on observations/measurements

No that would require a identification of the observed physical reality with one of the many possible mathematical universes (and its set of mathematical axioms).

Maybe someone pulls this off in the future, but right now, we are not there.

But even then that identification must be rooted on observation.

why is the speed the same for all observers?

This is a as true believed physical truth, in accordance with all observations. A physical principle.

Einstein got the credit and not Lorentz or Poincaré, who certainly was the better mathematician, because of his formulations of physical principles.

The physical principles Einstein gave at that time was the

1. The special principle of relativity: physical laws should have the same form in all inertial systems (systems at constant speed).
2. The speed of light (in vacuum) is constant for every observer, independent of the movement of the light's sources.

From this he was the one to drop the ether hypothesis: Before it was assumed, even by Lorentz and Poincaré, that electromagnetical waves need a mechanical medium which does the vibrating and propagating like the media for water or gas waves. That hypothetical medium was the ether. The dropping of the ether hypothesis was a physical insight, not a mathematical one.

Answer 2

There is some mathematical justification, but it's not very user friendly. First you need to understand an operation in vector calculus known as curl, which is already a beast. Second, you need to be familiar with Maxwell's equations, where these curl operations are used. Third, you need to know the one-dimensional wave equation. Most of that stuff is on Wikipedia.

Once you have a basic familiarity with that stuff, you can sort of follow the respected physicist Maxwell's argument that an electrical field propagates at the constant speed of light. He started with the given equation, discovered by Michael Faraday (inventor of party balloons), that the curl of an electric field is equal to the negative change of magnetic field with respect to time. He then took the curl of both sides of the equation and after using a vector identity he saw that the equation was a one-dimensional wave equation. From this equation, he was able to see that the velocity of the equation equal to the the square root of a product of two numbers. These two numbers as I understand it are the magnetic permeability constant in a vacuum and an electric permittivity constant in a vacuum. And when you take the square root of the product of these two numbers, it's supposed to equal the speed of light. I never actually did the calculation myself. I just trust the argument. I'm not even familiar with the vector identity he used.

He may not even have used that exact argument, but that's what I found online, and it's probably the most user-friendly you're ever going to see.

Answer 3

The Wikipedia article on the speed of light states,

There are different ways to determine the value of $c$. One way is to measure the actual speed at which light waves propagate, which can be done in various astronomical and earth-based setups. However, it is also possible to determine $c$ from other physical laws where it appears, for example, by determining the values of the electromagnetic constants $\epsilon_0$ and $\mu_0$ and using their relation to $c$. Historically, the most accurate results have been obtained by separately determining the frequency and wavelength of a light beam, with their product equalling $c$.

It then lists a few methods, among them is the most precise method of interferometry (referred to in the above passage). Here, a (coherent) beam of light, with known frequency $\nu$, is split along its path (see image, taken from the speed of light link at the top) and generates a measurable destructive interference pattern which can be used to find the wavelength, $\lambda$. As stated in the above quote, the product $\lambda\nu$ returns the speed of light, $c$, with a fractional uncertainty of about $3\times10^{-9}$ (which corresponds to a variation of about $\pm0.001$ km/s).

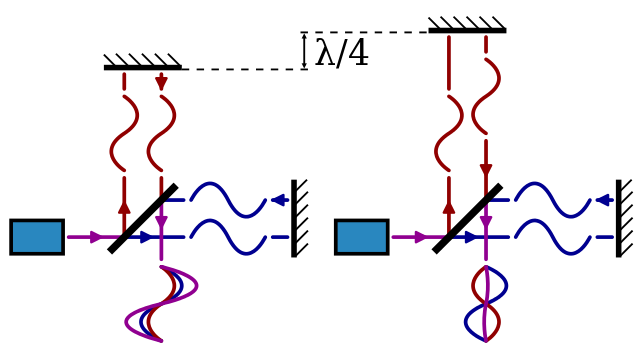
In the above image, the purple line denotes the combined light beam that is split in the North (red) and East (blue) directions at the slanted mirror. They bounce off mirrors (the thick black lines) and recombine. Either the two combine constructively into the original signal (on left) or they combine destructively into a zero signal (on right).

With regards to its constancy in reference frames, there are a collection of experiments that uphold the constancy; a list of these tests can be found at Wikipedia.

Answer 4

The idea is that if you and your friend are out in deep space far from anything and far from each other (but say moving away from each other), then each of you should be able to do physics experiments in your spare time and when you do you should be able write your laws of physics in a way that looks the same. You might place your origin in different places and so disagree on your coordinates, you might place your x-axis in different directions and so disagree on where something is on the x-axis, but you agree on what the laws themselves look like.

Some of those laws are Maxwell's equations (they might look scary, but you don't need to spend forever studying them, first just note that the things with zeros on them are constants, numbers with units):

$\nabla \cdot E =\rho /\epsilon_0$,

$\nabla \cdot B =0$,

$\nabla \times E =-\frac{\partial B}{\partial t}$,

$\nabla \times E =\mu_0(J+ \epsilon_0\frac{\partial E}{\partial t}).$

These laws, including the values of the constants $\epsilon_0$ and $\mu_0$ should be the same to both of you, and that is a matter of principle. A principle that so far has been affirmed by observation. Then we notice that these laws permit a travelling wave at speed $\sqrt{\frac{1}{\epsilon_0\mu_0}}$, and so you and your friend should both predict you will see waves that travel at a speed $\sqrt{\frac{1}{\epsilon_0\mu_0}}$. You both predict this even though you are moving relative to each other. We do measurements on the speed of light and notice that light moves at that speed, so maybe those waves are what light is. If so then we predict there are also other colors of light invisible to the human eye and when we do the experiments we detect them (UV, radio waves, gamma rays, microwaves). So we really think these waves, the ones described and predicted by the laws describing electric and magnetic fields are exactly what light is. So light moves at the same speed to everyone because the laws of electromagnetism are the same for everyone, including the values of the constants in those laws.

Answer 5

Because it makes the physical laws a lot simpler. There are many possible mathematical representations of reality that make identical predictions. Physically, they're all equally valid. One commonly used alternative just says $c=1$, for simplicity.

We could make models in which $c$ is not a constant, but the problem then is that a whole bunch of other things we believe should be constant are no longer constant either, and vary along with $c$. One well-known example is $$E=mc^2$$ which means that mass and/or energy would vary along with $c$.