Faraday's paradox

Question

I have studied that Faraday's law of induction and motional emf are two different lines of thinking but are essentially same.

But then, how can Faraday's paradox be explained by Faraday's law of induction? Particularly in paradoxes in which Faraday's law of induction predicts zero EMF but there is a non-zero . The wikipedia article is more confusing rather than enlightening.

I need an explanation regarding the article regarding this picture

EDIT : I wanted to know how is flux changing if faraday's law is valid .

Answer 1

My position is the same as Richard Feynman's and David Griffiths's and the wikipedia article's. It's simple: The law "change of flux = EMF" is not universally valid. The homopolar generator (that picture you copied) is a lovely counterexample.

(The law does always work for a loop of thin wire, but does not always work in other situations.)

Instead we should use the laws $\nabla\times E = -dB/dt$ and $F=qv\times B$, which are universally valid.

Answer 2

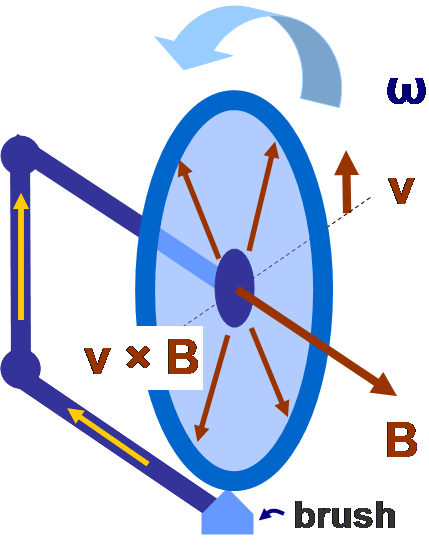
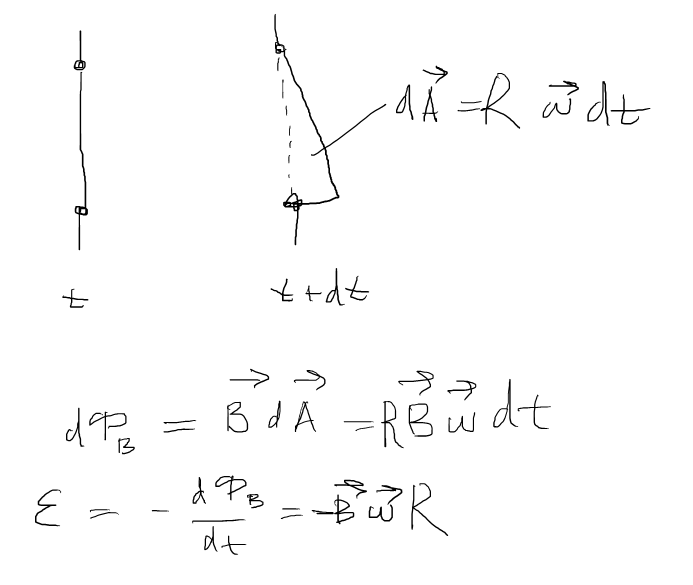
In Faraday's time, the paradox was not so much that there should be no EMF, but that there should be no flux if the magnet rotated with the disk. The resolution is that the magnetic field lines remain stationary even while the magnet rotates, so that the rate at which field lines cut across a line between the centre and the perimeter is not zero. It is really just for open wire segments that Faraday's law is derived, and we make use of it for closed loops in special situations. What is fundamental is the Lorentz force, $F = q \vec{v}\times \vec{B}$, or the existence of an electric field $\vec{E} = \vec{v}\times\vec{B}$ in the frame of reference in which the conductor is stationary. From these laws Faraday's law can be derived in certain situations.

Edit:

I suspect that you're after an answer that doesn't exist. Faraday's law is a mathematical statement that we try to make memorable by saying "the rate of change of flux through a loop is equal to the emf", which then loses a lot of what is actually going on.

Another person, Dims, gave the explanation of thinking of the disk as a limit of wheel spokes, and you were not satisfied with that answer. Instead, consider the case where the disk is continuous but the magnetic field only exists in say, the first quadrant. Now, pick a pizza-slice-shaped loop that is about to rotate across that quadrant. There will be an EMF around that loop according to Faraday's law. That is the statement that $\oint\!d\vec{r}\cdot \vec{E} \neq 0$ around the loop. This integral, I hope you can convince yourself, only depends on the radial parts of the path around the pizza slice, and not the circumferential part. This means that $\int_0^R\! dr \, E_r$ on one side of the loop is not equal in magnitude to that on the other side. I hope you can convince yourself that the EMF around the loop is solely due to the side that is entering the first quadrant, and not due to the part that is in zero field. Therefore, $\int_0^R\! dr \, E_r \neq 0$ as long as part of the slice remains outside of the first quadrant.

But that part of the slice contributes nothing to the integral, and might as well be lifted out of the plane of the disk, far away from the generator, in such a way that the flux is unchanged. You could imagine realizing this loop using electrical leads attached to your voltmeter. Therefore only the part of the loop that is in the magnetic field needs to be considered. That the result remains true for the entire disk follows from the principle of superposition.

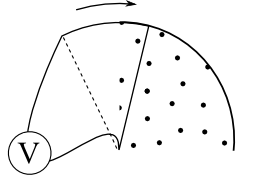
Edit 2: If you don't want to talk about electric fields, you can simply say that the EMF around the closed loop is the sum of the EMFs due to each segment of the loop.

Answer 3

You can regard disk as very dense wheel of spokes. Each spoke is a conductor and it moves in magnetic field. Hence it will induce current in it.

In continuous disk there are no spokes, but there is something similar: a resistance, hampering electrons to move in tangential direction.

UPDATE

Flux IS changing in spokes interpretation

Answer 4

FIX This answer needs heavy redevelopment, as I see now.

Just don't take all here as 100% truth :) 1. Need to rework currents part. 2. Need to rework integral part. 3. Generally unfinished.

Original text:

Lets take Maxwell law from which this is derived. I have dropped boldness, assume you see vectors below $\oint_{\partial \Sigma} E \cdot d\ell = - \int_{\Sigma} \frac{\partial B}{\partial t} \cdot ds$. Here path of integration is choosen such that it encloses surface $\Sigma$.

Clearly this form does not have anything to do with "Lorentz" force. But that does not have anything to do with "wires crossing something" too. You are right that wires do not cross anything. It is not wires crossing magnetic flux, but you are choosing the path of integration, along which you are accounting those differential forms.

Now the most interesting move is coming in. They say mostly in books that $$\int_{\Sigma} \frac{\partial B}{\partial t} \cdot ds = \frac{d}{dt} \int_{\Sigma} B\cdot ds$$ while not opening why is that satisfying. Lets investigate this equation more closely.

Right part coming from Faraday is, considering advective derivative $$\frac{d}{dt} \int_{\Sigma} B\cdot ds = \int_{\Sigma} \frac{\partial B}{\partial t} \cdot ds + \vec{v}\cdot \nabla \int_{\Sigma} B\cdot ds$$

Now you just need to compute gradient of B. Doing that is not easy task. You need always remember that metals are not so easy to build magnetic field, so that you will have to carefully treat conductive layer. As you already should know, external $B$ field does not comes inside your metal disk, so that there is always gradient of $B$.

Easy way is to go with Faraday, assuming that there is only wires, because they localize current.

Conclusions: magnetic flux is meaninless unless your current is localized. Faraday's law is meaninless outside of wires.

Answer 5

Two circuits one magnetic field

The electric circuit can be seen as being made up by two sections of wire.

Section A is the stationary circuit being the instrument and the wires to the stationary contact points at the center and the peripheral of the disk.

Section B is the circuit on the disk between the contact points.

At all times when there is a relative motion between the disk and the magnet a voltage Ub is induced in section B.

At all times when there is a relative motion between the magnet and section A a voltage Ua is induced in section A.

The trick is to observe that A+B makes up a closed loop but the motion of the conducting parts relative to the magnet differ.

Actually only voltages Ua and Ub has to be considered as the rest of the loop outside the disk has two arms with equal voltage canceling each other out. (Sum of flux entering and exiting the area is zero)

Voltage Ua reflects magnetic fields lines harvested when the magnet rotates.

Voltage Ub reflects magnetic field lines harvested when the magnet rotates relative to the disk.

The closed loop voltage U is Ua - Ub.

If magnet rotating and disk is stationary Ua>0, Ub>0 and they cancel out making U = 0.

If magnet stationary and disk rotates Ub>0, Ua=0 making U = -Ub

If magnet rotates with the disk Ub=0, Ua>0 making U = Ua