When is the principle of stationary action not the principle of least action?

Question

I've only had a very brief introduction to Lagrangian mechanics. In a physics course I took last year, we briefly covered the principle of stationary action --- we looked at it, derived some equations of motion with it, and moved on.

While the lecturer often referred to it as the principle of least action, he always reminded us that it wasn't actually least action, but stationary action --- a minimum, maximum, or point of inflexion, rather than just a minimum. He never, however, gave an example of a system where we didn't seek the least action.

Why is it, then, the principle of stationary action, instead of least action? What is an example of a system which we would seek a maximum instead of a minimum?

Answer 1

If we solve equations of motion for a particle with mass $m=1$ in some potential, e.g. $U=x^4-4x^3+4.5x^2$, fixing two points like $x(0)=0$ and $x(1)=2.651$, we'll get infinite number of solutions, here're some of them:

They differ by initial velocity. Now each of them satisfies equations of motion, but only one makes the action minimal, here's how $S$ depends on $v_0$ for such paths in this example:

As all they satisfy equations of motion, they make the action stationary, and it'd be wrong to just throw them away because they don't minimize the action.

Answer 2

In optics, you can take the example of a concave mirror : the optical path chosen by the light to join two fixed points A and B is a maximum.

Answer 3

I think, usually we don't seek minimum or maximum or saddle point action. We solve the Lagrangian for the first-order derivative of the variation of the path with respect to time and set it equal to zero because that is the condition for the actual path. The principle of stationary action states that the physical system follows a path for which the action is stationary (not necessarily minimal or maximal) under small variations of the path. The action is defined as the integral of the Lagrangian over time. The principle of least action is a special case of the principle of stationary action when the action is minimal for the true path of the system. However, this is not always true, as some systems may have multiple paths with the same action, or paths that are saddle points or maxima of the action. For example, in optics, light rays can follow paths that are not minimal in length, but are stationary under small variations. This can happen when light reflects from a curved mirror or refracts through a medium of negative refractive index. But it comes ultimately down to the physical configuration of those systems.

Historically principle of stationary action was first conceptualized as the principle of least action because most simple physical phenomena we experience in nature are ones that minimise the action, it was when scientists and philosophers began to divert attention to less frequent, complex or more artificial phenomena that they began to consider perhaps least action after all is a misnomer when and if you include all cases. And with quantum mechanics and relativistic physics principle of stationary action probably became the more appropriate term.

Here are two relevant posts:

1. lagrangian formalism - Confusion regarding the principle of least action in Landau & Lifshitz "The Classical Theory of Fields" - Physics Stack Exchange
2. classical mechanics - Is Action Always "Locally" Least? - Physics Stack Exchange

Answer 4

In this answer I will explain for which classes of cases the true trajectory corresponds to a maximum of Hamilton's action, after going over some preliminaries.

Depending on the circumstances it can be a minimum or a maximum. What counts is that which those two opposites have in common: as you sweep out variation the true trajectory is at a point in variation space such that the derivative of Hamilton's action is zero.

(Fermat's stationary time has an analogous property: with reflection against a concave surface: when the surface is concave enough the true trajectory of the light is a maximum of the Fermat time. Discussion in an answer by me: Fermat time)

In the case of Hamilton's action: whether the point (in variation space) where the derivative of Hamilton's action is zero happens to be a minimum or a maximum is determined by the form of the expression for the potential energy.

(Anyway, minimum or maximum is immaterial; it doesn't play any part. What counts is where in variation space the derivative of Hamilton's action wrt variation is zero.)

I start with using the simplest possible case as example. Further down I will discuss how the reasoning generalizes to all cases.

Example:
An object is thrown vertically upward. The object ascends decelerated by gravity, the object reaches its highest point, and from there is accelerated back down.

For simplicity: let the gravitational acceleration be 2 $m/s^2$, that gives the simplest expression for the motion.

Let the starting time be $t=-1$, and let the end time be $t=1$, let the initial and end height be zero height.

As we know: with a potential like that (potential increases linear with height) the resulting trajectory follows a parabola. The function that describes that parabola:

$$ f(t) = -(t+1)(t-1) = -t^2 + 1 \tag{1} $$

In this example sweeping out variation is by setting up a multiplication factor that modifies the height of the parabola. A variational parameter $p_v$ is implemented, in a form such that when $p_v$ is zero there is zero variation:

$$ f(t, p_v) = (1 + p_v)(-t^2 + 1) \tag{2} $$

In the image below:
Black curve: trajectory of the object
red curve: kinetic energy
green curve: minus potential energy

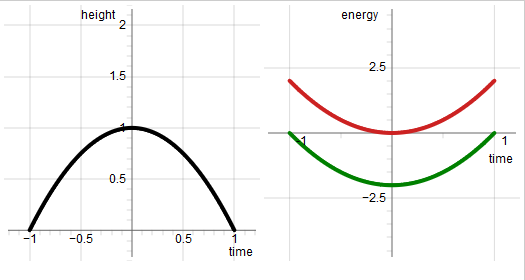
Diagram 1

In the diagram the curve that represents the potential energy (as a function of time) is mirrored in the horizontal axis. Mirroring the graph for the potential energy highlights a property of the true trajectory: at every point along the trajectory the rate of change of kinetic energy is equal to the rate of change of potential energy.

In the diagram below the sub-panel on the right shows how the integral of the red/green curve responds to change of the variational parameter $p_v$

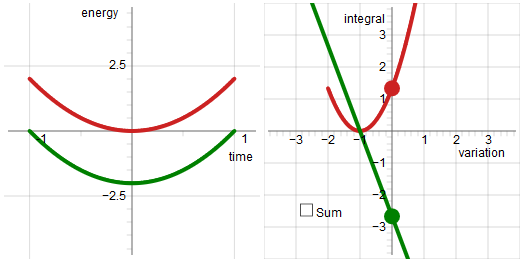
Diagram 2

In diagram 2: In the sub-panel on the right:
The red curve is a plot of the value of the integral of the kinetic energy as a function of the variational parameter $p_v$.
The green curve is a plot of the value of the integral of the potential energy as a function of the variational paramter $p_v$.

When you double the height of the trial trajectory then you quadruple the value of the integral of the kinetic energy; doubling the height doubles the corresponding velocity, and the kinetic energy is proportional to the square of the velocity. That is: the response of the integral of the kinetic energy to variation is proportional to the square of the variational parameter.

In the case depicted in the diagram the potential energy is a linear function of the height, therefore: the response of the integral of the potential energy to variation is linear.

The diagram below is an animated GIF. The value in the slider is the value of the variational parameter $p_v$

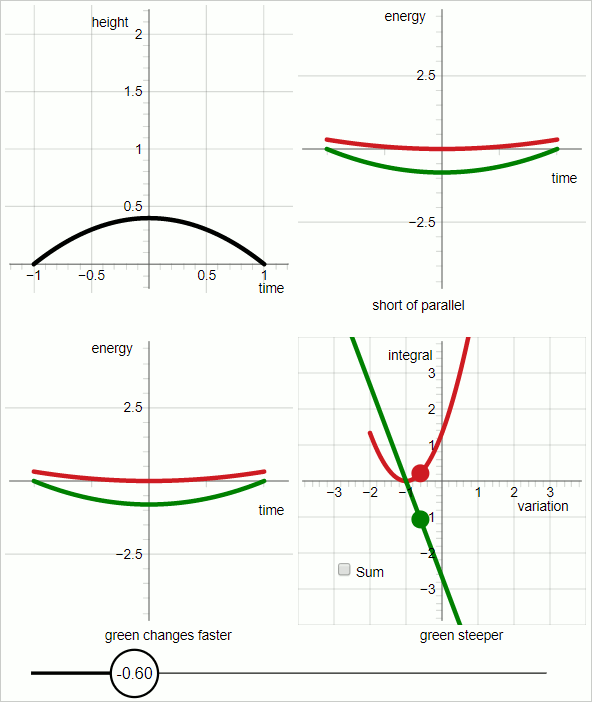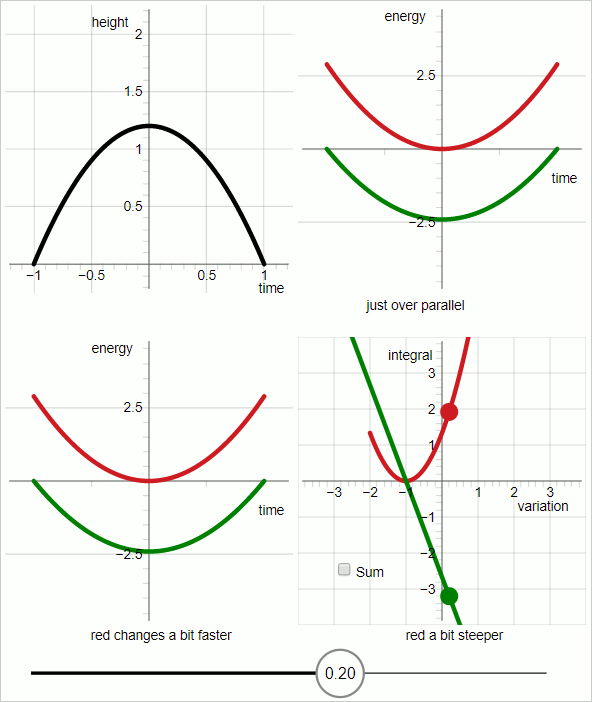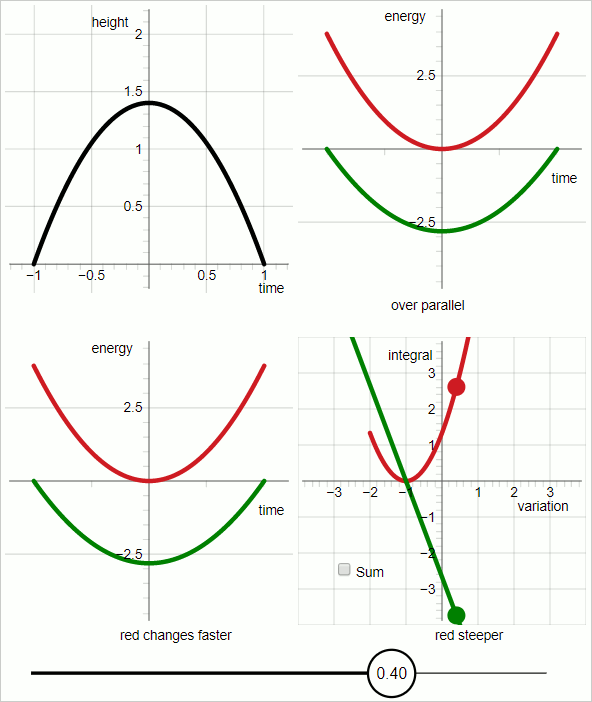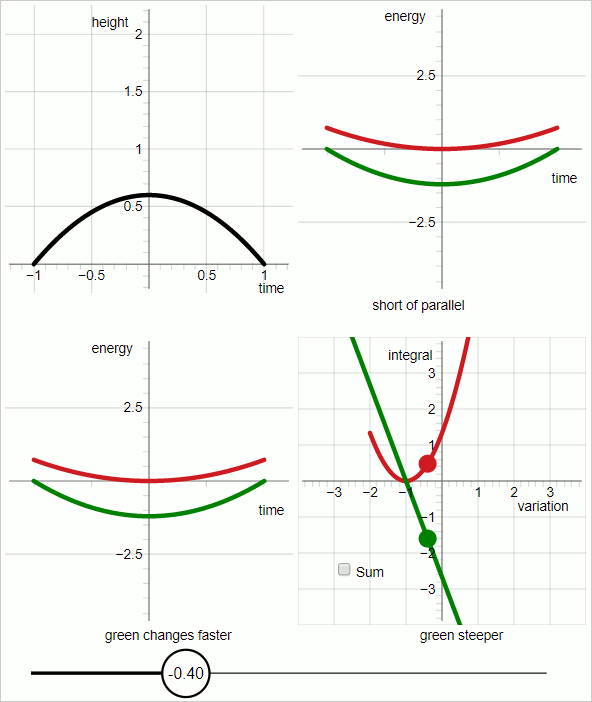
Diagram 3

The crucial property is displayed in the lower-right sub-panel:
In the lower-right sub-panel: the response to sweeping out variation: at the point in variation space where the trial trajectory coincides with the true trajectory the slope of the red curve matches the slope of the green curve.

In the lower-right sub-panel: the slope of the red/green curve is the rate of change with respect to variation. Now combine the red curve and the green curve: $\int E_k dt + (-\int E_p dt) = \int(E_k - E_P)dt$
At the point in variation space where the trial trajectory coincides with the true trajectory the derivative (wrt variation) of that combined quantity is zero.

The way that the potential energy responds to variation sweep follows from the form of the potential energy.

I will first discuss a potential that is proportional to the cube of the position coordinate, and then I will discuss a quadratic potential.

Cubic potential
With a cubic potential: when you double the height of the trial trajectory the integral of the potential energy comes out 8 times larger. That is, with a cubic potential the response of (the integral of) the potential energy to sweeping out variation is larger than the response of (the integral of) the kinetic energy.

So: with a cubic potential we get the inverse of what happens in the case of a linear potential. With a cubic potential the point in variation space where the derivative (wrt variation) of the quantity $\int(E_k - E_p)dt$ is zero will be a maximum of $\int(E_k - E_p)dt$.

Summerizing what has been discussed so far:

While the expression for the kinetic energy is always of the same form: proportional to the square of the (generalized) velocity, the potential energy can be any function of the position coordinate.

Using $x$ as position coordinate: the potential energy can be proportional to $\ldots, \ x^{-3}, \ x^{-2}, \ x^{-1}, \ x, \ x^2,\ x^3, \ \ldots $

If the power to which the coordinate is raised is lower than quadratic: the point in variation space where the derivative of $(E_k - E_P)$ wrt variation is zero will be a minimum.

If the power to which the coordinate is raised is higher than quadratic: the point in variation space where the derivative of $(E_k - E_P)$ wrt variation is zero will be a maximum.

So:
As we go through classes of cases, with the expressions for potential energy along the sequence $\ldots, \ x^{-3}, \ x^{-2}, \ x^{-1}, \ x, \ x^2,\ x^3, \ \ldots $, there is an inversion from minimum to maximum that occurs at the case $x^2$.

Noteworthy:
A crucial factor in this comparison of response to variation is that differentiation is a linear operator. Potential energy is a function of $x$ and kinetic energy is a function of $\tfrac{dx}{dt}$, but for the response of $(E_k - E_P)$ to variation: the variation just propagates through the differentiation: the only factor making a difference is the power to which the coordinate is raised.

The case where the potential increases in proportion to the square of the displacement is particularly interesting. The quadratic case is at the cusp of the inversion, the inversion from minimum to maximum.

As we know: when the potential increases quadratic with the displacement the resulting motion is harmonic oscillation. As we know, in the idealized case harmonic oscillation has the following property: the period is independent of the amplitude.

When you pump energy into the oscillating system the amplitude of the oscillation increases, but the period remains the same. The velocity and acceleration (as a function of time) increase in equal proportion to the increase of amplitude.

Now take the case of harmonic oscillation and evaluate the integral $\int (E_k - E_p)dt$ for half a period of the oscillation (or any integer multiple of half a period). To sweep out variation: increase/decrease the amplitude.

Let the position as a function of time be given by $f(t)=cos(t)$
then:
kinetic energy is proportional to $sin^2(t)$
potential energy is proportional to $cos^2(t)$

It follows: when you double the amplitude both the integral of kinetic energy and the integral of the potential energy double. It follows that the respective derivatives wrt variation will be the same.

Hence: In the case of a quadratic potential: for any amplitude the derivative of $\int (E_k - E_p)dt$ wrt variation will be the same: zero.

That gives us a class of trajectories that all have the same value for the derivative of $\int (E_k - E_p)dt$: zero.

So: In the case of harmonic oscillation evaluating the quantity $\int (E_k - E_p)dt$ leaves the problem underdetermined. In order to narrow down to a single trajectory an initial condition must be provided.

Incidentally, recognition that harmonic oscillation is a special case is not novel. Quote: E. F. Taylor and Chris G. Gray, 2007, When action is not least

The harmonic oscillator will turn out to be the only exception to many of the rules for the action.

Generalization

For the purpose of exposition I generated the variation by setting up a multiplication factor, madyfying the amplitude of the true trajectory. That is not a dependency; the validity of the reasoning generalizes to any form of implementing the variation.

The variation is exclusively variation of the position coordinate. That is: taking the derivative wrt variation boils down to taking the derivative wrt position coordinate.

That is to say:

If $\frac{d}{d(p_v)} \int(E_k - E_p)dt = 0$

Then: $\frac{d}{dx} \int(E_k - E_p)dt = 0$

The reasoning is not dependent on declaring the integral $\int (E_k - E_p)dt$ over any particular range. You can divide the trajectory into subsections, obtain a general expression for the integral for each subsection, and then concatenate the subsections again. There is no lower limit to this subdivision in subsections. The same reasoning remains valid all the way down to infinitesimally short subsections.

The validity of the Euler-Lagrange equation hinges on the subdivision into infinitesimally short subsections.

For Hamilton's stationary action it works out as follows:

If: $\frac{d}{dx} \int(E_k - E_p)dt = 0$

Then $\frac{d(-E_p)}{dx} - \frac{d(E_k)}{dx} = 0$

Note that:
$\frac{dE_k}{dx} = \frac{d(\tfrac{1}{2}mv^2)}{dx} = \tfrac{1}{2}m\left( 2v\frac{dv}{dx} \right) = m\frac{dx}{dt}\frac{dv}{dx} = m\frac{dv}{dt} = ma$

The following expression also evaluates to $ma$:
$\frac{d}{dt} \frac{dE_k}{dv} = \frac{d}{dt}mv = ma$

That demonstrates:
$\frac{d}{dt} \frac{d(\tfrac{1}{2}mv^2)}{dv} = \frac{d(\tfrac{1}{2}mv^2)}{dx}$

(The images are screenshots of diagrams that are available on my own website. A link to my website is available on my stackexchange profile page.)