5
$\begingroup$

Consider two bodies A(black) and B(red) having equal mass. A is moving at a constant speed towards B, which is stationary. At certain point of time, they collide elastically, $\therefore u_{A}=v_{B}$ and $v_{A}=u_{B}$ ($u$ = initial speed; $v$ = final speed) enter image description here

At the instant when B starts to move, $a=\frac{v-u}{t}=\frac{4-0}{0}=\frac{4}{0}$. But how can $a=\frac{4}{0}$ ? Is this logic correct?

Also,

Newton's First Law

An object at rest stays at rest and an object in motion stays in motion with the same speed and in the same direction unless acted upon by an unbalanced force.

Newton's law states that force is needed to change the state of rest or motion of a body. Does a force act on B? If so, then what is the magnitude of this momentary force that sets B in motion?

EDIT

I took $t=0$ while finding $a$ because I considered that velocity of B instantaneously changed from 0 to 4.

EDIT - 2

Now, as @bobie said, $E_{k}$ transfer from A to B requires time, which he demonstrated with an example. He used steel balls and calculated the speed with which $E_{k}$ propagated, i.e., the speed of sound in steel. We know that for body B to move, vibrations caused by A must reach the other end of B. But what if A and B are just single particles? Wouldn't then $E_{k}$ travel from particle A to B in an instant, as there are no intermediate particles? Would it still require some time for $E_{k}$ to propagate to the other end of B?

Improve this question
$\endgroup$
1
  • $\begingroup$ Comments are not for extended discussion; this conversation has been moved to chat. $\endgroup$
    – Manishearth
    Commented Oct 24, 2014 at 15:05

2 Answers 2

Reset to default
7
$\begingroup$

What acts here is called impulse

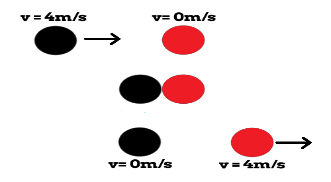
Suppose balls A, B are made of stainless steel and (m = 0.1 Kg r = 0.03 m) A has v = 4 m/s, B is at rest (v = 0, p = 0) they collide in 1-D. Since the mass is the same, A will stop dead and give B all its velocity and momentum p = (v = 4 * m = 0.1 Kg) = 0.4 Kg m/s: $$J = [F . t] = \Delta p = 0.1*4 -[0] = 0.4 Kg*m/s$$ If you know exactly of what steel the balls are made you can calculate the time of the collision and consequently the impulse of the force: kinetic energy travels in steel at the speed of sound $\approx 6000m/s$ (5800 -6100), let's say 6000.

Therefore the collision, at a rough estimate, will last d (= 2r = 2* 0.03 =) 0.06/ v = 6000 = 0.00001 sec, (or any value you like, since the the value is only indicative, and the force, as you'll see below is nominal). You can find out the force exerted $$F * \frac{1}{10^5} = [\Delta p] = 0.4 \rightarrow F \approx 4,000N$$

This peculiar description depends on the fact that the definition of 'Force' was modelled upon gravity, which is a force with constant acceleration and it was difficult to deal with other forces that give just velocity (and consequently momentum). You can find a thorough analysis here of the historical reasons for the newtonian definition of force, KE and work and a conceptual analysis of impulse here

If we consider a perfectly elastic collision, wouldn't the collision last for 0 seconds? meaning $a$ will be Undefined, and so will the $F$(force). – The Pragmatick

No, the collision lasts for the time KE takes to reach the other end of the ball. If you want an intuitive explanation for this just think KE cannot push, only pull. It is like a locomotive getting to the head of a train. A more rational explanation is that the mass at the far end cannot move until it gets its fair share of KE

But what if A and B are just single particles? Wouldn't then Ek travel from particle A to B in an instant, as there are no intermediate particles? Would it still require some time for Ek to propagate to the other end of B?

Also particles have a diameter. An instant is not $0$ time, as bright magus has showed in a comment. No time = no change. But time is irrelevant also here, as no force is acting, else it would be insanely great.

".. the concept of impulse which, to me, seems like a handwaving for explaining forces that appear and disappear for whatever reason." - luk32

Yes, I avoided to mention that explicitly, because it may rise some highbrows: the concept of impulse is an awkward attempt to describe mathematically the exchange of KE and momentum. It is just a fig leaf, a ruse, an artifice to fill in a gap in newtonian physics. A suggestive, subliminal message is shrewdly sent to the student adding to 'impulse' the tag 'of a force', trying to suggest that it is a sort of a force, but it has not the units.

In practice it is actually considered an instantaneous force, operating in this way an arbitrary idealization of solid bodies: the term 'instantaneous' itself is not scientific, as an 'instant' is not defined: in every-day speech it's just a short time, in various disciplines it can have [different values , see for example here: "...instantaneous force is for us the one that is destroyed in less than 30 seconds..."

Improve this answer
$\endgroup$
8
  • $\begingroup$ Thanks for your answer. If we consider a perfectly elastic collision, wouldn't the collision last for 0s? meaning $a$ will be Undefined, and so will the $F$(force). $\endgroup$
    – user49111
    Commented Oct 24, 2014 at 10:03
  • 4
    $\begingroup$ @imakesmalltalk, no, the collision lasts for the time KE takes to reach the other end of the ball. If you want an intuitive explanation for this just think KE cannot push, only pull. It is like a locomotive getting to the head of a train. A more rational explanation is that the mass at the far end cannot move until it gets its fair share of KE. $\endgroup$
    – bobie
    Commented Oct 24, 2014 at 10:06
  • 3
    $\begingroup$ I don't think you can talk about force in such context. There is a transfer of momentum. If you say there was force of 80kN then why the ball didn't shoot off? There must have been another force that cancelled it out during the time of collision. I don't think the mechanism of transfer of momentum is explained by Newtonian physics. Not 100% sure, though. Except of course, for the concept of impulse which, to me, seems like a handwaving for explaining forces that appear and disappear for whatever reason. $\endgroup$
    – luk32
    Commented Oct 24, 2014 at 13:27
  • 4
    $\begingroup$ @luk32 , ".. the concept of impulse which, to me, seems like a handwaving for explaining forces that appear and disappear for whatever reason." I explained that succintly in the post as it is OT there, if you read the links you realize that force is just nominal, it is just a ruse, an artifice to fill in a gap in newtonian physics, to justify with an ad hoc math, a procedure. What is really used is momentum; but OP asked for that and had a right to know $\endgroup$
    – bobie
    Commented Oct 24, 2014 at 13:50
  • 2
    $\begingroup$ @bobie Maybe if I find time later. I honestly wanted to reply to you, that I'd like to get a lecture from you =). Not necessarily that I would agree, but definitely it would be very interesting. I really like to be taught about concepts mathematical concepts, their realization and bounduaries of physical models. $\endgroup$
    – luk32
    Commented Oct 25, 2014 at 7:21
1
$\begingroup$

Sure. An elastic collision is merely an idealized process which never occurs in nature. On the microscopic level, the two bodies are made up out of atoms. The electron shells of these atoms contain electrons. Since negative charges repel each other, the two bodys will repel each other in a smooth fashion (an inverse square force is acting).

Improve this answer
$\endgroup$
4
  • 2
    $\begingroup$ I think you didn't get the question at all. The force I was talking about here was the momentary force exerted by A on B and vice-versa. $\endgroup$
    – user49111
    Commented Oct 24, 2014 at 9:05
  • $\begingroup$ @imakesmalltalk this answer is correct, if you need sth maybe you rephrase the question (see my comments on top as well) $\endgroup$
    – Nikos M.
    Commented Oct 24, 2014 at 9:23
  • 1
    $\begingroup$ I know perfect elastic collisions never really occur. But what I was asking is that in an ideal elastic collision, does second body's initial speed $u_{B}$ instantaneously become final speed $v_{B}$? And what is the magnitude of this instantaneous force? $\endgroup$
    – user49111
    Commented Oct 24, 2014 at 9:40
  • $\begingroup$ You are turning the definition of an elastic collision into a question. Forces never act isntantaneous. The magnitude of your "isntantaneous force" would be infinite. Talking about forces in elastic collisions is misleading. Also, as a general advice, study differential calculus. $\endgroup$
    – droemel
    Commented Oct 24, 2014 at 9:55

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.