This is a homework assignment, but not the physics part. I have to write a python script to simulate the size of a warhead required to deflect an asteroid (parameters inputted by user) that is on a collision course with earth. What is constant is that the missiles velocity is 11 km/s, the largest warhead created is rated at 50000 megatons, and the save distance from earth is 3 time the radius of earth from earth's center. We are also assuming half of the energy of the missile will be used to propel the asteroid while the other half will be used to deflect it.
How do I calculate the minimum distance from earth required to deflect it enough from earth to pass 3x earths radius? I also need to make some calculations with smaller missiles, but I figure once I know the needed equations I can figure this out.
I know how to program but it has been some time since I took physics and I went through my old book, test, and homework and cant seem to find anything about explosions that will help me.
Thanks in advance.
EDIT:
Here is a diagram given to help illustrate the problem I am to write the code for.
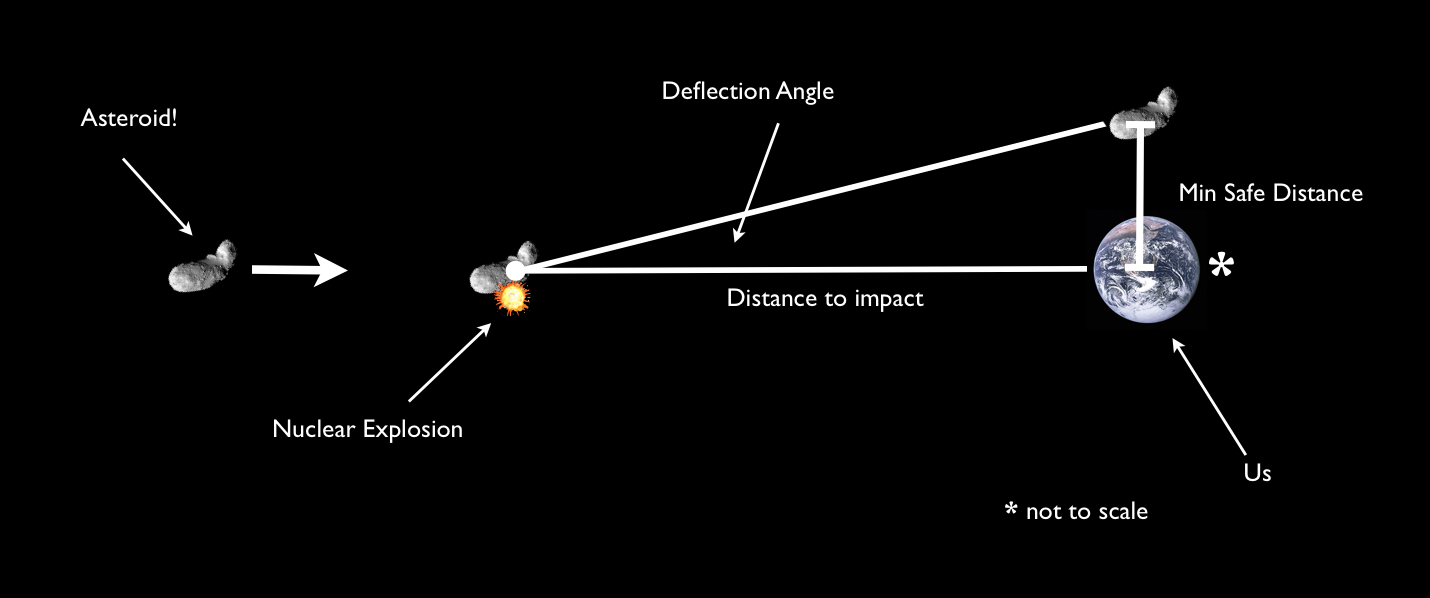
The inputs for the program are:
- the asteroids velocity,
- diameter (assume its a ball),
- type ( from type we estimate density and from there mass).
The outputs for the program are:
- the energy of the missile used for the calculation with the max being a 50000 megaton
- distance to impact
- missiles time of flight
- lead time required
I am pretty sure I can get time of flight and lead time and use different energies but the distance is what is throwing me off.
