1
$\begingroup$

As we go down the Earth, assuming it to be a uniform sphere abiding only to Newtonian physics, the gravitational acceleration imparted to an object by the Earth goes on decreasing, and decreases to 0 m/s^2 at the center.

Usually, we use the shell law to do these calculations. They are made easy and concise, but I would like to have an alternate approach.

I am going to do some really interesting calculations regarding how the acceleration due to gravity changes as we go down the Earth. Suppose we are at a depth 'h' in the Earth. If we choose to split the Earth for calculation purposes into two halves: one below us and the other part above, we can say that the part below would attract us in one direction and the other in the direction opposite to it(because their center of masses lie in one line)

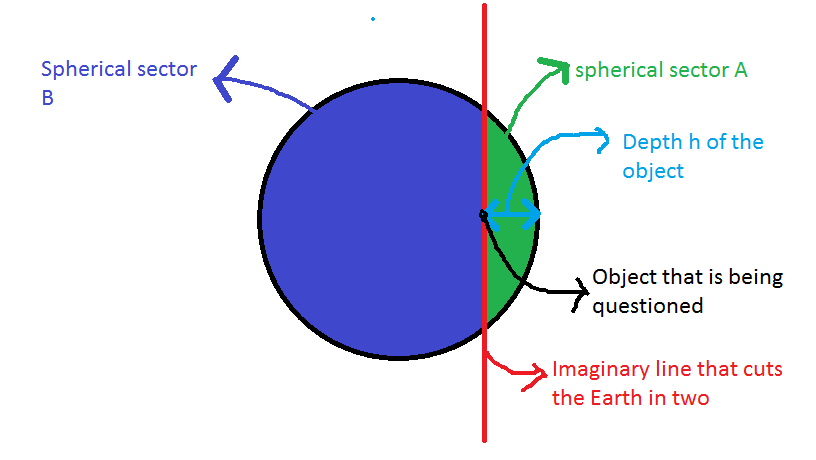
Idea pictorially represented

Now, if we figure out the respective attractions between these two sectors of the sphere and the body, we can determine the value of gravitational acceleration by subtracting one force by another and then dividing the resultant force by our mass to obtain the acceleration.

But I have a doubt. While I calculate the force, I take the the two sectors of the spheres to be point bodies at their respective center of masses and then calculate. Can this be done?

I have already used integration to find out the masses of these two sectors, but have yet to find the center of masses.

Can this assumption be made? If it cant, then how do you solve for gravity in objects with complex shapes?

Also, while finding the center of mass, i plan on cutting up the Earth into infinite infinitely thin plates, finding the mass of each plate and then defining the co-ordinates of each plate(with respect to a common origin), then integrate(co-ordinate*mass) with suitable limits.

Will this method yield an accurate result? I used a similar technique to find the masses, and they turned out to be accurate.

Improve this question
$\endgroup$
4
  • $\begingroup$ I think it is more a mathematical problem like this. But you can only approximate them as point particles in the far field limit. You will have to integrate the forces itself, i.e., the y-componentn of the 1/r^2. You see why people like the spherical shell approach? $\endgroup$
    – Bernhard
    Commented Sep 25, 2014 at 13:29
  • $\begingroup$ @Bernhard, I definitely see why people prefer shell method, but as a student in XI-th grade, I try to make my mini-eureka moments by doing stuff like this. $\endgroup$
    – Aayush Mahajan
    Commented Sep 25, 2014 at 13:42
  • $\begingroup$ There is no doubt that you could set up an integral given the geometry you have sketched. I'd suggest you use the symmetry provided the extending the light blue line of 'Depth h', and seeing how that goes. However, it will likely be painful and tedious. I'm of the opinion that (1) cranking through math can only result in errors, and as a corollary (2) those who learn to 'see' the easy math solution are those who get physics at a deeper level... $\endgroup$
    – Jon Custer
    Commented Sep 25, 2014 at 13:51
  • $\begingroup$ Your alternative method doesn't work. The reason is hidden in Newton's 'shell theorem': en.wikipedia.org/wiki/Shell_theorem $\endgroup$
    – Johannes
    Commented Sep 25, 2014 at 16:19

1 Answer 1

Reset to default
1
$\begingroup$

The point mass assumption is only good if you're sufficiently far away such that the angle formed between the center of mass and the farthest extent of the massive body is sufficiently small. (i.e. the level of error of using the approximation sin x = x is considered acceptable).

You can certainly divide the Earth into two pieces, but you'll have to integrate the effect of each piece.

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.