Particle acceleration at magnetized shocks by convective electric fields?

Question

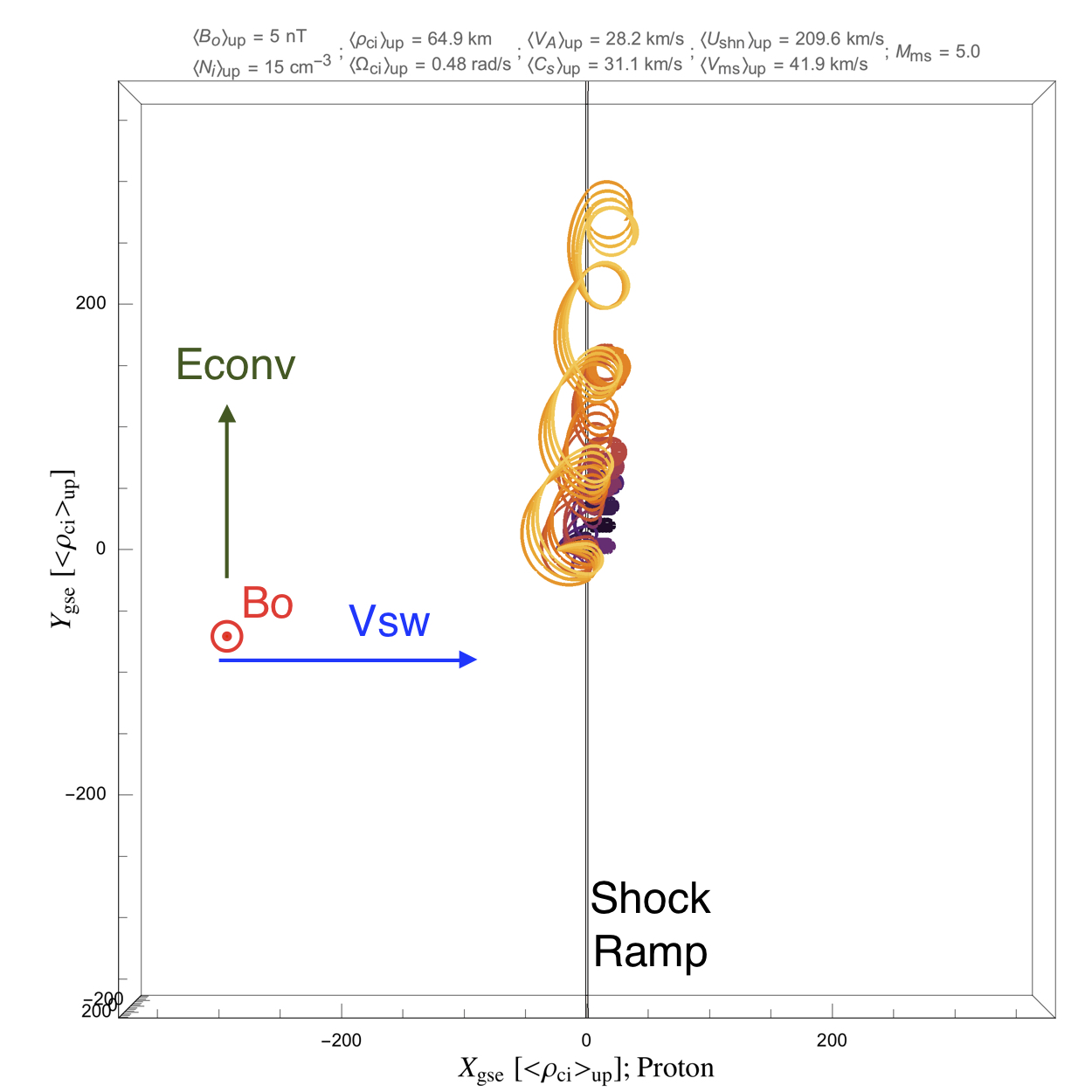
Let us assume we have a flow of charged particles in a quasi-neutral state (i.e., a plasma) convecting at some speed, $\mathbf{V}_{sw}$ = V$_{sw}$ $\hat{x}$, and the particles have species-dependent thermal speeds, V$_{Ts}$ $\ll$ V$_{sw}$ (for simplicity, just assume protons and electrons). Assume there is a magnetic field, $\mathbf{B}_{o}$ = B$_{o}$ $\hat{z}$, that is small enough that particles can be said to have effectively balistic trajectories (i.e., ignore the gyration initially and/or V$_{Ts}$ $\ll$ V$_{sw}$). Assume that this flow and magnetic field are entirely laminar and homogeneous everywhere initially and that the magnetic field moves with the flow at V$_{sw}$ (this latter part confuses me since sources move, not really the fields, but just bare with me).

Now what if these particles are incident on a magnetic field gradient, $\nabla$B, which is a vector parallel to $\hat{x}$ and |B| only increases in the $\hat{z}$ component. Assume the gradient is strong enough to reflect a small fraction of the incident particles. For now, let us just focus on protons and assume the reflection is specular. Also, let us work in the reference frame where the magnetic field gradient is at rest.

(1) Since the upstream flow and magnetic field are both homogeneous everywhere upstream of the magnetic field gradient, will any given reflected proton know that the magnetic field is moving?

The purpose of the question is to understand arguments that say once a proton is reflected, it will "see" an electric field, $\mathbf{E}_{conv}$ = -$\mathbf{V}_{sw}$ $\times$ $\mathbf{B}_{o}$. However, in my memory of Lorentz transformations, $\mathbf{v}$ $\equiv$ the speed of a reference frame relative to some other lab frame. The idea of a magnetic field moving in this manner bothers me, but not as much as the expression for $\mathbf{E}_{conv}$.

(2) Should not $\mathbf{E}_{conv}$ be expressed in terms of the reflected proton's instantaneous velocity relative to $\mathbf{B}_{o}$ (i.e., $\mathbf{v}$), where $\mathbf{B}_{o}$ is the magnetic field in the proverbial lab frame?

The arguments to which I refer suggest that particles gain energy from this frame-dependent electric field. This should only work if $\mathbf{v}$ $\parallel$ $\mathbf{E}_{conv}$. However, if $\mathbf{E}_{conv}$ = -$\mathbf{v}$ $\times$ $\mathbf{B}_{o}$ as my intuition suggests, then $\mathbf{v}$ $\cdot$ $\mathbf{E}_{conv}$ = 0, right?

(3) Am I wrong to be bothered by the concept of magnetic fields "moving" in the manner I described above, or am I missing something?

Answer 1

(1) Since the upstream flow and magnetic field are both homogeneous everywhere upstream of the magnetic field gradient, will any given reflected proton know that the magnetic field is moving?

This, unfortunately, displays how easily I can confuse myself about something that should be relatively trivial. As @CuriousOne noted, I managed to over complicate things and this led to my confusion.

The first thing to notice is that for the scenario where $\mathbf{V}_{sw} = V_{sw} \ \hat{\mathbf{x}}$ and $\mathbf{B}_{o} = B_{o} \ \hat{\mathbf{z}}$ are satisfied, there must exist an electric field that allows the plasma to flow across the magnetic field. Let's assume this is the Sun's rest frame. From the Lorentz transformation of the electric field (in the non-relativistic limit) we find that $\mathbf{E}_{conv} = -\mathbf{V}_{sw} \times \mathbf{B}_{o}$ (i.e., parallel to $+\hat{\mathbf{y}}$) in the Sun's rest frame. It is then easy to show that in this frame that $\mathbf{V}_{sw}$ is the ExB-drift speed given by: $$ \mathbf{V}_{sw} = \frac{ \mathbf{E}_{conv} \times \mathbf{B}_{o} }{ B_{o}^{2} } \tag{1} $$

In the plasma rest frame (i.e., where $\mathbf{V}_{sw} \rightarrow 0$), however, there is no electric field.

Thus, part of the answer is not that magnetic fields move, which is clearly wrong, rather that the sources move and the fields respond. When we think specifically about a shock moving through this medium, we need an additional transformation velocity. In the case of, say, Earth's bow shock, the shock rest frame is roughly defined by subtracting $\mathbf{V}_{sw}$ since the Earth is roughly at rest relative to the solar wind.

So a proton initially on a balistic trajectory toward the shock will, if reflected, suddenly "see" an electric field due to the frame transformation. Thus, the second part of the answer to my first question is just that the frame transformation results in the electric field and has nothing to do with moving magnetic fields.

(2) Should not $\mathbf{E}_{conv}$ be expressed in terms of the reflected proton's instantaneous velocity relative to $\mathbf{B}_{o}$ (i.e., \mathbf{v}), where $\mathbf{B}_{o}$ is the magnetic field in the proverbial lab frame?

Again, this shows a clear confusion on my part. The relevant electric field is expressed in the frame of reference where calculations are being performed. The relevant reference frame is the shock rest frame.

The particles can gain energy from $\mathbf{E}_{conv}$ in this frame because upon reflection from the moving magnetic gradient, the magnetic contribution to the Lorentz force will accelerate the particle along the $\hat{\mathbf{y}}$ direction, thus $\mathbf{v} \cdot \mathbf{E}_{conv} \neq 0$ in this frame. The particle can gain energy so long as it continues to "skip" along the upstream edge of the shock ramp.

I wrote a simple Mathematica routine to simulate this with numerous test particles with different initial trajectories (see image below). The particles that undergo multiple reflections gain more energy than those that are transmitted into the downstream after one (or zero) reflection(s), which is evidenced by the larger gyroradii of the particles with increasing distance along $+\hat{\mathbf{y}}$.

The above image is an example of something called shock drift acceleration (SDA), where shock-reflected particles gain energy while skipping along the upstream edge of the shock front from the convective electric field. This is not to be confused with diffusive shock acceleration (DSA) (or first order Fermi acceleration) whereby particles gain energy by reflecting off of merging magnetic mirrors. A nice animation of SDA can be found at https://svs.gsfc.nasa.gov/4513.

(3) Am I wrong to be bothered by the concept of magnetic fields "moving" in the manner I described above, or am I missing something?

As I have already stated, I was missing several things. Further, magnetic fields do not "move" (i.e., field lines do not move), sources move or change and the fields respond.