In case of banked roads without friction, there is an additional $mg \sin(\theta)$ which is unbalanced. Why isn't this taken into account because it is responsible for making the vehicle slide down the inclined plane?
$\begingroup$
$\endgroup$
2
-
1$\begingroup$ Can you elaborate on this? Are you talking about a stationary car or a moving car? If the latter, what speed is the car moving? $\endgroup$– John RennieCommented Sep 1, 2014 at 5:50
-
$\begingroup$ When describing a physical situation it is important to communicate clearly. Otherwise people will be left to guess. (This is too-often a fault in published papers, which makes me wonder if anyone read them prior to publication.) In addition to not specifying the motion of the car, you leave us guessing as to what $\theta$ is. Here it's not a big deal because it's clear from context, and a little work. Contributors here are volunteers; it's good form not to give them extra work in figuring out your meaning. On an exam, you'll get points off. $\endgroup$– garypCommented Sep 1, 2014 at 12:03
Add a comment
|
2 Answers
$\begingroup$
$\endgroup$
7
When you're in a vehicle turning on a road, you'll feel a centrifugal force on the vehicle. This will be in the horizontally outward direction. The centrifugal force and weight add up vectorially.
\begin{align} \vec{F_w} &= m\vec{g} \\ \vec{F_c} &= m |\vec{\omega}|^2 \vec{r} \\ \therefore \vec{F_{net}} &= \vec{F_w} + \vec{F_c} \end{align}
Now, it so happens that, if your velocity through the turn is just right, the forces line up such that the net force is perpendicular to the road, and this is what you get:
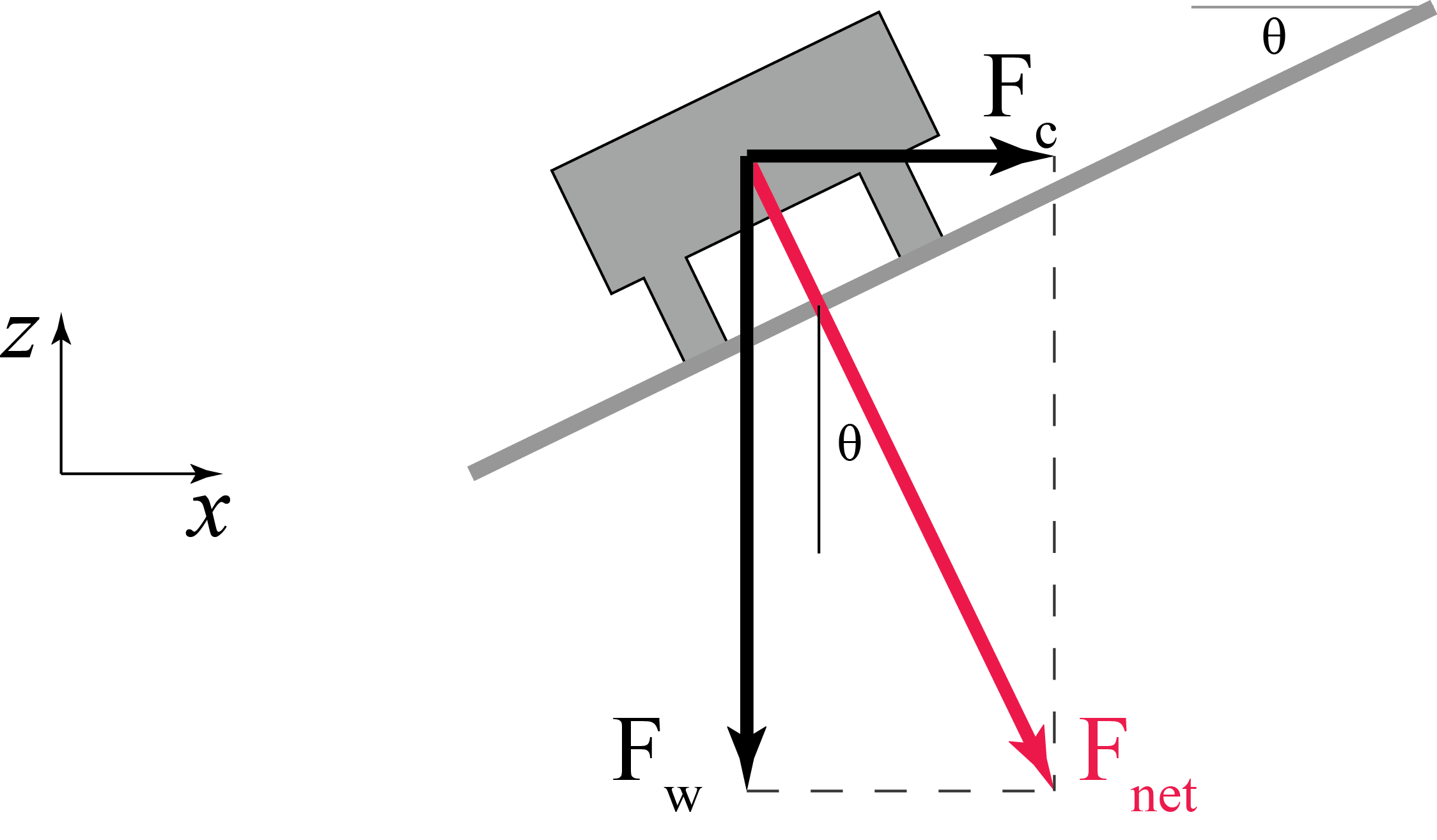
This would mean that the only force on the vehicle is pushing it into the road, so it doesn't slide down.
In this case,
\begin{align} \tan(\theta) &= \frac{|\vec{F_c}|}{|\vec{F_w}|} \\ \therefore \theta &= \tan^{-1} \left( \frac{\omega^2 r}{g}\right) \\ &= \tan^{-1} \left( \frac{v^2}{gr}\right) \end{align}
Where, $r$ is the radius of the turn $v$ is the velocity through the turn $\omega$ is the angular velocity through the turn
-
2$\begingroup$ Interestingly, a bobsled track has a "continuously varying" bank angle, and as the sled goes faster it "climbs" more as it attempts to maintain exactly the equilibrium described here. $\endgroup$– FlorisCommented Sep 1, 2014 at 6:27
-
$\begingroup$ Isn't there a reaction force exerted on the car by the road? $\endgroup$– DJohnMCommented Sep 1, 2014 at 6:27
-
$\begingroup$ @User58220, the reaction is equal and opposite to the net force, so it cancels it out. This is why the car doesn't sink into the road. The reaction force is always perpendicular to the surface, so it is irrelevant when you're thinking about the car sliding down the road. $\endgroup$– phoCommented Sep 1, 2014 at 22:09
-
$\begingroup$ So when the missing force is added, there is no net force on the car, and thus no acceleration in any direction? $\endgroup$– DJohnMCommented Sep 1, 2014 at 22:13
-
$\begingroup$ @User58220, The car is not an inertial reference frame, so no force does not entail no acceleration. $\endgroup$– phoCommented Sep 1, 2014 at 22:20
$\begingroup$
$\endgroup$
The normal analysis of this problem involves working in the frame of reference of the road; one where Newton's laws of motion apply, and there are no fictitious forces needed.
In this diagram:
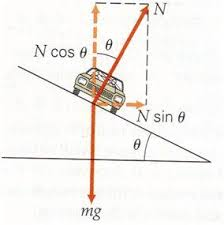
the two solid lines represent the only two real forces acting on the car: the force of gravity, $mg$, acting downward, and the reaction force of the road, $N$ , acting upward at right-angles to the road.
Since the car is not accelerating vertically, we have that, vertically:$$N\cos\theta=mg$$and, since there is enough horizontal Centripetal Force to keep the car moving in a circle $$N\sin\theta=\text{Centripetal Force}$$ The Centripetal Force is specified by the mass of the car, the velocity, and the radius of the curve.
You could chose to resolve all these forces along the road and at right angles to the road; the results would be messier, but not basically different; for forces along the road (down to the right positive)$$mg \sin\theta = (\text{Centripetal Force})\times \cos\theta$$ and for forces acting at right angles to the road (above the road positive)$$N-mg\cos\theta=(\text{Centripetal Force})\times \sin\theta$$
