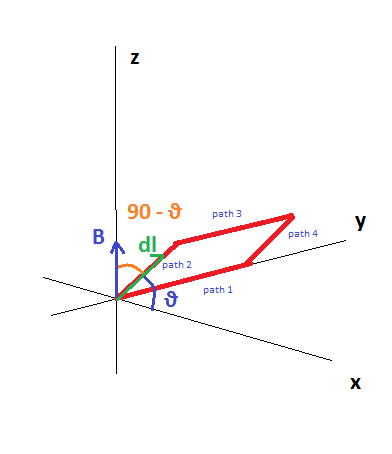
I was reading an answer about torque acting on a rectangular current carrying loop kept in a uniform magnitude field B. Force acting on each sides is $F_1$, $F_2$, $F_3$, $F_4$. It's written here : Force $F_2$, $F_4$ acting on these sides has same magnitude $F' = Blb \sin(90-\theta) = Blb \cos\theta$. And $F1$ and $F3$, have $F=IlB\sin90° = IlB$.
(where $l$=length; $b$=breadth) I understand $F_1$ and $F_3$. But I don't get how the magnitude of forces of $F_2$, $F_4$ become $Blb \cos\theta$ . Which law says that? Am i missing some knowledge?
If you need the diagram or the book text for more details , please ask for it.