A rectangular loop and a circular loop are moving out of a uniform magnetic field to a field-free region with a constant velocity V as shown in the figure. Explain in which loop do you expect the induced emf to be constant during the passage out of the field region. The magnetic field is normal to the loops.
Well if we take $B$ and $v$ as constants here then it is pretty easy, because all you need to do is calculate the change of the magnetic flux through the surface enclosed by either loops, and you know that the induced EMF ($\epsilon$) is given by: $$ \epsilon=-N\frac{d\Phi}{dt} $$ where $\Phi = \mathbf{B}\cdot \mathbf{A}$, since we started off with $B$ constant then, $$\frac{d\Phi}{dt} \propto \frac{dA}{dt} $$ In the case of the rectangular loop, the amount by which the surface changes as the loop moves out, is constant, so: $$\frac{dA_{\rm rect}}{dt} = constant \leftrightarrow \epsilon = constant$$ Whereas the change of magnetic flux in the ciruclar loop isn't constant since the surface of the circle doesn't change by equal amounts as the loop moves out: $$\frac{dA_{\rm circ}}{dt}\ne constant $$
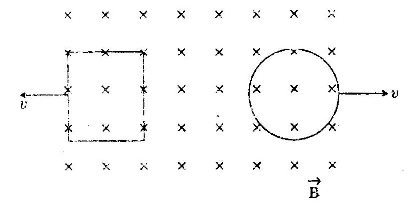
What @Phonon explained seems partially correct to me as it appears to me that the radius of the circle is infact constant and just like the rectangle,for an infinitesimal value dr=vdt.The rate of change of area of the loop with respect to time is infact not a constant value,but it appears to me that when a certain portion of the circle comes out of the field the part inside the field no longer remains a circle and infact becomes a segment of a circle so we can no longer use the simple formula of πr² to calculate the rate of change of area of the loop.What we should do instead is calculate the rate of change of area of the segment of a circle with a constant radius r.Now there are obviously two segments of a circle, namely major and minor segments.But what we are interested in is to calculate the area of the major segment.It turns out their is no direct formula to calculate the area of a major segment so what we can do is calculate the area of the minor segment that is outside the field and subtract it from the total area of the circle.The formula for area of a minor segment of a circle is
$$A = \frac{R^2}{2}(\theta - \sin\theta)$$
where R is the radius of the circle and θ is the angle made by the minor arc at the centre of the circle bounded by the respective radii thus forming a sector.Now the significant variable in this situation is θ because as the circle moves out of the field the value of θ changes continuously and it has no relation with the linear velocity v of the loop hence θ is the variable due to which the area of the segment is not a constant(and not 'r' as @Phonon suggested) and hence the rate of change of area of the circle is not a constant.Which means that the emf of the rectangular loop is infact constant whereas that of the circular loop is not. Another way we could have calculated the area of the segment is for the case when major segment would have already left the field and all we would be left with would have been a minor segment in which case we could have directly used the formula for the area of the minor segment. Either way would get the same result. This is my first answer so feel free to correct me! Thanks
