Describing a circular current loop as delta functions

Question

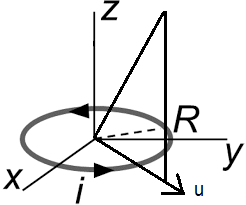
It would be really nice to see how Jackson got eqn 5.33 on his example problem for finding the vector potential of a circular current loop

$$ J_{\phi}=I\sin\theta'\delta(\cos\theta')\frac{\delta(r'-a)}{a} $$

for describing this geometry:

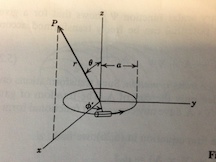
I've found a few 'explanations' googling, but I'm still confused on the origin of the $\sin\theta$

My best guess

$$ \int \vec{J}\cdot\hat{n} \; d^3x=\int \vec{I}\cdot d\vec{l} \\ \nabla_\phi \left ( \int \vec{J}\cdot\hat{n} \; d^3x=\int \vec{I}\cdot d\vec{l} \right) \\ \frac{1}{\sin\theta} \frac{\partial}{\partial\phi} \int \vec{J}\cdot\hat{n} \; r^2 dr d\cos\theta d\phi = \frac{1}{\sin\theta} \frac{\partial}{\partial\phi} \int I a d\phi \\ \frac{1}{\sin\theta} \int J_\phi r^2 dr d\cos\theta = \frac{1}{\sin\theta} I a \\ \frac{1}{\sin\theta} \int A \delta(\cos\theta) \delta(r-a) r^2 dr d\cos\theta =\frac{1}{\sin\theta} I a \\ Aa^2=Ia \\ A=\frac{I}{a}\\ \therefore J_\phi = I \delta(\cos\theta) \frac{\delta(r-a)}{a} $$

I know the $\sin\theta$ doesn't really matter.. because

$$ \int \sin^2\theta \delta(\theta-\pi/2)d\theta=1 $$

but I'd still like to know where it comes from.

EDIT
The explanation that "it doesn't matter, so lets include it" bothers me. There must be some place in the derivation he picks it up. What possible reason would there be for adding it in at the end?

The change of variables [ref] doesn't work because

$$ \delta(\cos\theta)=\frac{\delta(\theta-\pi/2)}{\sin\frac{\pi}{2}} $$ so you still end up with $$ \int \sin^2\theta \delta(\theta-\pi/2)d\theta $$

I also don't think that it comes out of the Jacobian. Because he later goes on to integrate over the solid angle

$$ \int J r^2 d\Omega dr = \int J r^2 \sin\theta d\theta d\phi $$ which would, again, give something of the form $\sin^2\theta$

EDIT
Jackson's next step (Eqn. 5.35)

$$ A_\phi(r,\theta)=\frac{\mu_0I}{4\pi a}\int r'^2dr'd\Omega' \frac{\sin\theta'\cos\phi'\delta(\cos \theta')\delta(r'-a)}{\left | \mathbf{x}-\mathbf{x'} \right |} \\ \; \\ \text{with } d\Omega' = d\cos\theta' d\phi = \sin\theta' d\theta' d\phi $$

I would agree that a $\sin \theta$ belongs in the formula if he only integrated over $d\theta$ not $\sin\theta d\theta$

Answer 1

See, you are required to find volume current density $J_\phi$. Though its name is volume current density, you know it is the current flowing per unit surface area. Now the subscript $\phi$ in $J_\phi$ denotes it is flowing in the $\hat{\phi}$ direction. Now in the spherical polar co-ordinate the infinitesimal length elements along the direction $\hat{r},\hat{\theta} \rm\ and\ \hat{\phi}$ are dr, $rd\theta$ and $r\sin\theta d\phi$ respectively. So $dr\times rd\theta$ is the area you are interested in.

Now looking at your figure I am interested in rewriting the current I.

$$I=I\int \delta(r-a) dr= I\int \delta(r-a) dr\ \delta\big(\cos\theta-cos(\pi/2)\big) d(cos\theta)$$ $$=I\int \delta(r-a) dr\ \delta\big(\cos\theta-0\big) d(cos\theta)$$ $$=I\int \delta(r-a) dr\ \delta\big(\cos\theta\big) d(cos\theta)$$ $$=I\int \delta(r-a) dr\ \delta\big(\cos\theta\big) \sin\theta d\theta$$ $$=I\int \delta(r-a)\delta\big(\cos\theta\big) \sin\theta dr\ d\theta$$ $$=I\int \frac{ \delta(r-a)\delta(\cos\theta) \sin\theta}{r} {dr\ r d\theta}$$

So you see your $$J_\phi = I\frac{ \delta(r-a)\delta(\cos\theta) \sin\theta}{r}$$

Answer 2

If you note that $$\delta(\cos\theta)=\frac{\delta(\theta-\pi/2)}{\sin\theta}$$ Then you can see that the sine terms actually cancel out.

Answer 3

EDITED ANSWER: The delta distribution $\delta(x)$ is not unique. It is invariant under transformations of the form $\delta(x) \to f(x)\delta(x)$ where $f(0) = 1$. This is because it is really a distribution and not a function. It is mathematically improper to talk about $\delta(x)$ instead of $\int \delta(x)dx$. Derivations of the term you're interested in will not be unique either. You can show that

$$ I = I\int \delta(r-a)dr = I\int \delta(r-a)\delta(\theta - \pi/2)drd\theta = I\int\frac{\delta(r-a)}{r}\delta(\theta - \pi/2)rdrd\theta. $$

From this expression, it is apparent that we can write the current as

$$ I = \int J_{\phi}rdrd\theta \implies J_\phi = I \frac{\delta(r-a)}{r}\delta(\theta - \pi/2). $$

This result leaves out the $sin(\theta)$ and replaces $a$ with $r$. It really makes no difference because distributions are mathematically represented by $(T, F)$ where $F$ is a set of continuous functions and $T$ is the map from $F \to \mathbb{R}$. While it is common for physicists to represent distributions as just the mapping (i.e., $T = (T,F)$), this is somewhat false and leads to the non-unique representation of quantities like $\delta(x)$.

Answer 4

the correct answer can be easily obtained when you use the definition of \begin{equation} \overrightarrow{J}=\rho \overrightarrow{v} \end{equation}

with $\rho=q\delta(\overrightarrow{x}-\overrightarrow{x'})$, you will have:

\begin{equation} \overrightarrow{J}=q \overrightarrow{v} \delta(\overrightarrow{x}-\overrightarrow{x'}) \end{equation}

a small moving charge $dq$ yields to a small current density $d\overrightarrow{J}$, so

\begin{equation} d\overrightarrow{J}=dq \overrightarrow{v} \delta(\overrightarrow{x}-\overrightarrow{x'}) \end{equation}

the charge will move along the distance $d\overrightarrow{l}$:

\begin{equation} d\overrightarrow{J}=dq {{d\overrightarrow{l}}\over dt} \delta(\overrightarrow{x}-\overrightarrow{x'}) \end{equation}

but one defines $I={dq\over dt}$

\begin{equation} d\overrightarrow{J}= I {d\overrightarrow{l}}\delta(\overrightarrow{x}-\overrightarrow{x'}) \end{equation}

\begin{equation} \overrightarrow{J}= \int I \hspace{0.2cm}{d\overrightarrow{l}}\hspace{0.2cm}\delta(\overrightarrow{x}-\overrightarrow{x'}) \end{equation}

now you must write ${d\overrightarrow{l}}$ according the problem as well as the Dirac's delta function. For example, if you have a circular current loop with radius a in a x-y plane and with its center and the origin, you can write ${d\overrightarrow{l}}=a\hspace{0.1cm}d\phi \hat{\phi}$ and using cylindric coordinates for Dirac's delta:

\begin{equation} \overrightarrow{J}= \int I \hspace{0.2cm} a\hspace{0.1cm}d\phi \hat{\phi}\hspace{0.2cm}{\delta(r-a)\over r} \delta(\phi) \delta(z) \end{equation}

\begin{equation} \overrightarrow{J}= I \hspace{0.2cm} \hspace{0.1cm} \hat{\phi}\hspace{0.2cm}\delta(r-a) \delta(z) \end{equation}

I hope that can help you!

Answer 5

The following is a systematic way of obtaining the desired description for the current in the wire.

In order to represent the current, we must make use of the azimuthal symmetry to reduce the problem to one involving two coordinates $(u,z)$, check the figure below.

Where $u$ represents a point in the x-y plane. This is related to the spherical coordinates by $$u=r\sin\theta$$ $$z=r\cos\theta$$ Notice that rotating $u$ about the z-axis would give us the original problem. The current wrt u and z is given by $$\vec{J}=i\delta(u-R)\delta(z)\hat{\phi},$$ where $i$ is the current flowing in the wire of radius $R$ and $\hat{\phi}$ is the unit vector corresponding tangential to the ring. Now using the transformation law for delta functions, we obtain $$\vec{J}=i\dfrac{\delta(r-R)\delta(\theta-\frac{\pi}{2})}{|J|}\hat{\phi},$$ where $|J|$ is the Jacobian of the transformation. This is given by $$|J|=\vert det\begin{pmatrix}\dfrac{\partial u}{\partial r} & \dfrac{\partial z}{\partial r}\\ \dfrac{\partial u}{\partial \theta} & \dfrac{\partial z}{\partial \theta}\end{pmatrix}\vert=\vert det\begin{pmatrix}\sin\theta & \cos\theta\\ r\cos\theta & -r\sin\theta\end{pmatrix}\vert=r.$$

Plugging in, we obtain $$\vec{J}=i\dfrac{\delta(r-R)\delta(\theta-\frac{\pi}{2})}{r}\hat{\phi},$$ which is the desired result.