How come an anti-reflective coating makes glass *more* transparent?

Question

The book I'm reading about optics says that an anti-reflective film applied on glass* makes the glass more transparent, because the air→film and film→glass reflected waves (originated from a paraxial incoming wave) interfere destructively with each other, resulting on virtually no reflected light; therefore the "extra" light that would normally get reflected, gets transmitted instead (to honor the principle of conservation of energy, I suppose?).

However, this answer states that "Superposition is the principle that the amplitudes due to two waves incident on the same point in space at the same time can be naively added together, but the waves do not affect each other."

So, how does this fit into this picture? If the reflected waves actually continue happily travelling back, where does the extra transmitted light come from?

_{* the film is described as (1) having an intermediate index of refraction between those of air and glass, so that both the air-film and film-glass reflections are "hard", i.e., produce a 180º inversion in the phase of the incoming wave, and (2) having a depth of 1/4 of the wavelength of the wave in the film, so that the film-glass reflection travels half its wavelength back and meets the air-film reflection in the opposite phase, thus cancelling it.}

Answer 1

The thickness of the AR coating is chosen such that the reflections from the two interfaces cancel out (at the wavelength for which the AR coating was designed):

See Anti-reflective coating in Wikipedia.

As endolith points out in the comments, to explain how the transmission is enhanced, you have to draw a few more rays in the diagram. Here's another illustration, from the Wikipedia article for Fabry–Pérot interferometer, which shows a few higher-order reflections:

For the anti-reflective coating, you choose the thickness such that R1 and R2 cancel while T1 and T2 constructively interfere. Note that this is dependent on the wavelength, the angle of incidence, and the index of refraction of whatever is being coated. With other thicknesses, you can make a high-reflectivity coating, or a coating of whatever reflectivity you want.

Answer 2

I think that to really understand this, you have to abandon the idea that there are individual, separate electromagnetic waves. In reality, there's just one global electromagnetic field, $\bigl(\mathbf{E}(\mathbf{x}),\mathbf{B}(\mathbf{x})\bigr)$. It evolves in space and time in a manner determined by Maxwell's equations.

For certain configurations of the EM field - specifically, those with 2D translational symmetry - the evolution described by Maxwell's equations results in the shape of the field propagating in one direction. It's much like the way waves on the ocean (normally) propagate across its surface in one direction, without changing their shape. For this reason, we call these configurations of the EM field "plane waves." This is the sort of wave most people usually think of when they imagine a light wave. The key point, though, is that the idea of propagating plane waves really only arises in one particular case: when you have an isolated, 2D-symmetric EM field configuration. In general, the way the field evolves in time and space is more complicated than simple directional propagation, so in general, you can't always think of the field evolution as a wave.

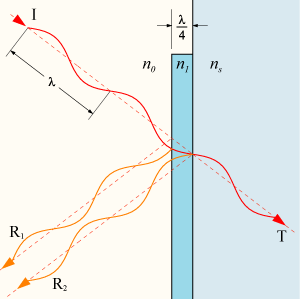
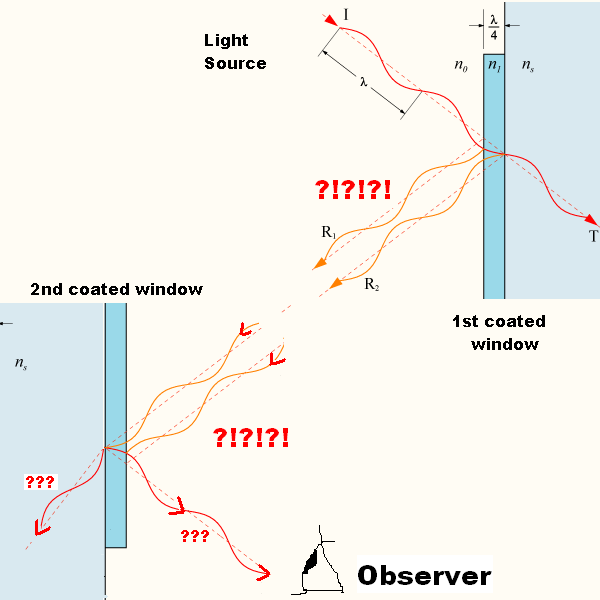
In the case of reflection specifically, even just reflection from a single surface, what this means is that the model of the incident wave reflecting off the boundary to produce a separate reflected wave is too simplistic. A more realistic description would be that the EM field has to satisfy specific conditions at the boundary between the surfaces, and that the only way to do this is for the field on the side of the incident wave to take a different value than it would have based on the incident wave alone. The difference between the actual field and the field that would be produced by the incident wave alone is called the reflected wave, because if you shut off the incident wave and wait a long time, you'll wind up with a simple plane wave propagating backwards away from the boundary.

The same holds true (i.e. the wave description is too simplistic) for double reflection with thin film interference; in fact, even more so, because it's a more complicated system. In this case, if you have a particular relationship between the distances and frequencies involved, you can arrange it so that the boundary conditions are satisfied by the incident wave alone, so there's no "extra" contribution to the field to be considered a reflected wave. Or in other words, if you shut off the incident wave and wait a long time, you will wind up with nothing propagating backwards, and thus we say that there is no reflected wave.

Answer 3

Perhaps it will help to recall that energy is a nonlinear function of the electromagnetic field. The superposition principle applies to the electromagnetic field, not the energy or power. So if two waves are superimposed out of phase, 1 - 1 = 0, we can say they are both happily traveling "independent" of each other (from the point of view of the EM field), but from the point of view of the energy they contain they are not independent.

Answer 4

The wave reflected from the air-film interface continues happily traveling back, as you say, but so does the wave reflected from the film-glass interface. Since they are the same frequency but in antiphase, they interfere destructively as long as they keep going. Since the superposition principle states that they do not affect each other in any way, they keep on going as long as they like.

However, there is no energy transported backwards in the reflected waves, because the energy is proportional to the square of the total electric field. It is the energy that is conserved, not the electric field, so all the energy (if not absorbed) is transmitted.

Answer 5

In the WP-link we can obtain a substantial explanation.
Read about the Fresnel coefficients WP-here
Play with multilayers at «thinfilm» (it uses the WP-transfer-matrix method).
The transmission is increased due to the forward and backward reflections in the coated film (medium 2) that are forward propagated to the medium 3 as seen in this image from pag 13.7 of B.O Sernelius lectures

What happens to the reflected rays in the layer 1 ? They simply cancel (vanish,do not exist) because they are in phase opposition in relation to the incident wave and almost all of the energy in the layer 2 will be forward propagated to 3.
Lets analyse the following experiment :

The image is a rework of a picture from the first WP-link, that can be misleading because it figures two reflected rays R1 and R2 that probably do not exist.
If those rays are really there, and we can not measure them because they are in phase opposition, then the observer in the bottom of the image will see the light restored to a nice level because the second coated window do the opposite action of the first window (beam splitter). The particle nature of light may favour this outcome.
If the observer can not see light then we must conclude that there is no field, energy, photons in the regions ?!?!?! of the experiment. The wave nature of light favour this outcome.

The experiment is very easy to perform.
Can someone post the outcome?

Answer 6

Let's talk about Where the extra transmitted energy comes from.

The energy quantum collapses into that position where it is found when measured.

Where the energy is found is a fundamentally random thing.

So, sometimes all energy is found in the area where waves cancel. Where cancellation approaches perfect cancellation, there probability of finding energy approaches zero.

So I'm saying that you will find photons with the normal photon energy in the cancellation area, and sometimes you find more photons in the cancellation area, than in the area of constructive interference.

Let's consider a very short pulse of light going through a window pane with anti-reflective coating. When a photon is detected behind the window, energy comes from where the reflected pulse is, and from where the transmitted pulse is.

When absence of photon is detected behind the window, energy goes from where the transferred pulse is, to where the reflected pulse is.