I am confused about Newton's 3rd Law. If a person jumps off the ground a force is applied both to the person and to the ground. However, as $F=ma$ acceleration experienced by the Earth is much less than that experienced by the person.
But: I press with gravitation force on the ground so it should press with the same force on me, so if my mass is less than earth mass my acceleration should be greater as well but I am not moving (flying)?
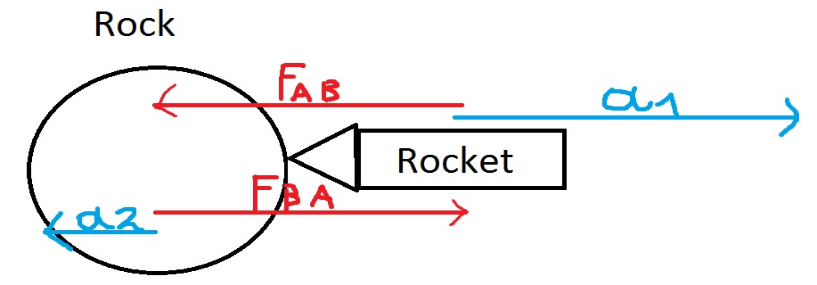
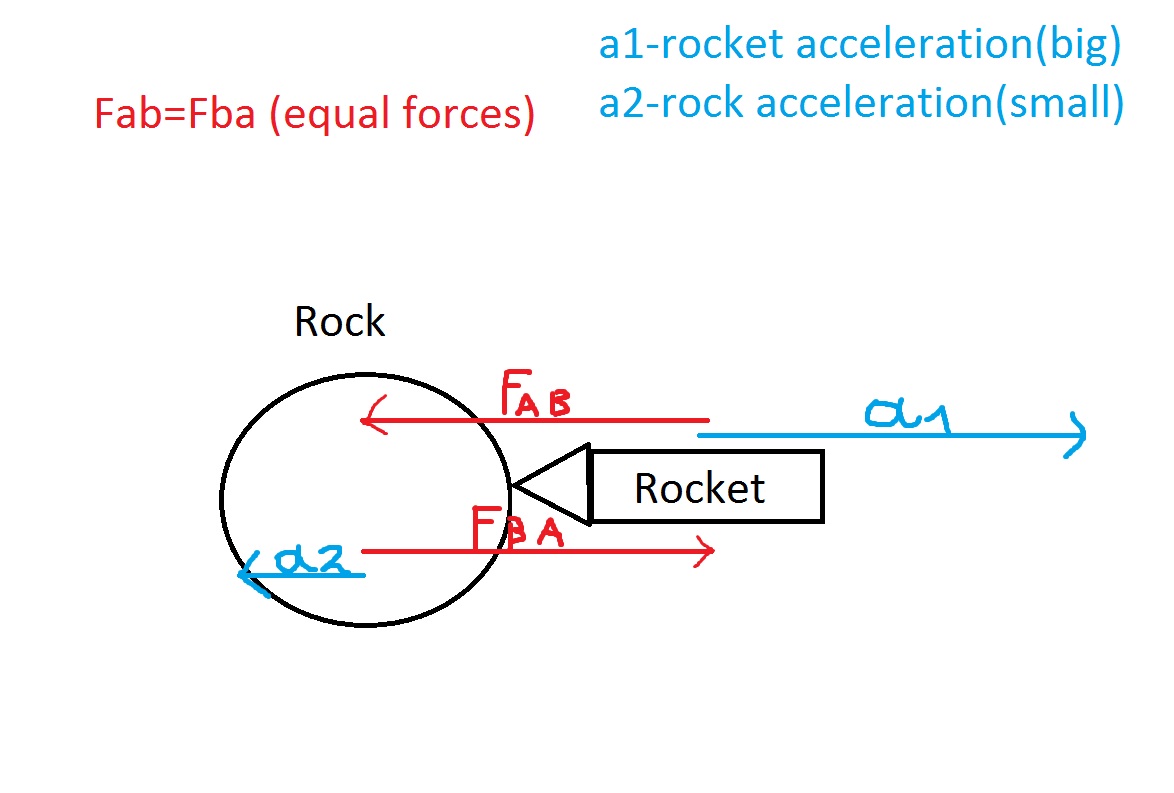
Second: If small rocket(with small mass) pressure against bigger rock with greater mass the rocket should have greater acceleration towards direction opposite to its flying path so how the rocket can actually move the rock(towards left at picture where rock acceleration is small) and not the opposite(rocket acceleration at picture towards right is bigger)?
Edited
If a rocket with mass $m_{rocket}$ pushes against a rock with mass $m_{rock}$ with force $F_{thrust}$ the rock will push back with equal force (Newton's 3rd Law). The rocket will experience an acceleration $a_{rocket}$ in the opposite direction of $F_{thrust}$ and the rock will experience $a_{rock}$ in the same direction as the thrust. However, in this example $m_{rocket} < m_{rock} \therefore a_{rocket} < a_{rock}$
Why doesn't the rocket move in the opposite direction of $F_{thrust}$ since the rock has a greater mass?
Not sure if it helps but added picture to second question
Edit:To put second question simple how a small(low mass) object can push big(great mass) object if according to newton 3rd law a big mass object causes greater acceleration on small mass object