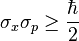You seem to be assuming that there is a measurement process that can be applied to the electron on a single, isolated hydrogen atom twice within somewhere under 10–19 seconds, and that because an electron in a hydrogen atom's ground state (or any $s$ state) is equally likely to be found along any direction from the nucleus, there's some chance that the electron will "jump" from one side of the nucleus to other.
But you are forgetting about the uncertainty principle, which governs the precision with which you may make a measurement. If you want to measure which side of the atom the electron sits on, you must have a precision better than the Bohr radius, $\Delta x < a_0 \approx \frac12\times10^{-10}\,\mathrm m$. After such a measurement, the electron must have a momentum uncertainty (in energy units) of
$$
\Delta p\, c \gtrsim \frac{\hbar c}{2\Delta x} = \mathrm{\frac{0.2\,GeV\cdot fm}{2\cdot \frac12\times10^{5}\,fm}} = \mathrm{2\times10^{-6}\,GeV} = 2\,\mathrm{keV}
$$
We can assume that the most likely value for $p$ is zero, since the electron began at rest. But a momentum of $pc = 0\pm 2\,\mathrm{keV}$, normally distributed, implies a typical squared momentum $(pc)^2 \approx (2\,\mathrm{keV})^2$, or a typical energy
\begin{align}
E
= \frac{p^2}{2m} \frac{c^2}{c^2}\approx \mathrm{\frac{(2\,keV)^2}{2\cdot 500\,keV}} \approx 4\,\mathrm{eV}
\end{align}
Remember that the hydrogen ground state has energy $-13.6\,\mathrm{eV}$, and that the first excited state has energy $-13.6\,\mathrm{eV}/2^2 = -3.4\,\mathrm{eV}$, neary 10 eV away. A position measurement with enough precision to distinguish "this side" from "that side" of a hydrogen atom involves nearly enough energy to promote the electron into a different state.
However it's also not really enough precision to say that you've measured faster-than-light travel for the electron: since the electron's $s$-wave wavefunction is roughly a three-dimensional Gaussian wavepacket whose width is the Bohr radius, you're mostly likely to find successive measurements of electron position separated by $1\sigma$. If you wanted to typically find electron positions separated by $3\sigma$ ("$3\sigma$ is a measurement, $4\sigma$ is a discovery," as one of my mentors likes to say, but $3^2=10$ is a nice round number), you'd have to improve your precision by a factor of three; this takes the energy associated with the measurement to 40 eV and means that your first position measurement will definitely ionize the atom, and your assumption that both measurements take place in the atom's ground state is broken.
Or alternatively, you could prepare your atom in an excited state (with radius $na_0$ for principal quantum number $n$) and keep the precision of your measurement the same. However, in that case also your first measurement would have enough energy to change the atom's state, or ionize it completely.
Then you have the question of how you'll measure the electron's position twice within 10–19 seconds—that's fast! Suppose you bathe the atom in an oscillating electric field with frequency $\nu=10^{19}\,\mathrm{Hz}$. In the quantum-mechanical picture, this field is made of photons, each with energy $E = h\nu \approx 40\,\mathrm{keV}$! It is vanishingly improbable that the atom would remain in its ground state after interacting with such a field. Your experiment would never work.
This second argument is in the spirit of Bohr's arguments during his debates with Einstein about the proper interpretation of quantum mechanics. But to my mind the first argument, based on the observation that bound-state wavefunctions tend to have nearly the minimum uncertainties $\Delta x,\Delta p$ allowed by the Heisenberg principle, is more interesting.
In some sense, the different states of the hydrogen atom have the size that they do because of the uncertainty principle.
Notice that relativity doesn't enter that argument explicitly, apart from the definition of energy $(E_\text{rest}+E_\text{kinetic})^2 = pc^2 + (mc^2)^2$, which I used in the more familiar low-momentum approximation $E_\text{kinetic} \approx p^2/2m$. The universe conspires in many ways to preserve its fundamental laws.