12
$\begingroup$

From the non-trivial nature of the QCD vacuum, the Lagrangian is augmented with a term like

\begin{equation} \theta \frac{g^2}{32 \pi^2} G_{\mu \nu}^a \tilde{G}^{a, \mu \nu} \end{equation}

where $ \tilde{G}^{a,\mu \nu} = \frac{1}{2} \epsilon^{\mu \nu \rho \sigma} G^a_{ \rho \sigma} $ is the dual field strength tensor. This term is said to violate CP, giving rise to the strong CP problem.

I understand the CP violation comes from the epsilon tensor in the dual field strength but I am looking for a simple straightforward demonstration of the CP violating nature of a term like $G \tilde{G}$.

Improve this question
$\endgroup$
4
  • 3
    $\begingroup$ I have answered this question here: physics.stackexchange.com/a/111178/6178 $\endgroup$
    – Frederic Brünner
    Commented May 26, 2014 at 9:08
  • 1
    $\begingroup$ possible duplicate of Yang-Mills CP violation $\endgroup$
    – Robin Ekman
    Commented May 26, 2014 at 9:27
  • 1
    $\begingroup$ Ok thanks. Is there another way to answer without using electric and magnetic fields, nor differential forms ? $\endgroup$
    – user42865
    Commented May 26, 2014 at 9:30
  • 9
    $\begingroup$ Well the simplest answer then is what you wrote, namely that it's because the Levi-Civita is a pseudo-tensor, so this term changes sign under parity. $\endgroup$
    – Robin Ekman
    Commented May 26, 2014 at 9:32

1 Answer 1

Reset to default
2
$\begingroup$

Old post, but I just heard of the following analogy recently so I thought I'd share. In the comments and elsewhere on this site, people have given a good answer about the mathematical answer to why the $\theta$ term breaks $CP$: the $\epsilon$-tensor is not invariant under $CP$, so the Lagrangian you wrote is not. But perhaps what you're looking for is something like this.

Physically, the $\theta$ angle (after appropriate gauge fixing if there are e.g. massless quarks) is determined by the electric dipole moment of the neutron. Classically, we can picture this the same way we picture the electric dipole moment of a water molecule. A neutron is (roughly) a bound state of an up and two down quarks. Imagine the classical picture of this scenario, where all three quarks are in the $xy$-plane. By symmetry, the "bond lengths" of the two down quarks to the up quark will be the same. Choose a coordinate system where the up quark is at the origin, and the down quarks are reflection-symmetric about the $y$-axis. In other words, imagine the following scenario, but with $O$ being the down quarks, and $H$ being the up quark:

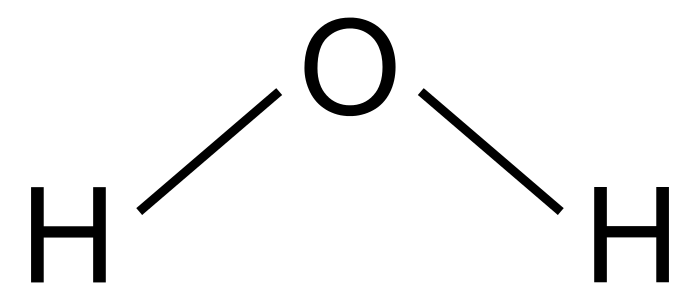
water but it's a neutron

(Sorry I don't have time to just draw the diagram directly). Call the angle subtended by the $x$-axis and one of the down quarks $\theta/2$. This is the classical analog of the $\theta$ angle.

This picture immediately reveals three things. First, $CP$ is unbroken if and only if $\theta=0$, i.e., if the quarks are in a straight line on the $x$-axis. That's because if $\theta \neq 0$, then a charge conjugation followed by an $y$-axis parity transformation will flip the sign of the dipole vector. That's true of the quantum case.

Second, $\theta =\pi$ will maximally break $CP$ because that's when the two down quarks are "on top of each other". That's also true of the quantum case.

Finally, we can see that the $\theta$ angle is a property of a bound state of QCD, and so it must be non-perturbative in nature. Indeed, the theta angle receives no loop corrections because of its topological character.

Of course, this is just a classical analogy, and not a proof in the quantum case. But you can show directly in QFT that $\theta$ is indeed related to the neutron dipole moment, so this classical picture isn't 100% wrong either.

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.