1
$\begingroup$

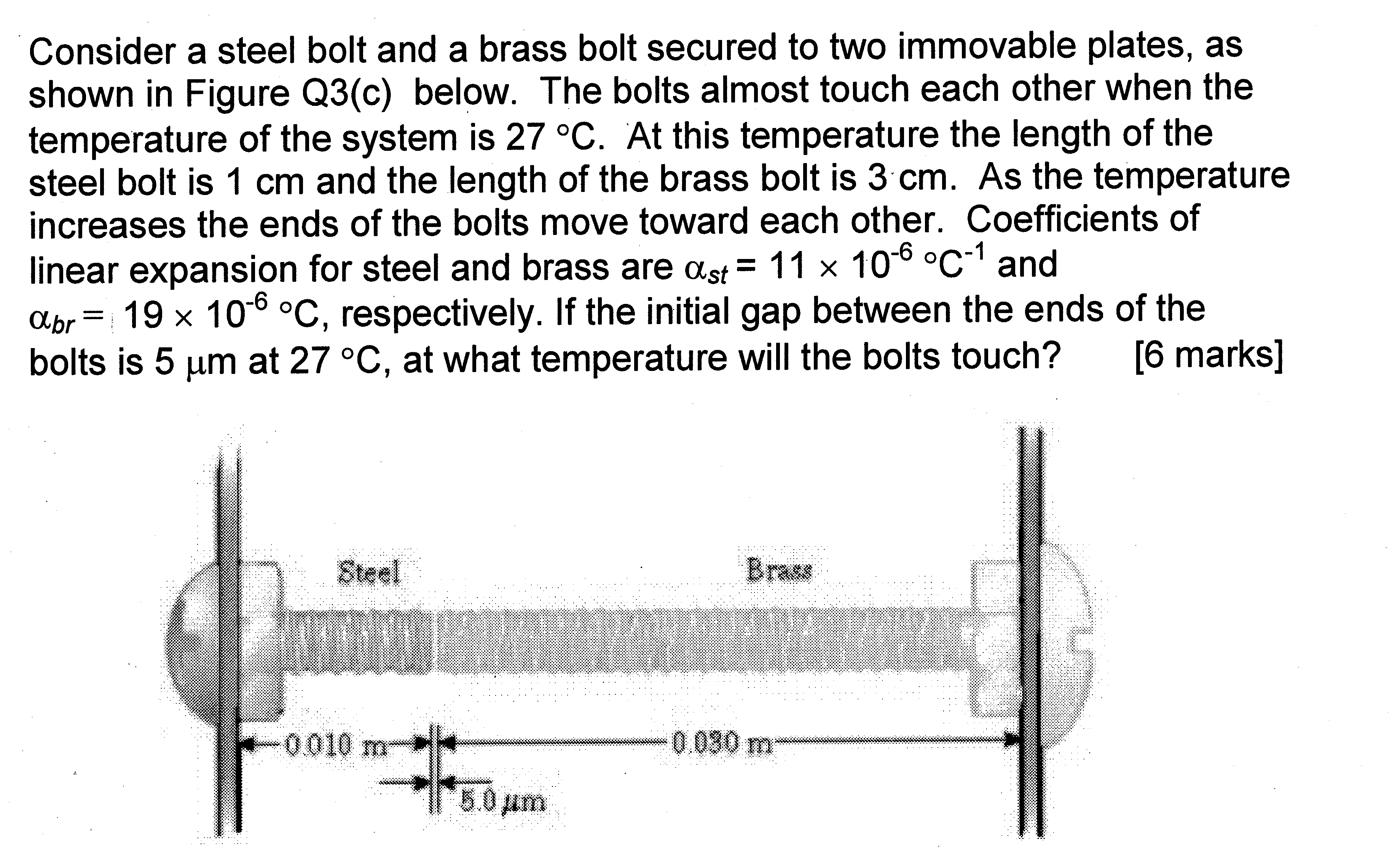
Bolts

I have this question asking me about the thermal expansions of two bolts, a steel one, and a brass one. I have the equation:

$\ (\delta L/L)/T = \alpha $

I don't understand how I can use this equation to manipulate the temperature for the two bolts simultaneously, as opposed to the temperature required to expand each individual bolt by 5 $\mu$m. Is this even the correct equation? Or is there another that I should be using.

What I have done is rearranged for T:

$\ (\delta L/L)/\alpha = T$

Then, using the individual values for $\delta$L = 5$\mu$m L= 0.01 m and the $\alpha$ for steel to get $T=45.5^\circ C$ and similarly, for Brass, to get $T=8.77^\circ C$. This obviously cannot be correct, considering, using this method, the $T_0=27^\circ C$ has not been taken into consideration.

Any help would be good. Thanks.

Improve this question
$\endgroup$
8
  • $\begingroup$ The total length for the expansion is $5 \mu m$, so you will want to add the expansion of the steel with the expansion of the brass. Also I believe you have $\Delta T$, not $T$. I also saw that the units for $\alpha _{br}$ is incorrectly stated as $°C$ and not $°C^{-1}$. $\endgroup$
    – LDC3
    Commented May 25, 2014 at 17:18
  • $\begingroup$ The incorrect units are within the image made by the creator of the question. By adding the expansions, I assume you mean add the two coefficients of linear expansion together? $\endgroup$
    – Weasel
    Commented May 25, 2014 at 17:25
  • $\begingroup$ That will not work. What I mean is $\delta L_{st}+\delta L_{br}=5.0μm$. $\endgroup$
    – LDC3
    Commented May 25, 2014 at 17:35
  • $\begingroup$ So are you implying: $((\delta L_st + \delta L_Br) / L)/ \alpha = \Delta T $ Clearly I must not understand where you are coming from, considering both $\alpha$ are different. $\endgroup$
    – Weasel
    Commented May 25, 2014 at 17:44
  • $\begingroup$ The equation is more complex; give me a moment and I'll post it. $\endgroup$
    – LDC3
    Commented May 25, 2014 at 17:51

0

Reset to default

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.