A question about a complex integration in Peskin's QFT textbook

Question

In page 27 (2.52), the integration is $$\int_{-\infty}^{\infty}dp \frac{p e^{ipr}}{\sqrt{p^2+m^2}}$$ He says that there are two branch cuts starting from $\pm im$

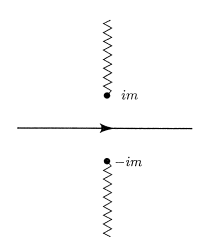
But I learn in complex analysis that $\sqrt{z^2+m^2}$ has only one branch cut from $-im$ to $im$, because point going around $im$ or $-im$ only will gets a minus, but point going around $\infty$ only will keep the sign. Therefore $\infty$ point is not the branch point and the branch cut is from $-im$ to $im$. So who is wrong?

Answer 1

Remember this all start from the fact that $\left(z^2+m^2\right)^{-\frac{1}{2}}=\left(z+im\right)^{-\frac{1}{2}}\left(z-im\right)^{-\frac{1}{2}}.$

So you have two branch points: one in $-im$ and one in $im.$

Let's now consider the branch cuts as on the image you posted. If you rotate upper branch of $\pi$, so is directed to the bottom, then the two brances will intersect. You have the following situation:

• From $im$ to $-im$: you have only one branch (the one starting from $im$ that has been rotated)
• From $-im$ to, let's say, "$0-i\infty$": you have two branches overlapping. In this situation, since the sum of the exponents is an integer number ($-\frac{1}{2}-\frac{1}{2}=-1$), you have no polydromy. Then you have no line of discontinuites here.

So, rotating the branch cuts in figure you obtain the well known branch cuts from $im$ to $-im.$ Then they are equivalent.