2
$\begingroup$

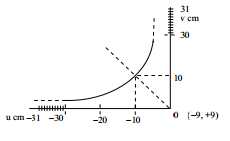
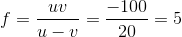enter image description here

$u=-10cm$ $v=10cm$

enter image description here

Using the formula the focal length is 5 cm. But how do I get the fractional error in focal length when neither $\Delta u$ nor $\Delta v$ are specified?

The options given are
A. (0.05±0.05) cm
B. (0.05±0.10) cm
C. (5.00±0.05) cm
D. (5.00±0.10) cm

Improve this question
$\endgroup$
3
  • $\begingroup$ What are the given options? $\endgroup$
    – fibonatic
    Commented May 2, 2014 at 8:22
  • $\begingroup$ You can assume an error of 1mm in each like they were measured by a "normal" scale. $\endgroup$
    – evil999man
    Commented May 2, 2014 at 9:59
  • $\begingroup$ @Awesome Yes I was going to do that, but do you know what the formula for error in "f" would be? $\endgroup$
    – Niharika
    Commented May 2, 2014 at 11:29

2 Answers 2

Reset to default
0
$\begingroup$

Error is $1 mm$ ,i.e. the least count as provided at the end of your axes where $1 cm $ is divided into 10 divisions.

$$f=\frac{uv}{u-v}$$

Fractional Error in $f$=fractional error in $uv$ + fractional error in $u-v$.

And fractional error in $uv$=fractional error in $u$+ fractional error in $v$.

Error in $u-v$= Error in $u$+error in $v$ // Note that it is not relative error

Can you continue from here?

Improve this answer
$\endgroup$
8
  • $\begingroup$ We can do it without the calculus right? I'd rather prefer not to use calculus right now. $\endgroup$
    – Niharika
    Commented May 2, 2014 at 13:01
  • $\begingroup$ @Niharika How have you been taught to find error? $\endgroup$
    – evil999man
    Commented May 2, 2014 at 13:02
  • $\begingroup$ Yes. First we have to make "f" the subject of the equation, you'll get the same equation I used above. From there, fractional error in "f"= fractional error in (uv) + fractional error in(u-v). Fractional error in (uv) is fractional error in u + fractional error in v. How do I simplify fractional error in (u-v) ? $\endgroup$
    – Niharika
    Commented May 2, 2014 at 13:07
  • $\begingroup$ @Niharika That result is derived using calculus. This works. Let me edit. $\endgroup$
    – evil999man
    Commented May 2, 2014 at 13:09
  • $\begingroup$ Yes. But just because end of the graph is calibrated to mm, doesn't mean that the least count is 1mm right? So that is just the most logical assumption right? $\endgroup$
    – Niharika
    Commented May 2, 2014 at 13:21
0
$\begingroup$

This is a bit of a sloppy problem, because the numbers given ("10cm") are not in engineering notation. Sans any other specifications, I would default to the uncertainty being 0.5cm , i.e. half the least significant digit provided. Trouble is, it could just as easily be a value rounded to the nearest ten-centimeter value, implying the uncertainty is 5cm. I do think Awesome's assumption of 1mm is highly optimistic.

If your teacher wrote this problem, ask him to be more careful about his notation. If it's from a book, just write down your assumptions about the uncertainty so you can justify your work.

Improve this answer
$\endgroup$
2
  • $\begingroup$ Well the question has a bit of a back story. This is the graph drawn by a student who conducted an experiment to caluculate the focal length using the "uv" method. Though we cannot be certain, It is very much possible that he used a 30 cm scale to record his readings so I GUESS taking the 1mm error can be justified. Also, can we take "half the least significant digit provided" as the error when we have no clue about the least count of the instrument or possible error? $\endgroup$
    – Niharika
    Commented May 2, 2014 at 13:05
  • $\begingroup$ @Niharika The markings are provided on axes telling the least count. between 30 and 31. $\endgroup$
    – evil999man
    Commented May 2, 2014 at 13:26

Not the answer you're looking for? Browse other questions tagged or ask your own question.