There has to be a few assumptions. Let's assume we are talking about a linear plane wave in relatively deep water. Because the the case where the bottom comes into play the upward hydrostatic force distorts the wave. Picking deep water or insuring the relative depth of d to L (d is average water depth and L is the wavelength of the wave) is $d/L > 0.5$. Compare the deep water case to the shallow water case and the particle motion in each condition (SWL is standing or still water level) is shown by the circles or ovals:
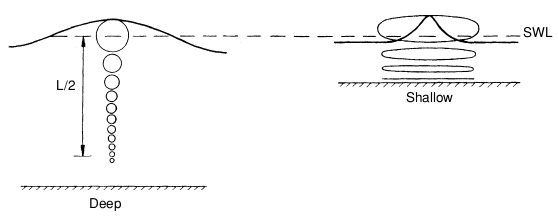
When a wave propagates from deep water offshore in to shallower water near-shore the wave length decreases, but at a slower rate than that at which the depth decreases. This shift to higher frequency also deforms the wave. The bottom is applying force on the wave which also lifts it up above the SWL until it becomes unstable and breaks.
Since in your comments you mention you're looking for momentum (not really mass), we have to talk about what that means in a wave. In fluid flow problems, some analyses are best carried out by energy considerations (e.g., head loss along a length of pipe) and some by momentum considerations (e.g., force exerted by a water jet hitting a wall). Similarly, for waves it is better to consider the flux of momentum for some problem analyses. For wave analyses, the flux of momentum is commonly referred to as the wave "radiation stress" which may be defined as "the excess flow of momentum due to the presence of waves" (Longuet-Higgins and Stewart, 1964).
The analysis of the momentum is based on the particle motion shown above and the computation is well illustrated on the wiki page for momentum flux including the boundary conditions. (Note that in this derivation gravity is defined as negative downwards). The end result for deep water waves is a momentum flux of
$\frac{dM}{dt}=-\frac{1}{4}\rho gA^2$
Where $\rho$ is the water density, g is gravity and A is the height of the wave (middle to crest).
As an aside about breaking waves they are very different conditions.
In the case of a breaking wave the common profile is shown below (not a sawtooth wave).
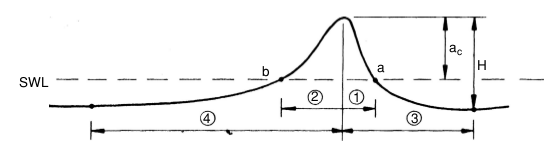
With some of the common terms defined as:
- Vertical asymmetry: $\frac{a_c}{H}$
- Slope asymmetry: 0.5(slope a + slope b)
- Horizontal asymmetry (1): distance 1/distance 2
- Horizontal asymmetry (2) distance 3/distance 4
The vertical asymmetry continuously increase as the wave shoals, reaching a maximum of between 0.62 and 0.74 at breaking. The slope and horizontal asymmetries also continuously increased as the wave shoaled; but, as opposed to vertical asymmetries steeper bottom slopes caused greater slope and horizontal asymmetries. And as the wave height increases the crest particle velocity will eventually equal the wave's phase velocity (or celerity $C=\frac{L}{T}$) and the wave will break.
Breaking waves fit into 4 basic category conditions/shapes: