Generally speaking, the lowest mass particles are stable against decay because they can't spontaneously decay into more massive particles (energy wouldn't be conserved in the rest frame). So particles not made up of up and down quarks (least massive of the quarks; see figure) will quickly decay into particles made up of only up and down quarks.
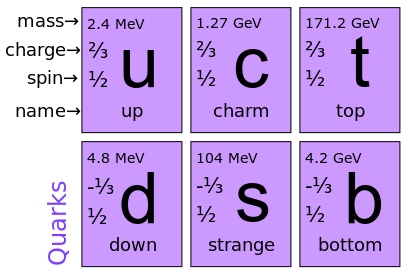
This leaves the neutron (udd) and proton (uud) as the only stable particles of which the neutron is more massive and can hence decay into a proton (by conversion of a more massive down quark to an up quark through the weak interaction) - this is called Beta decay and is an important and well established radioactive process. There is nothing that the proton can spontaneously decay into while conserving energy in the Standard Model.
Beyond the Standard Model, most Grand Unified Theories that try to unify the three non-gravitational forces require proton decay since quarks and leptons can now change into each other. This means that the proton will have a finite (albeit very large) lifetime. Some GUTs, such as SU(5), have already been ruled out because the proton lifetimes they predict are smaller than the observed lifetime.

