Questions about spacetime curvature and gravity

Question

These are some further important queries regarding the question here Why would spacetime curvature cause gravity?

Q1. Explain the statement “Everything in spacetime moves at the speed of light”. Is this statement even true or false? My naïve understanding is as follows: If an object is stationary in space (relative to an observer?) then it will be moving in time, so, it will, say, move from (x=0,t=0) to (x=0,t=10). The velocity is the space interval / time interval, which from above coordinates is still zero. So it is moving through spacetime, but at zero velocity. So velocity will just determine the angle of its path to the time axis. If this angle is zero, ie it is moving parallel to the time axis, then velocity = zero, and it can really move in time from one time point to the other at zero velocity. Where am I wrong, and what is the real explanation?

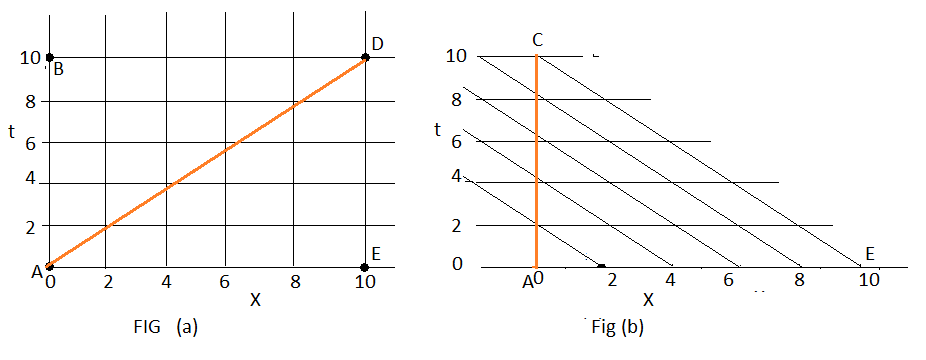
Q2. Suppose there are two objects (see fig), an apple A above the earth at x=0, and the earth E at x=10 as shown in the fig. If there is no earth and just the apple in open space, then the spacetime is not curved due to the gravity of the earth, and the apple stays at x=0 but moves in time from A to B (fig a). Now the earth is at x=10 and presumably the spacetime curves and the axes tilt as in fig b. Then the apple moves from A to C, just following the geodesic. But if we assume this, then the apple has not moved at all, because, due to the tilt of the axis, point C is also at x=0. So fig b cannot be the correct situation, otherwise the apple will arrive at point C which is still on the x=0 axis. So I assume that in fact the apple has not moved from A to C in fig b, but has moved from A to D in fig. a, where D is really at x=10. But if I assume this, then it is not spacetime itself that has curved. The spacetime is still straight, but the apple has moved from (x=0,t=0) to (x=10,t=10). Again, where am I wrong, and what is the correct explanation? How exactly has the spacetime curved or tilted for the apple at A due to the presence of the Earth at E, and how does the apple move to the earth by following the geodesic in free fall? (assume only one spatial dimention)

Q3: We say that gravity is not just a “force of attraction” between two pieces of mass, and it does not “pull” the two pieces of mass towards each other. Instead, acceleration is manifested because the two pieces of mass are simply following their now curved geodesics. But it can be shown that there really is an attractive force due to gravity. E.g. Take the apple in the earth’s gravity and suspend it on a spring balance. The spring will extend. Yes, you can say that since it is suspended motionless, so it is no longer in free fall, and since the spring balance exerts an external force upwards on the apple, so it no longer follows its free fall geodesic. But it is not only the spring balance which is exerting an upward force on the apple. The apple is also exerting a downward force on the spring, which causes the spring to extend. Surely it is too naïve to say that the downward force which the earth exerts on the apple and in turn the apple exerts on the spring suddenly vanishes if the apple is released into free fall.

If two masses were remotely attracting each other with a force, why would they cease to attract each other with a force when in free fall? It is logically a much more satisfying explanation that the gravitational attraction force which was there at rest remains in free fall too and it is this gravitational attraction force which causes the freefall acceleration by F=ma, just like any force on any object would cause an acceleration. Also, it is the net force on the object which causes the acceleration. When the object was suspended, the net force was zero, so acceleration was zero. When in free fall, the net force IS THE GRAVITATIONAL ATTRACTION FORCE, so acceleration is accordingly. This seems totally opposite to the statement that there is no gravitational force in free fall.

The reason why the spring of the spring balance does not extend when in free fall is that there IS a gravitational force downwards, but there is no force exerted by the spring upwards, so the net force on the object is downwards due to gravity, which causes the acceleration F=ma. Where am I wrong? What is the correct explanation?

Answer 1

Re (1): the relativistic extension of velocity is four velocity. Pre relativity we separate the spatial and time coordinates, then define velocity as $dx/dt$ etc. In relativity this no longer makes sense because $x$ and $t$ are both spacetime coordinates and indeed the Lorentz transformations will mix them up. So we define the four velocity $U$ as the vector:

$$ U = \left(\frac{cdt}{d\tau}, \frac{dx}{d\tau}, \frac{dy}{d\tau}, \frac{dz}{d\tau}\right) $$

where $\tau$ is the proper time. In your example of a stationary object $dx$, $dy$ and $dz$ are all zero, and $dt/d\tau = 1$ (because in the rest frame of an object the coordinate time is equal to the proper time). So the four velocity is:

$$ U = \left(c, 0, 0, 0\right) $$

and hence the claim that a stationary object has a four velocity equal to $c$. Lorentz transformations to other frames will change the components of the four vector, but its magnitude will always be $c$.

Re (2): your drawing isn't a good description of the spacetime near a spherically symmetric object like the Earth. In these circumstances the curvature is given by the Schwarzschild metric. Calculating the trajectory of your apple in this metric is far from trivial. As it happens this issue is addressed on the recent question Naive visualization of space-time curvature. Sadly, the answer to this question is that there is no reliable naive visualisation of spacetime curvature. The only way to calculate the trajectory is to bite the bullet and use the geodesic equation.

Re (3): the stationary apple attached to the spring is accelerating, and therefore a force is being applied to it. The procedure for calculating this acceleration is described in the question What is the weight equation through general relativity?, as is the force required to be exerted by the spring to keep the apple accelerating.

You object that the apple is also exerting a force on the spring. Well yes, this is just Newton's third law. The spring in turn exerts a force on whatever is supporting the upper end of the spring, and so on.

Answer 2

Since no one has answered the second question, and since I discovered the answer subsequently, so I will post it. I only hope that it is correct. The spacetime bends backwards, so that if the the apple takes the shortest path, ie vertical, it reaches the point C where x=10 (in fig b) due to the backward tilt of the axis. Actually, these lines are curves to allow for parabolic nature of acceleration. I have drawn them as straight lines, just to show approximately which way they will bend. Remember, the apple started out at A, where x=0, and the earth was at x=10. Compare with the fig in the question which did not make sense due to the tilt of the axes in the wrong direction.

The vertical line followed by the apple in fig b shows that the apple thinks that it is at rest and the earth is accelerating towards it.

Answer 3

How exactly has the spacetime curved or tilted for the apple at A due to the presence of the Earth at E, and how does the apple move to the earth by following the geodesic in free fall? (assume only one spatial dimention)

Spacetime is not "tilted", like you show it, because the two dimension must be orthogonal everywhere. This is shown here:

http://www.youtube.com/watch?v=DdC0QN6f3G4