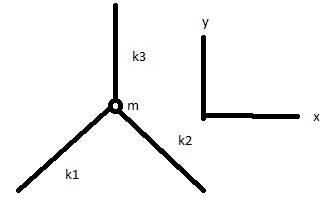
The simplest setup is for small displacements. Suppose the spring rest lengths are $L_1,L_2,L_3$, the mass has mass $m$, the springs have constants $k_1,k_2,k_3$, the angle is 120 degrees between attachments, and the attachment points are set up so that at rest, the springs are all unstretched.
The potential becomes
$$U(\mathbf{r})=\sum_{j=1}^3\frac{k_j}{2}(|\mathbf{r}-\mathbf{u}_j|-L_j)^2$$
where
$$\mathbf{u}_j=\{L_j\cos(2\pi j/3),L_j\sin(2\pi j/3)\}.$$
It's easy to verify that
$$\nabla U(\mathbf{0})=\mathbf{0}$$
which means that the system is at equilibrium when the mass sits at the origin.
Defining
$$H=\nabla\nabla U(\mathbf{0})=\left(
\begin{array}{cc}
\frac{1}{4} \left(k_1+k_2+4 k_3\right) & \frac{1}{4} \sqrt{3}
\left(k_2-k_1\right) \\
\frac{1}{4} \sqrt{3} \left(k_2-k_1\right) & \frac{3}{4}
\left(k_1+k_2\right) \\
\end{array}
\right)$$
we obtain eigenvalues
$$\lambda_\pm=\frac{1}{2} \left(k_1+k_2+k_3\pm\sqrt{k_1^2-k_2 k_1-k_3
k_1+k_2^2+k_3^2-k_2 k_3}\right)$$
and the ordinary vibrating frequencies become
$$\omega_\pm=\frac{1}{2\pi}\sqrt{\frac{\lambda_\pm}{2m}}.$$
Notice that the lengths are irrelevant, and that in the case $k_1=k_2=k_3$ the frequencies become identical, thus becoming like a 2D spherical oscillator.
When you add more masses to the system, things get interesting.
What manner of driving force are you planning on applying?