2
$\begingroup$

A galvanometer can be converted into an ammeter by connecting a low resistance (called shunt resistance) in parallel to the galvanometer.

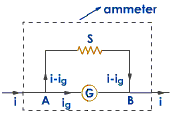
enter image description here

Firstly, why do we need to connect the resistance?

If a resistance is connected in parallel, then some of the current will flow through resistance. So how can the current in the circuit be determined? Won't it be inaccurate, as some of the current flows through resistance?

Improve this question
$\endgroup$
0

3 Answers 3

Reset to default
3
$\begingroup$

First things first, the resistance is connected in the circuit parallel to the galvanometer to minimise the total resistance of the ammeter. Whenever 2 resistances are connected in parallel, the equivalent resistance is always less than the lowest resistance.

You know that:
$\frac{1}{R_{eq}} = \frac{1}{R_1} + \frac{1}{R_2}$

$$\implies R_{eq} = \frac{R_1R_2}{R_1 + R_2}$$

eg: Try plugging in values. If $R_1 = 5\Omega$ and $R_2 = 0.2\Omega$:
$$R = \frac{5\times0.2}{5 + 0.2} = \frac{1}{5.2} = 0.19$$ which is less than 0.2, thus reducing the equivalent resistance.

This is done so that the ammeter has the least resistance possible and it doesn't interfere with the potential drop across the circuit.

Now, the ammeter is callibrated in such a way that it gives the correct reading, even if the current through G isn't what it says.
It's like calculating your weight while holding a 20kg bag. You can still get the correct weight by shifting the scale of your weighing machine down by 20kg! ;)

Improve this answer
$\endgroup$
3
  • 1
    $\begingroup$ But why we need to connect resistance?? $\endgroup$
    – vaibhav
    Commented Feb 22, 2014 at 8:52
  • 1
    $\begingroup$ @vaibhav As I said, connecting a low-resistance brings down the total resistance of the ammeter, so that it doesn't interfere with the amount of current in the circuit. Ideally, an ammeter should have 0 resistance, but obviously, that isn't possible, so we try to bring the resistance as low as possible. $\endgroup$
    – mikhailcazi
    Commented Feb 22, 2014 at 9:02
  • 1
    $\begingroup$ @mikhailcazi Since the majority of current will flow through the resistance then how will the ammeter give full scale reading $\endgroup$
    – Hydrous Caperilla
    Commented Apr 16, 2018 at 2:04
1
$\begingroup$

You yourself have answered your question.

...into an ammeter by connecting a low resistance (called shunt resistance) in ...

The galvanometer has it's own resistance $R$ (of course it will unless it is ideal). If entire current flows through it, it will have a lot of contribution to potential difference ($i*R$) across it and affect circuit working.

To decrease the potential difference across it a low resistance ($r << R$) is connected across it so that the potential difference becomes much less than it was previously ( $ r*i_s << R*i$) and has a less influence of circuit working.

The galvanometer is already set to measure the correct current despite of the shunt.

Improve this answer
$\endgroup$
1
$\begingroup$

galvanometer can be converted to an ammeter by using a shunt resistor in parallel with it. procedure;connect the galvanometer in parallel with a resistor with low resistance namely shunt resistor.as the current flows much of it will flow through the shunt resistor and little flows through the galvanometer.basing on ohms law, current is inversely proportional to resistance,implying when resistance is low much current flows through the resistor.by doing so you will have got an ammeter.

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.