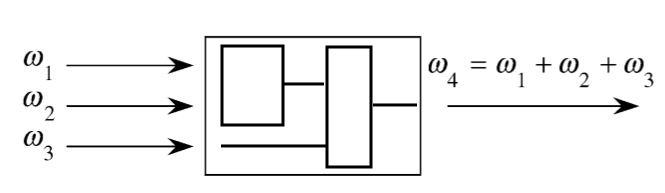
It's pretty straight-forward to visualise second-order non-linear optical mixing processes in which two waves of frequencies $\omega_{1}$ and $\omega_{2}$ mix up to generate new waves of different frequencies.
For example the sum-frequency generation:
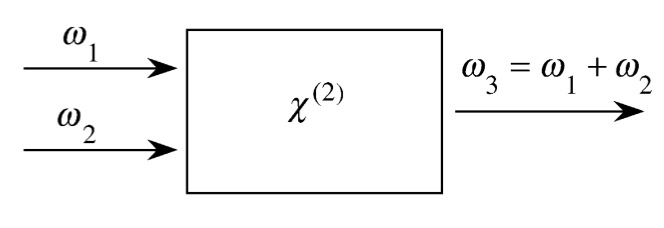
Or the difference-frequency generation:
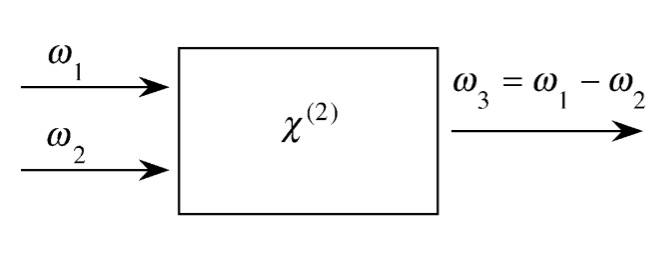
But my question is, what new waves could the two ($\omega_{1}$ and $\omega_{2}$) waves generate by third-order non-linear optical mixing processes?
Would it be possible to have a two-wave input and a two-wave output? For example, $\omega_{1}$ and $\omega_{2}$ input; $\omega_{1}+\omega_{2}$ and $\omega_{1}+2\omega_{2}$ output?
The only examples of third order processes that I found on my references involve an input of three waves, as in: